
What are conservative forces?
Answer
494.7k+ views
HintConservative force has a property that work done in moving a particle between two points is independent of the path taken.
Complete step-by-step solution:The work done by such forces
Does not depend upon path.
Depends only and only initial and final position.
For example:- If A and B are the two points and a conservative force acts on point A and B. Then we assume the three ways to go at point B . 1,2 and 3 are the three ways.
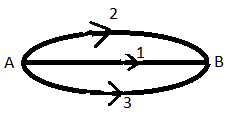
If the force is conservative, then work done by this force by first path, by second path and third path are equal.
Let
Then,
Examples of conservative forces are gravitational force , spring force, and electrostatic force.
First we talk about gravitational force, let us assume
For path 1:-
Angle between force
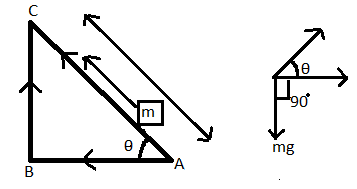
Than
For path 2
First we go from point A to point B and then from point B to point C.
When we go from A to B then the angle between force and displacement is
Than
When we go from point B to C then the angle between force and displacement is
So the work done in the first path is equal to work done in the second path. So it is independent of path.
Note:
Students think that whose path has long distance then work done is maximum for that path, but for conservative force work done does not depend on path distance.
Complete step-by-step solution:The work done by such forces
Does not depend upon path.
Depends only and only initial and final position.
For example:- If A and B are the two points and a conservative force acts on point A and B. Then we assume the three ways to go at point B . 1,2 and 3 are the three ways.

If the force is conservative, then work done by this force by first path, by second path and third path are equal.
Let
Then,
Examples of conservative forces are gravitational force , spring force, and electrostatic force.
First we talk about gravitational force, let us assume
For path 1:-
Angle between force

Than
For path 2
First we go from point A to point B and then from point B to point C.
When we go from A to B then the angle between force and displacement is
Than
When we go from point B to C then the angle between force and displacement is
So the work done in the first path is equal to work done in the second path. So it is independent of path.
Note:
Students think that whose path has long distance then work done is maximum for that path, but for conservative force work done does not depend on path distance.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

In forest ecosystem pyramid of number is a Upright class 12 biology CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE

Which of the following is a renewable resource A Natural class 12 physics CBSE




