Question

What is a normal line to a curve?

Answer 1

Hint: For solving this type of question you should know about the normal line of a curve. Generally, a normal line of a curve is the line which is perpendicular on the tangent line at the point of tangency. The slopes of the perpendicular lines are negative reciprocals of one another and the slope of the normal line to the graph of $f\left( x \right)$ is always defined as $\dfrac{-1}{f'\left( x \right)}$.

Complete step by step answer:
As it is asked in the question to explain the normal line to a curve, so for this we have to understand what a curve is and what are the components for the curve.

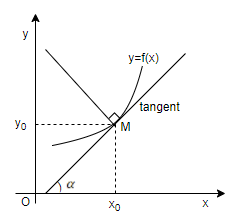
By the above diagram, we can see that the tangent line is defined as tangent and the tangent point is $\left( {{x}_{0}},{{y}_{0}} \right)$ which is denoted as M. And a straight line perpendicular to the tangent and passing through the point of tangency $\left( {{x}_{0}},{{y}_{0}} \right)$ is called normal line to the graph of the function $y=f\left( x \right)$.
So, we can find that the geometry is showing that the product of the slopes of perpendicular lines is equal to -1.
Therefore, the equation of then tangent at the point $\left( {{x}_{0}},{{y}_{0}} \right)$ will be,
$y-{{y}_{0}}=f'\left( {{x}_{0}} \right)\left( x-{{x}_{0}} \right)$
We can write this equation of the normal in the form,
$y-{{y}_{0}}=\dfrac{-1}{f'\left( {{x}_{0}} \right)}\left( x-{{x}_{0}} \right)$
Because the slope of any line perpendicular to a line with slope m is the negative reciprocal $\dfrac{-1}{m}$. So,
$m=f'\left( {{x}_{0}} \right)$
And slope of the line which is perpendicular to a line is $\dfrac{-1}{m}$, which is $\dfrac{-1}{f'\left( {{x}_{0}} \right)}$.

Note: If you calculate the slope of any normal line then you should be careful because first you have to find the tangent of the line and then by the help of tangent line you can find the tangent point where the perpendicular line or the normal line will contact this at an angle of ${{90}^{\circ }}$ which is known as perpendicular or normal line. And after finding the slope you can directly get the normal line by putting this in the line equation.