Answer
Verified411.6k+ views
Hint: In this problem, we have to find the Z score for \[95\%\]. We can see that this statistical problem is based on the z confidence interval for the mean. We should know that to obtain the value for the given percentage, we have to refer to the area under the Normal distribution table. We can first draw the area and split into two parts to find the z score for the given percentage.
Complete step by step solution:
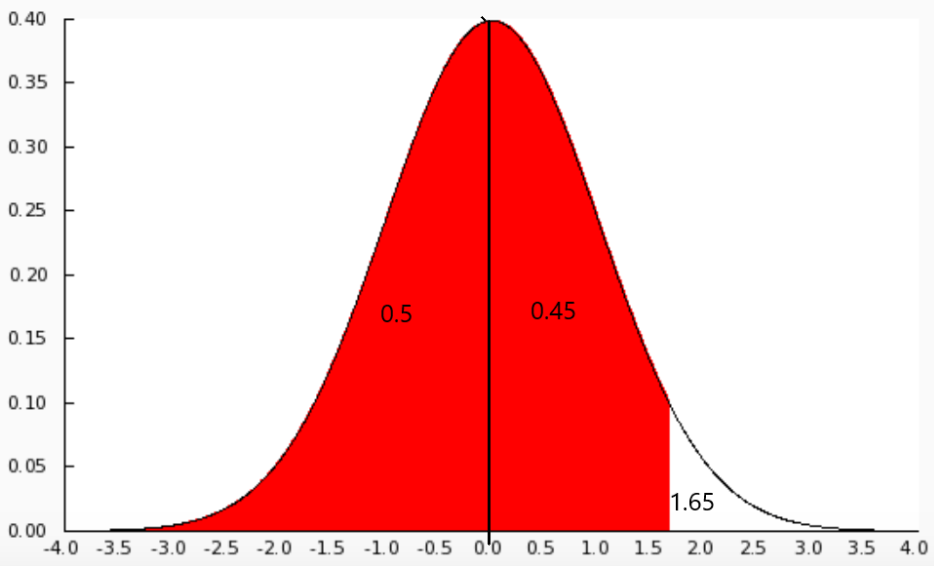
We have to find the Z score for \[95\%\].
We know that to obtain the value for the given percentage, we have to refer to the area under the Normal distribution table.
We know that the area under the normal curve represents total probability, which is equal to 1 at two extreme values \[z=\infty \] and \[z=-\infty \].
We can see that the area of one half of the area is 0.5 and the value of z exactly at the middle is 0.
We know that on one side, we have 0.5, so the remaining half will be,
\[\Rightarrow 1-0.5=0.45\]
Now we have to find the z value for 0.45, we can move along the area in the table and locate the nearest value. It will be 0.4505 in the table.
We will see a value in the z column if we move left in the table, which will be 1.6.
We can then move vertically up and reach the top most row in the table, we will find 0.05.
We can now add these values we get,
\[\Rightarrow 1.6+0.05=1.65\]
Therefore, the Z score of \[95\%\] is 1.65.
Note: Students make mistakes while drawing the area under the normal curve and plotting the correct points over there. We should always remember that We know that the area under the normal curve represents total probability, which is equal to 1 at two extreme values \[z=\infty \] and \[z=-\infty \]. We can see that the area of one half of the area is 0.5 and the value of z exactly at the middle is 0.
Complete step by step solution:
We have to find the Z score for \[95\%\].
We know that to obtain the value for the given percentage, we have to refer to the area under the Normal distribution table.
We know that the area under the normal curve represents total probability, which is equal to 1 at two extreme values \[z=\infty \] and \[z=-\infty \].
We can see that the area of one half of the area is 0.5 and the value of z exactly at the middle is 0.
We know that on one side, we have 0.5, so the remaining half will be,
\[\Rightarrow 1-0.5=0.45\]
Now we have to find the z value for 0.45, we can move along the area in the table and locate the nearest value. It will be 0.4505 in the table.
We will see a value in the z column if we move left in the table, which will be 1.6.
We can then move vertically up and reach the top most row in the table, we will find 0.05.
We can now add these values we get,
\[\Rightarrow 1.6+0.05=1.65\]
Therefore, the Z score of \[95\%\] is 1.65.
Note: Students make mistakes while drawing the area under the normal curve and plotting the correct points over there. We should always remember that We know that the area under the normal curve represents total probability, which is equal to 1 at two extreme values \[z=\infty \] and \[z=-\infty \]. We can see that the area of one half of the area is 0.5 and the value of z exactly at the middle is 0.