
Which is the smallest wavelength that will be observed in the spectra of
Answer
502.2k+ views
Hint: The smallest wavelength in the spectra of atoms corresponds to the Lyman series of the spectral series. The atomic number of
Formula used:
where
Complete step-by-step answer:
When electric current is passed through an atomic gas, the gas emits radiations of certain specific wavelengths. A spectrum is formed and this is called the Line emission spectrum. In this spectrum, groups of spectral lines are observed. These groups are named as spectral series. According to increasing wavelength, these series are named as Lyman series, Blamer series, Paschen series, Brackett series and Pfund series.
When electric current is passed through
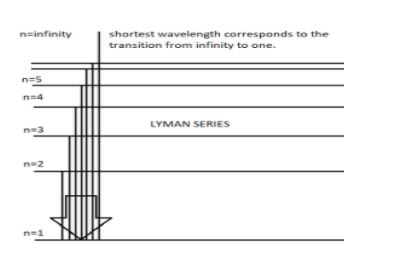
Rydberg equation is given by:
Here,
Substituting the values given above, we have:
The answer close to the calculated value is
Note: Students should not get confused with the atomic number of
Formula used:
where
Complete step-by-step answer:
When electric current is passed through an atomic gas, the gas emits radiations of certain specific wavelengths. A spectrum is formed and this is called the Line emission spectrum. In this spectrum, groups of spectral lines are observed. These groups are named as spectral series. According to increasing wavelength, these series are named as Lyman series, Blamer series, Paschen series, Brackett series and Pfund series.
When electric current is passed through

Rydberg equation is given by:
Here,
Substituting the values given above, we have:
The answer close to the calculated value is
Note: Students should not get confused with the atomic number of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




