
Which of the following figures represent the variation of particle momentum and the associated de-Broglie wavelength?
(A)
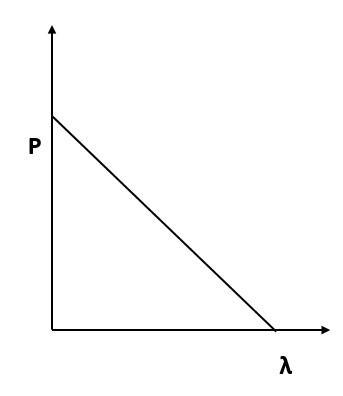
(B)

(C)
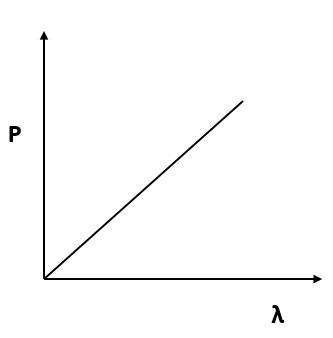
(D)
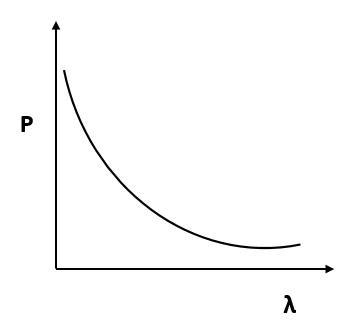




Answer
138k+ views
Hint: The relation between de Broglie wavelength and linear momentum is calculated from the energy of the photon. De Broglie’s equation equates de Broglie wavelength to the ratio of Planck’s constant and linear momentum.
Formula Used: The formulae used in the solution are given here.
Momentum of a photon is given by-
$P = \dfrac{E}{c} = \dfrac{h}{\lambda }$ where $E$ is the energy of the photon, $c$ is the speed of light in vacuum, $h$ is the Planck’s constant and $\lambda $ is the de Broglie wavelength.
Complete Step by Step Solution: The wavelength that is associated with an object in relation to its momentum and mass is known as de Broglie wavelength. A particle’s de Broglie wavelength is usually inversely proportional to its force.
Momentum of a photon is given by-
$P = \dfrac{E}{c} = \dfrac{h}{\lambda }$ where $E$ is the energy of the photon, $c$ is the speed of light in vacuum, $h$ is the Planck’s constant and $\lambda $ is the de Broglie wavelength.
According to de Broglie, $p = \dfrac{h}{\lambda }$ or $p\alpha \dfrac{1}{\lambda }$.
By this relation we can conclude that the linear momentum of a photon is inversely proportional to the de Broglie wavelength. The graph of $p$ vs $\lambda$ shall be a rectangular hyperbola.
It will look like,
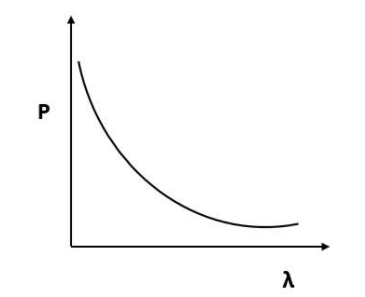
Hence, the correct answer is Option D.
Note: It is said that matter has a dual nature of wave-particles. De Broglie waves named after the discoverer Louis de Broglie, is the property of a material object that varies in time or space while behaving similar to waves. It is also called matter-waves. It holds great similarity to the dual nature of light which behaves as particle and wave, which has been proven experimentally.
The physicist Louis de Broglie suggested that particles might have both wave properties and particle properties. The wave nature of electrons was also detected experimentally to substantiate the suggestion of Louis de Broglie.
The objects which we see in day-to-day life have wavelengths which are very small and invisible, hence, we do not experience them as waves. However, de Broglie wavelengths are quite visible in the case of subatomic particles.
Formula Used: The formulae used in the solution are given here.
Momentum of a photon is given by-
$P = \dfrac{E}{c} = \dfrac{h}{\lambda }$ where $E$ is the energy of the photon, $c$ is the speed of light in vacuum, $h$ is the Planck’s constant and $\lambda $ is the de Broglie wavelength.
Complete Step by Step Solution: The wavelength that is associated with an object in relation to its momentum and mass is known as de Broglie wavelength. A particle’s de Broglie wavelength is usually inversely proportional to its force.
Momentum of a photon is given by-
$P = \dfrac{E}{c} = \dfrac{h}{\lambda }$ where $E$ is the energy of the photon, $c$ is the speed of light in vacuum, $h$ is the Planck’s constant and $\lambda $ is the de Broglie wavelength.
According to de Broglie, $p = \dfrac{h}{\lambda }$ or $p\alpha \dfrac{1}{\lambda }$.
By this relation we can conclude that the linear momentum of a photon is inversely proportional to the de Broglie wavelength. The graph of $p$ vs $\lambda$ shall be a rectangular hyperbola.
It will look like,

Hence, the correct answer is Option D.
Note: It is said that matter has a dual nature of wave-particles. De Broglie waves named after the discoverer Louis de Broglie, is the property of a material object that varies in time or space while behaving similar to waves. It is also called matter-waves. It holds great similarity to the dual nature of light which behaves as particle and wave, which has been proven experimentally.
The physicist Louis de Broglie suggested that particles might have both wave properties and particle properties. The wave nature of electrons was also detected experimentally to substantiate the suggestion of Louis de Broglie.
The objects which we see in day-to-day life have wavelengths which are very small and invisible, hence, we do not experience them as waves. However, de Broglie wavelengths are quite visible in the case of subatomic particles.
Recently Updated Pages
Young's Double Slit Experiment Step by Step Derivation

How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Physics Average Value and RMS Value JEE Main 2025

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

Displacement-Time Graph and Velocity-Time Graph for JEE




