Question

Which one of the following shows the highest magnetic moment?
a. $F{e^{2 + }}$
b. $C{o^{2 + }}$
c. $C{r^{3 + }}$
d. $N{i^{2 + }}$

Answer 1

Hint: The magnetic moment is given by $\sqrt {n(n + 2)} $, where n is the number of unpaired electrons. For example, in$C{r^{3 + }}$the number of unpaired electrons is 3$(4s3{d^3})$. Here n is 3, therefore the magnetic moment will be $\sqrt {3\left( {3 + 2} \right)} = 3.873$. This formula is used for spin-only cases.

Complete step by step answer:
We know that magnetic moment $(M) = \sqrt {n(n + 2)} $, where n is the number of unpaired electrons.
Now we have to determine the electronic configuration of each ion.
For $F{e^{2 + }}$it is $[Ar]3{d^6}$

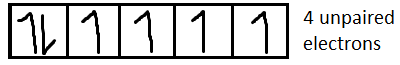
For $C{o^{2 + }}$it is $[Ar]3{d^7}$

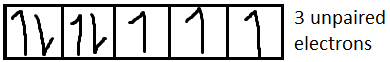
For $C{r^{3 + }}$it is $[Ar]3{d^3}$

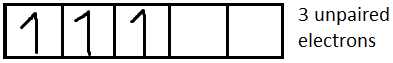
For $N{i^{2 + }}$it is $[Ar]3{d^8}$

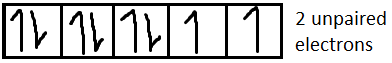
From the formula of magnetic moment, we can say that higher the number of unpaired electrons (n), more will be the amount of magnetic moment. In this case $F{e^{2 + }}$has the highest number of unpaired electrons (4 unpaired electrons) among the four given ions. Hence $F{e^{2 + }}$shows the highest magnetic moment.

So, the correct answer is Option A.

Note: Magnetic moments are often used in conjunction with electronic spectra to gain information about the oxidation number and stereochemistry of the central metal ion in coordination complexes. For first row transition metal ions in the free ion state, i.e. isolated ions in a vacuum, all 5 of the 3d orbitals are degenerate.
The formula used to calculate the spin-only magnetic moment can be written in two forms; the first based on the number of unpaired electrons n and the second based on the electron spin quantum number S. Since for each unpaired electron $n = 1$ and $S = \dfrac{1}{2}$then the two formulae are clearly related and the answer obtained must be identical.
${\mu _{so}} = \sqrt {n(n + 2)} $ and ${\mu _{so}} = \sqrt {4S(S + 1)} $

Whenever these types of questions appear, one has to consider the orbital contribution also. Remember the above formula is for spin-only cases. The orbital contribution might vary.