
Write all integers between
Answer
452.4k+ views
Hint: In order to find the solution of the given question that is write all the integers between
Complete step by step solution:
According to the question, we have to write the integers between
We will find the answer to this question with help of the number line of integers where a number line can be defined as a straight line with numbers placed at equal intervals or segments along its length. The number line of integers is expressed as the positive numbers are to the right of zero and the negative numbers are to the left of zero on a horizontal line. Now First look for
Here’s is the number line of integers:
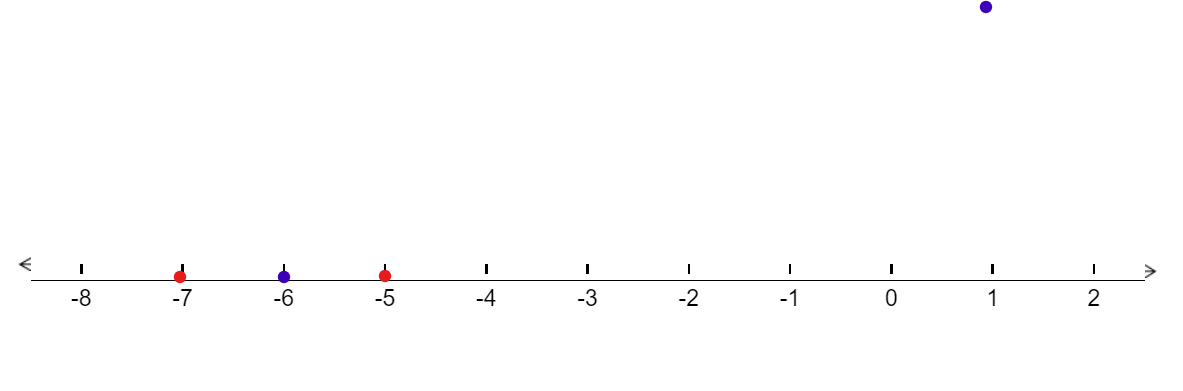
As we can see the points
Therefore, there’s only an integer between
Note: Students can be wrong with their answers by not using the concept of number line of integers. It’s important to remember a number line can be defined as a straight line with numbers placed at equal intervals or segments along its length. The number line of integers is expressed as the positive numbers are to the right of zero and the negative numbers are to the left of zero on a horizontal line.
Complete step by step solution:
According to the question, we have to write the integers between
We will find the answer to this question with help of the number line of integers where a number line can be defined as a straight line with numbers placed at equal intervals or segments along its length. The number line of integers is expressed as the positive numbers are to the right of zero and the negative numbers are to the left of zero on a horizontal line. Now First look for
Here’s is the number line of integers:
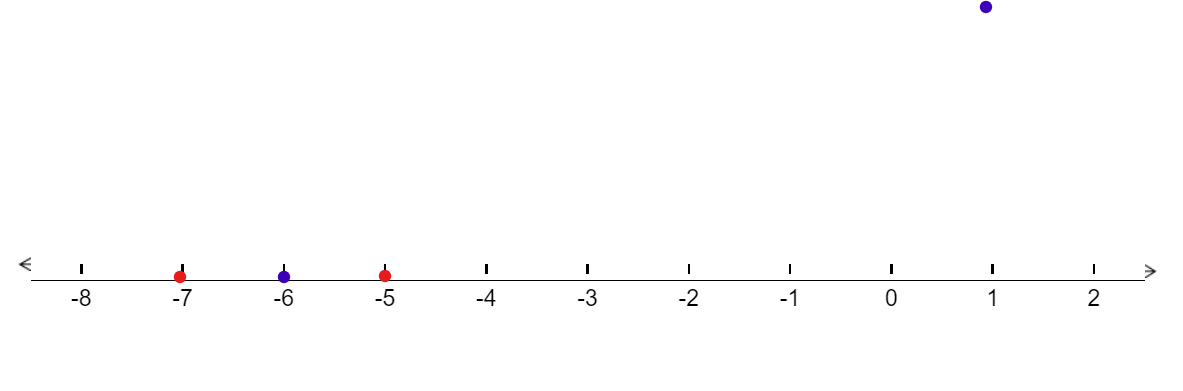
As we can see the points
Therefore, there’s only an integer between
Note: Students can be wrong with their answers by not using the concept of number line of integers. It’s important to remember a number line can be defined as a straight line with numbers placed at equal intervals or segments along its length. The number line of integers is expressed as the positive numbers are to the right of zero and the negative numbers are to the left of zero on a horizontal line.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
What is the full form of AD a After death b Anno domini class 6 social science CBSE

What is a joint family A Two or more families live class 6 social science CBSE

The planet nearest to earth is A Mercury B Venus C class 6 social science CBSE

How many time zones are in China class 6 social science CBSE

Give some important features of The Great Bath class 6 social studies CBSE

Write a letter to your headmasterheadmistress requesting class 6 english CBSE





