
Answer
444k+ views
Hint: We first find the slope-intercept form for any point $P\left( x,y \right)$ and also find the equation of the line. In this case we actually take the help of the origin where $O\left( 0,0 \right)$. Then we take two arbitrary points and place them on the equation of the line and find the value of the slope and its equation.
Complete step-by-step solution:
We need to find the equation of the line which is inclined at a given angle with the positive direction of the axis of x and cuts off a given intercept from the axis of y.
Suppose the line $\overleftrightarrow{AB}$ intersects the X-axis at D and the y-axis at C. if the line makes an angle $\alpha $ with the positive direction of X-axis and $\overleftrightarrow{OC}=c$. We have to find the equation of the line $\overleftrightarrow{AB}$.
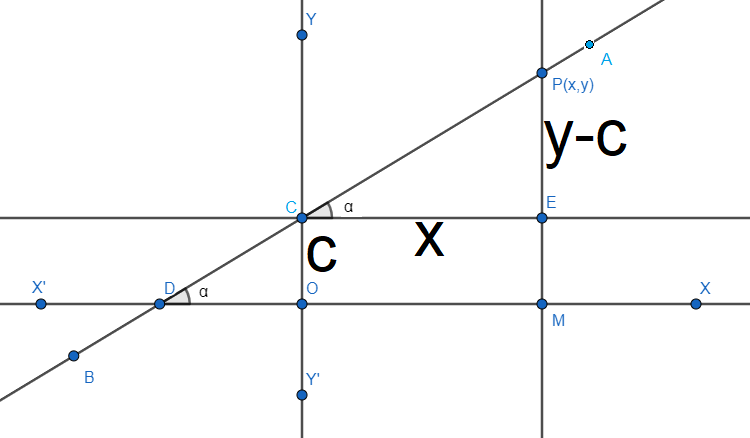
Let $P\left( x,y \right)$ be any point on the line $\overleftrightarrow{AB}$. We draw perpendicular $\overleftrightarrow{PM}$ on $\overleftrightarrow{OX}$ and $\overleftrightarrow{CE}$ perpendicular on $\overleftrightarrow{PM}$. Since, $CE||DM$, we have $\angle PCE=\angle CDM=\alpha $.
We also have $\overleftrightarrow{PM}=y;\overleftrightarrow{OC}=c;\overleftrightarrow{CE}=\overleftrightarrow{OM}=x$.
Then we have $\overleftrightarrow{PE}=\overleftrightarrow{PM}-\overleftrightarrow{EM}=\overleftrightarrow{PM}-\overleftrightarrow{OC}=y-c$.
Therefore, from the right-angled $\Delta PCE$, we get $\tan \alpha =\dfrac{\overleftrightarrow{PE}}{\overleftrightarrow{CE}}=\dfrac{y-c}{x}$.
We assume that the slope is m where $m=\tan \alpha $ which gives $m=\tan \alpha =\dfrac{y-c}{x}$.
The equation becomes $y=mx+c$.
Now if two points are given of the line where $P\left( x,y \right)$ and $Q\left( {{x}_{1}},{{y}_{1}} \right)$, the only change happens in the slope. For the above problem we took the second point as the origin.
Now both $P\left( x,y \right)$ and $Q\left( {{x}_{1}},{{y}_{1}} \right)$ goes through $y=mx+c$.
This gives $y=mx+c$ and ${{y}_{1}}=m{{x}_{1}}+c$. Subtracting we get $y-{{y}_{1}}=mx-m{{x}_{1}}=m\left( x-{{x}_{1}} \right)$.
The value of m becomes $m=\dfrac{\left( y-{{y}_{1}} \right)}{\left( x-{{x}_{1}} \right)}$. The equation becomes $\left( y-{{y}_{1}} \right)=m\left( x-{{x}_{1}} \right)$.
Note: We could have used the same process where we replace the origin value $O\left( 0,0 \right)$ with the point $Q\left( {{x}_{1}},{{y}_{1}} \right)$. The shifting of the origin will be sufficed to do that.
Complete step-by-step solution:
We need to find the equation of the line which is inclined at a given angle with the positive direction of the axis of x and cuts off a given intercept from the axis of y.
Suppose the line $\overleftrightarrow{AB}$ intersects the X-axis at D and the y-axis at C. if the line makes an angle $\alpha $ with the positive direction of X-axis and $\overleftrightarrow{OC}=c$. We have to find the equation of the line $\overleftrightarrow{AB}$.

Let $P\left( x,y \right)$ be any point on the line $\overleftrightarrow{AB}$. We draw perpendicular $\overleftrightarrow{PM}$ on $\overleftrightarrow{OX}$ and $\overleftrightarrow{CE}$ perpendicular on $\overleftrightarrow{PM}$. Since, $CE||DM$, we have $\angle PCE=\angle CDM=\alpha $.
We also have $\overleftrightarrow{PM}=y;\overleftrightarrow{OC}=c;\overleftrightarrow{CE}=\overleftrightarrow{OM}=x$.
Then we have $\overleftrightarrow{PE}=\overleftrightarrow{PM}-\overleftrightarrow{EM}=\overleftrightarrow{PM}-\overleftrightarrow{OC}=y-c$.
Therefore, from the right-angled $\Delta PCE$, we get $\tan \alpha =\dfrac{\overleftrightarrow{PE}}{\overleftrightarrow{CE}}=\dfrac{y-c}{x}$.
We assume that the slope is m where $m=\tan \alpha $ which gives $m=\tan \alpha =\dfrac{y-c}{x}$.
The equation becomes $y=mx+c$.
Now if two points are given of the line where $P\left( x,y \right)$ and $Q\left( {{x}_{1}},{{y}_{1}} \right)$, the only change happens in the slope. For the above problem we took the second point as the origin.
Now both $P\left( x,y \right)$ and $Q\left( {{x}_{1}},{{y}_{1}} \right)$ goes through $y=mx+c$.
This gives $y=mx+c$ and ${{y}_{1}}=m{{x}_{1}}+c$. Subtracting we get $y-{{y}_{1}}=mx-m{{x}_{1}}=m\left( x-{{x}_{1}} \right)$.
The value of m becomes $m=\dfrac{\left( y-{{y}_{1}} \right)}{\left( x-{{x}_{1}} \right)}$. The equation becomes $\left( y-{{y}_{1}} \right)=m\left( x-{{x}_{1}} \right)$.
Note: We could have used the same process where we replace the origin value $O\left( 0,0 \right)$ with the point $Q\left( {{x}_{1}},{{y}_{1}} \right)$. The shifting of the origin will be sufficed to do that.
Recently Updated Pages
There are two sample of HCI having molarity 1M and class 11 chemistry JEE_Main

For the reaction I + ClO3 + H2SO4 to Cl + HSO4 + I2 class 11 chemistry JEE_Main

What happens to the gravitational force between two class 11 physics NEET

In the reaction 2NH4 + + 6NO3 aq + 4H + aq to 6NO2g class 11 chemistry JEE_Main

A weightless rod is acted upon by upward parallel forces class 11 phy sec 1 JEE_Main

From a uniform circular disc of radius R and mass 9 class 11 physics JEE_Main

Trending doubts
Which is the longest day and shortest night in the class 11 sst CBSE

Who was the Governor general of India at the time of class 11 social science CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Define the term system surroundings open system closed class 11 chemistry CBSE

In a democracy the final decisionmaking power rests class 11 social science CBSE




