
Write the condition of collinearity of three points
Answer
476.7k+ views
Hint: First understand the definition of collinear points and their condition geometrically. Now, to write the condition of collinearity for the given points in the coordinate form, assume that these three points are forming a triangle and apply the formula for the area of the triangle given as
Complete step-by-step solution:
Here, we are provided with three points
(1)

Here we can see that we are able to draw a single straight line through the given three points P, Q, and R. So, they are collinear in nature.
(2)

Here, in this case, we cannot draw a straight line through P, Q, and R, so they are non – collinear.
Now, let us come to the question. We have to assume that the given points A, B, and C are collinear because only then we can find the condition for non – collinearity. So, we have,
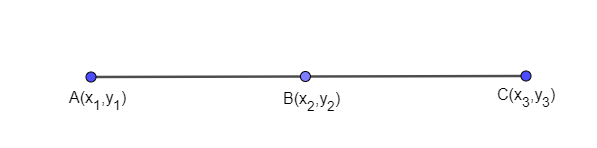
Since, we know that a triangle can be formed only when three points are non – collinear. Now, we can assume the above straight line formed by points A, B, and C as a triangle whose height is 0. So, we have,
Therefore, the condition for collinearity of three points is that its area should be 0.
Now, we have to find the collinearity condition in terms of the coordinate geometry. So, we know that the area relation of a triangle for points
So substituting area = 0, we get,
Hence, the above obtained relation is our answer.
Note: One may note that without understanding the definition and properties of collinear points we cannot solve the above question. You must remember the formula for the area of the triangle geometrically, in terms of base and height, and in coordinate geometry also. Remember that if we would have been provided with 4 points then we would have applied the formula of area of a quadrilateral and substituted it equal to 0 to get the answer.
Complete step-by-step solution:
Here, we are provided with three points
(1)

Here we can see that we are able to draw a single straight line through the given three points P, Q, and R. So, they are collinear in nature.
(2)

Here, in this case, we cannot draw a straight line through P, Q, and R, so they are non – collinear.
Now, let us come to the question. We have to assume that the given points A, B, and C are collinear because only then we can find the condition for non – collinearity. So, we have,

Since, we know that a triangle can be formed only when three points are non – collinear. Now, we can assume the above straight line formed by points A, B, and C as a triangle whose height is 0. So, we have,
Therefore, the condition for collinearity of three points is that its area should be 0.
Now, we have to find the collinearity condition in terms of the coordinate geometry. So, we know that the area relation of a triangle for points
So substituting area = 0, we get,
Hence, the above obtained relation is our answer.
Note: One may note that without understanding the definition and properties of collinear points we cannot solve the above question. You must remember the formula for the area of the triangle geometrically, in terms of base and height, and in coordinate geometry also. Remember that if we would have been provided with 4 points then we would have applied the formula of area of a quadrilateral and substituted it equal to 0 to get the answer.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




