
Write the converse of the Pythagoras Theorem and prove it.
Answer
534k+ views
Hint: Let’s consider a two triangles PQR and ABC, such that
Complete step-by-step answer:
The statement of the common Pythagoras theorem is that in a triangle, if the square of one side is equal to the sum of the squares of the other two sides, then the angle opposite to that side is the right angle.
Now we will prove it.
Consider a triangle ABC in which
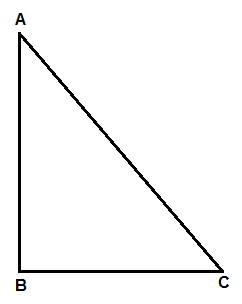
In this we have to prove that angle of B is
Now we will start by constructing a triangle PQR which is right angled at Q such that PQ = AB and QR = BC, as shown below:
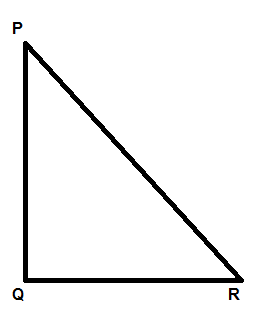
So, by using Pythagoras theorem in triangle PQR we can say that,
Now by constructing we said that PQ = AB and QR = BC then,
So, we can say that
Hence, PR = AC.
Now in triangle ABC and PQR we can say that,
i) AB = PQ which can be said by construction.
ii) BC = QR which can also be said by construction.
iii) AC = PR which is proved in the above section.
So, we can say that the triangle ABC and triangle PQR is congruent to each other using side-side –side congruence.
So, we can say that all sides and angles are equal by CPCT, i.e., corresponding part of concurrent triangle.
So,
We know
Hence the converse of Pythagoras theorem is proved.
Note: Students generally get confused while proving congruence of two triangles. They should know the rules of congruence by heart to prove these types of questions. This is a theorem, so proving it should not be a problem after the lesson is completed. Generally students don’t construct a triangle and get confused about how to prove this.
Complete step-by-step answer:
The statement of the common Pythagoras theorem is that in a triangle, if the square of one side is equal to the sum of the squares of the other two sides, then the angle opposite to that side is the right angle.
Now we will prove it.
Consider a triangle ABC in which

In this we have to prove that angle of B is
Now we will start by constructing a triangle PQR which is right angled at Q such that PQ = AB and QR = BC, as shown below:
So, by using Pythagoras theorem in triangle PQR we can say that,
Now by constructing we said that PQ = AB and QR = BC then,
So, we can say that
Hence, PR = AC.
Now in triangle ABC and PQR we can say that,
i) AB = PQ which can be said by construction.
ii) BC = QR which can also be said by construction.
iii) AC = PR which is proved in the above section.
So, we can say that the triangle ABC and triangle PQR is congruent to each other using side-side –side congruence.
So, we can say that all sides and angles are equal by CPCT, i.e., corresponding part of concurrent triangle.
So,
We know
Hence the converse of Pythagoras theorem is proved.
Note: Students generally get confused while proving congruence of two triangles. They should know the rules of congruence by heart to prove these types of questions. This is a theorem, so proving it should not be a problem after the lesson is completed. Generally students don’t construct a triangle and get confused about how to prove this.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





