Question

Write the expression for magnetic potential energy of a magnetic dipole kept in a uniform magnetic field and explain the terms.

Answer 1

Hint- This question demands us to state the expression for magnetic potential energy. A magnetic dipole moment will have the potential energy in a magnetic field that will depend on its magnetic field orientation.

Complete answer:

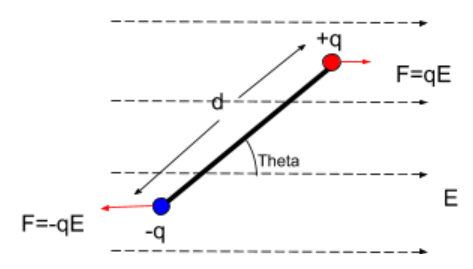
Because dipole sources, which can be seen as a present circuit with current I and area A are magnetic sources, energy is typically conveyed by magnetic dipole times: \[U(\theta ) = - \mu .B\] where \[\mu = IA\]. The energy is expressed like a scalar product and means that when the magnet moment is associated with the magnetic field the energy is lower.

Expression for magnetic potential energy of a magnetic dipole kept in a uniform magnetic field is

 \[ \Rightarrow U = - \vec m.\vec B\] Or \[ \Rightarrow U = mB\cos \theta \]

Where U= Magnetic potential energy
m− magnetic moment of the magnetic dipole
B− Uniform magnetic field.

Note- The magnetic dipole is the limit of either a closed loop of electrical current or a pair of poles as the size of the source is reduced to zero while keeping the magnetic moment constant. The electric dipole is a magnet analog, but the comparison is not perfect. Particular attention was never paid to a magnetic monopoly, the magnet equivalent of an electric charge. Therefore, the spin of elementary particles is related to one aspect of the magnetic dipole moment.