
Write the prime factorization of
Answer
500.7k+ views
1 likes
Hint:We have to find prime factorization of the given number by factor tree method.Prime factorization method is the method when dividing the given number by the first prime number
Complete step-by-step answer:
It is given that the number
Now, the prime factorization of
Prime factorization method is the method when dividing the given number by the first prime number which is
Then divide by
Factor Tree method
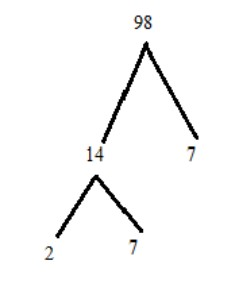
Factor is the number or algebraic expression which divides another number or expression with no remainder.
We can write
Now
Finally,
We don’t need to go further.
Our Factor tree method is complete
We got all prime factors by factor tree method.
∴
We got the required result.
Note:Factor of
All factor pairs of 98 are combination of two factors that when multiplied together give
They are
Smallest prime number is
Complete step-by-step answer:
It is given that the number
Now, the prime factorization of
Prime factorization method is the method when dividing the given number by the first prime number which is
Then divide by
Factor Tree method

Factor is the number or algebraic expression which divides another number or expression with no remainder.
We can write
Now
Finally,
We don’t need to go further.
Our Factor tree method is complete
We got all prime factors by factor tree method.
∴
We got the required result.
Note:Factor of
All factor pairs of 98 are combination of two factors that when multiplied together give
They are
Smallest prime number is
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

The minimum age required for becoming the Prime Minister class 8 social science CBSE

A girl of height 90 cm is walking away from the base class 8 maths CBSE

How was Junagadh State merged into the Indian Unio class 8 social science CBSE

Given that HCF 26 91 13 then the LCM of 26 91 is a class 8 maths CBSE




