
You are given one hundred
Answer
396.6k+ views
Hint: The smallest resistance is obtained when all the resistors are connected in parallel combination. The largest resistance can be obtained when the resistors are connected in series combination. The series combination of resistance is the algebraic sum of the values while the parallel resistance is the algebraic sum of the reciprocal of the values.
The formula for equivalent resistance
Where,
Here, the subscript “
The formula for equivalent resistance
Where,
Here, the subscript “
Complete answer:
A number of resistances are said to be connected in series if they are joined end to end and the same current flows through each one of them but different voltage across each of the components.
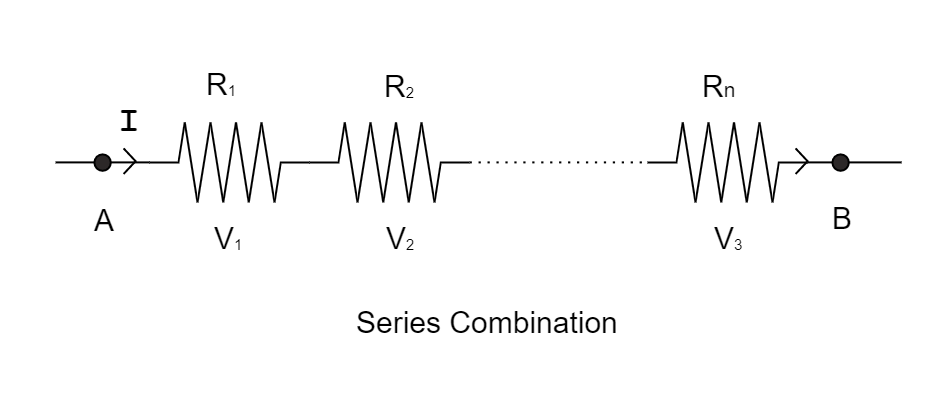
A number of resistances are said to be connected in parallel if they are joined between the same two ends with same voltage across each of the components but different current flow through each of them.
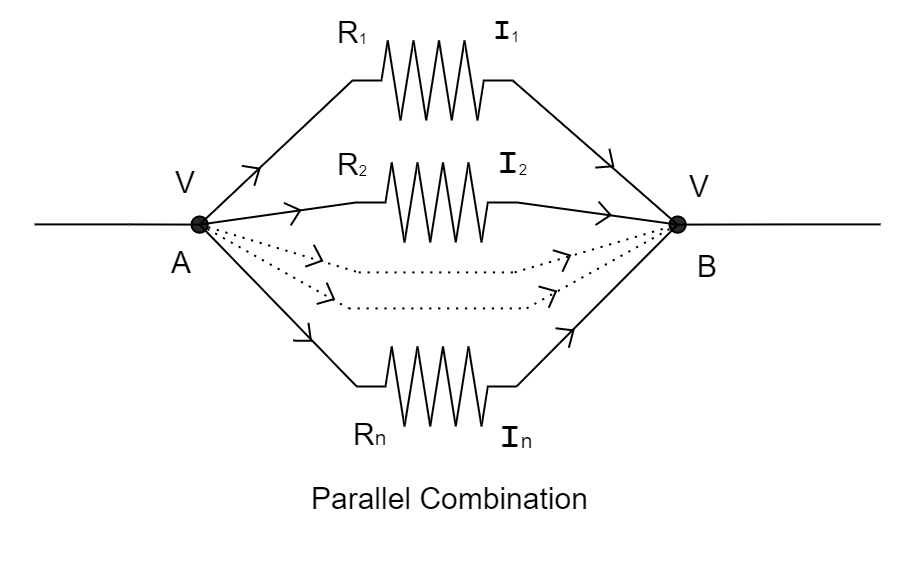
Given, The number of
Now, in order to obtain the smallest resistance using one hundred
By taking reciprocal on both sides, we get –
Now, in order to obtain the largest resistance using one hundred
Therefore, The required smallest resistance we can make with one hundred
Also, The required smallest resistance we can make with one hundred
Note:
The equivalent resistance will always be less than the value of the smallest resistance in parallel combination as the equivalent resistance is the sum of reciprocal of the individual resistances. The equivalent resistance series will always be greater than the individual resistances as it is the algebraic sum of individual resistance.
The formula for equivalent resistance
Where,
Here, the subscript “
The formula for equivalent resistance
Where,
Here, the subscript “
Complete answer:
A number of resistances are said to be connected in series if they are joined end to end and the same current flows through each one of them but different voltage across each of the components.

A number of resistances are said to be connected in parallel if they are joined between the same two ends with same voltage across each of the components but different current flow through each of them.

Given, The number of
Now, in order to obtain the smallest resistance using one hundred
By taking reciprocal on both sides, we get –
Now, in order to obtain the largest resistance using one hundred
Therefore, The required smallest resistance we can make with one hundred
Also, The required smallest resistance we can make with one hundred
Note:
The equivalent resistance will always be less than the value of the smallest resistance in parallel combination as the equivalent resistance is the sum of reciprocal of the individual resistances. The equivalent resistance series will always be greater than the individual resistances as it is the algebraic sum of individual resistance.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




