
You have two resistors of resistance
Answer
398.1k+ views
Hint: Firstly, we will connect the resistances in a series combination and calculate the equivalent resistance in this case. Then we will connect the two resistances in a parallel combination and then again we will calculate the equivalent resistance of these two resistors.
Complete step by step solution:
The two basic ways to connect any two resistances are, parallel combination and series combination. In a series combination the starting point of a resistor is the ending point of any other resistor. Whereas in a parallel combination, all the resistances have the same starting point and the ending point.
On connecting the two resistances in series,
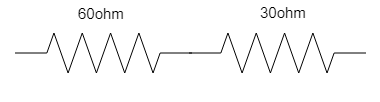
On putting the value of the two resistors, we get,
So, if we connect the two resistances in a series combination, then the value of the equivalent resistance is
Similarly, if we connect the two resistances in a parallel combination, then,
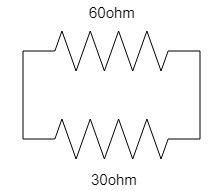
Since, the two resistances are in a parallel combination, so,
On putting the values of the two resistances, we get,
Now, we will take the LCM,
On taking the reciprocal on both the sides, we get,
So, if we connect the two resistances in a parallel combination, then the value of the equivalent resistance is
So, on connecting the two resistances in series combination, we get the equivalent resistance as
Note:
When two or more resistances are connected in a series combination, then the current flowing through all of them is the same but the potential difference of each of them is different. On the other hand, when two or more resistances are connected in a parallel combination, then the potential difference across each of them is the same but the current in each of them is different.
Complete step by step solution:
The two basic ways to connect any two resistances are, parallel combination and series combination. In a series combination the starting point of a resistor is the ending point of any other resistor. Whereas in a parallel combination, all the resistances have the same starting point and the ending point.
On connecting the two resistances in series,

On putting the value of the two resistors, we get,
So, if we connect the two resistances in a series combination, then the value of the equivalent resistance is
Similarly, if we connect the two resistances in a parallel combination, then,

Since, the two resistances are in a parallel combination, so,
On putting the values of the two resistances, we get,
Now, we will take the LCM,
On taking the reciprocal on both the sides, we get,
So, if we connect the two resistances in a parallel combination, then the value of the equivalent resistance is
So, on connecting the two resistances in series combination, we get the equivalent resistance as
Note:
When two or more resistances are connected in a series combination, then the current flowing through all of them is the same but the potential difference of each of them is different. On the other hand, when two or more resistances are connected in a parallel combination, then the potential difference across each of them is the same but the current in each of them is different.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




