Class 6 Maths Chapter 8 Playing with Constructions FREE PDF Download
FAQs on Playing with Constructions Class 6 Notes: CBSE Maths Chapter 8
1. What tools are used in geometric constructions?
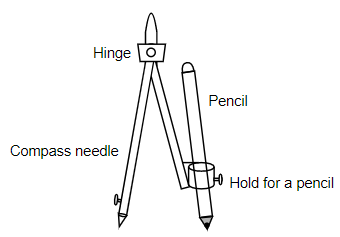
Geometric constructions typically use a compass and a ruler. The compass is used to draw circles and arcs, while the ruler is used to draw straight lines.
2. What is the purpose of using a compass in construction?
A compass helps draw accurate circles and arcs with a given radius, which is essential for many geometric constructions.
3. How do you construct a straight-line segment?
To construct a straight line segment, place the ruler on the paper and draw a line between two points, ensuring the line is straight and of the desired length.
4. What is an angle, and how can you construct one?
An angle is formed by two rays sharing a common endpoint called the vertex. To construct an angle, use the compass to draw two rays that meet at the vertex at the specified angle measurement.
5. How do you draw a circle with a given radius?
Place the compass point on the desired centre location, adjust the compass to the given radius, and then rotate the compass around the centre point to draw the circle.
6. What are the steps to construct a triangle with given side lengths?
Draw one side of the triangle using the ruler. Use the compass to draw circles with radii equal to the other two sides, intersecting at two points. Connect these intersection points to form the remaining sides of the triangle.
7. How can I check the accuracy of my constructions?
Use a protractor to measure angles and a ruler to check lengths. Ensure that your constructed shapes match the specifications given in the problem.
8. Why is it important to understand geometric constructions?
Understanding geometric constructions helps build precision, spatial awareness, and problem-solving skills, which are essential for more advanced mathematical concepts and real-world applications.
9. Can I use a digital tool for geometric constructions instead of a compass and ruler?
Yes, digital tools like geometry software can be used for construction, but it's important to practice with physical tools to develop a deeper understanding of the concepts.
10. How can I apply geometric constructions in real life?
Geometric constructions are useful in various real-life scenarios such as design, engineering, and architecture. They help in creating precise drawings and models in these fields.