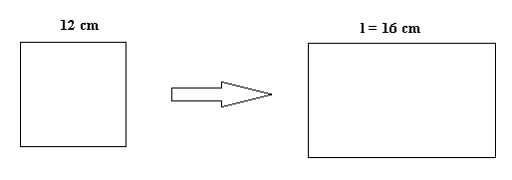
Revision Notes for CBSE Class 7 Maths Chapter 9 - Free PDF Download
FAQs on Perimeter and Area Class 7 Notes CBSE Maths Chapter 9 (Free PDF Download)
Q1. Where can I download the latest Revision Notes of Chapter 9 of Class 7 Maths?
Ans: You can download the latest Revision Notes of Chapter 9 of Class 7 Maths from the official website of Vedantu. It has all the notes of the chapters included in Class 7. They are well-curated and comprehensive. You will find important questions in those notes and it will be easier for you to understand the basics from those notes. You can use those notes a day before your exam to make revision easy. The notes are free of cost. They are also available on vedantu Mobile app.
Q2. How the concept of geometry with respect to the chapter is included in NCERT Solutions?
Ans:Geometry is a discipline that entails a thorough examination of all the figures that surround us, with a focus on determining the area and perimeter of those figures. Because of their importance in our daily lives, these factors must be calculated. As a result, the NCERT solutions for class 7 maths chapter 9 are available. The theories of area and perimeter of various forms such as the square, rectangle, parallelogram, triangle, and circle are covered in Perimeter and Area. When it comes to the area and perimeter of shapes, unit understanding is equally critical; so, the NCERT solutions class 7 mathematics chapter 9 The conversion of lengths and areas into dimensions is also explained by perimeter and area.
Q3. Shazli took a wire of length 44 cm and bent it into the shape of a circle. Find the radius of that circle. Also, find its area. If the same wire is bent into the shape of a square, what will be the length of each of its sides? Which figure encloses more area, the circle or the square? (Take π = 22/7)
Ans: In question,
Shazli took =44 cm of the wire
After that,
The wire bent in circle
Formula of circumference of circle = 2πr
44 = 2 × (22/7) × r
44 = 44/7 × r
(44 × 7)/44 = r
r = 7 cm
Circle’s formula = πr2
= 22/7 × 72
= 22/7 × 7 ×7
= 22 × 7
= 154 cm2
then,
Wire bent in square,
Length of every side of square = 44/4
= 11 cm
Square’s area = square’s length’s square
= 11^2
= 121 cm2
After comparison, it is proved that the circle has more area.
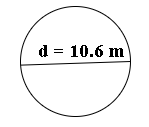
Q4. Find the cost of polishing a circular table-top of diameter 1.6 m, if the rate of polishing is ₹15/m2. (Take π = 3.14)
Ans: In question,
Circular table’s top diameter= 1.6 m
Radius is (r) = d/2
= 1.6/2
= 0.8 m
Now,
Circular table’s radius = πr2
= 3.14 × (0.8)^2
= 3.14 × 0.8 ×0.8
= 2.0096 m2
Cost incurred in polishing 1 m2 area = ₹ 15 [given]
Cost incurred in 2.0096 m2 area = ₹ 15 × 2.0096
= ₹ 30.144
Q5. If the circumference of a circular sheet is 154 m, find its radius. Also, find the area of the sheet. (Take π = 22/7)
Ans: In question,
Circle’s circumference = 154 m
After that
formula = 2πr
154 = 2 × (22/7) × r
154 = 44/7 × r
r = (154 × 7)/44
r = (14 × 7)/4
r = (7 × 7)/2
r = 49/2
r = 24.5 m
Then,
Circle’s area = πr2
= 22/7 × (24.5)^2
= 22/7 × 600.25
= 22 × 85.75
= 1886.5 m2
r= 24.5 and area is 1886.5