Class 9 Maths Revision Notes for Heron's Formula of Chapter 10 - Free PDF Download
FAQs on Heron's Formula Class 9 Notes CBSE Maths Chapter 10 (Free PDF Download)
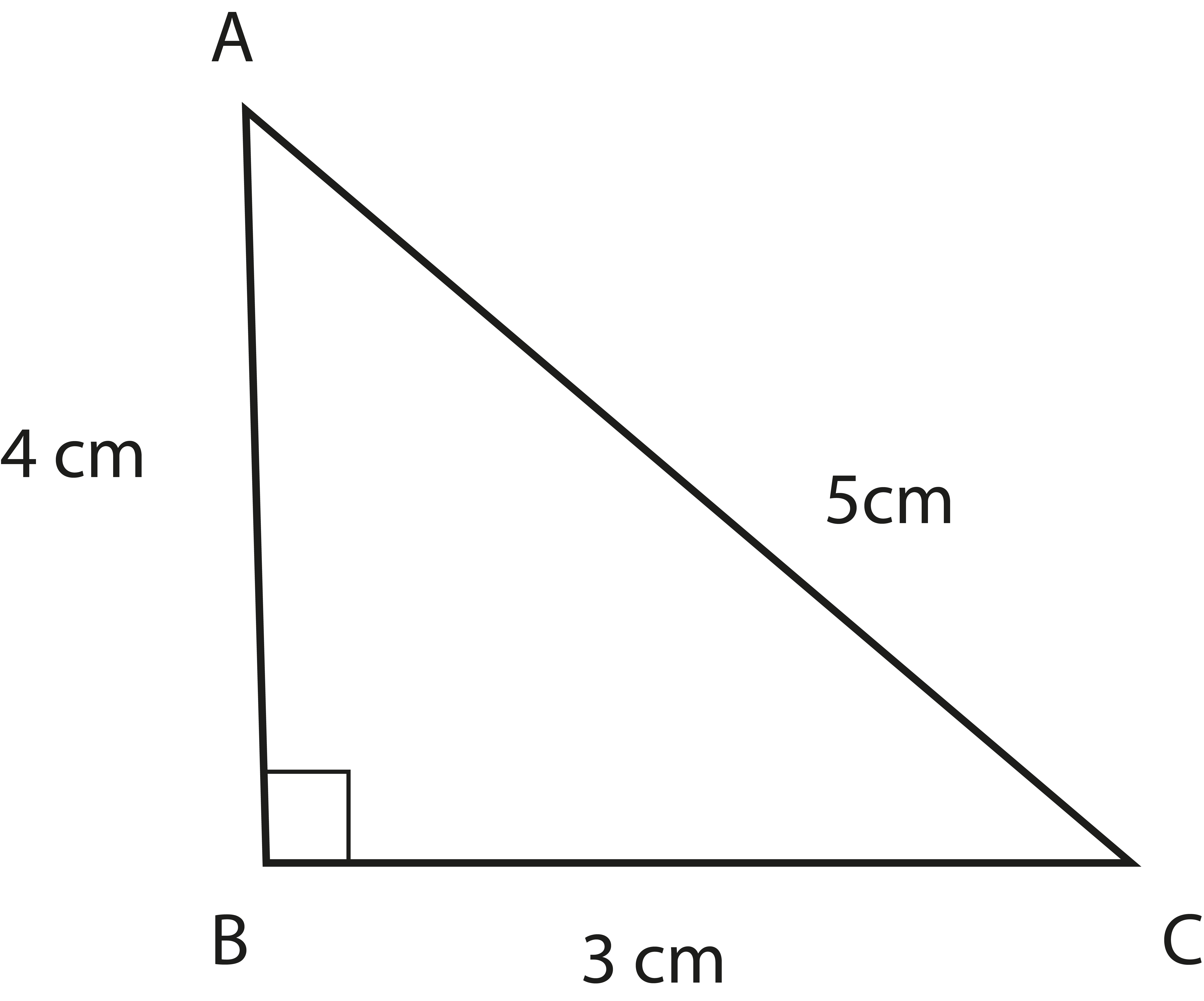
1. How to find the area of a triangle when the height is given?
The following formula is used to calculate the area of a triangle when its height is known:
Area = ½ × Base × Height
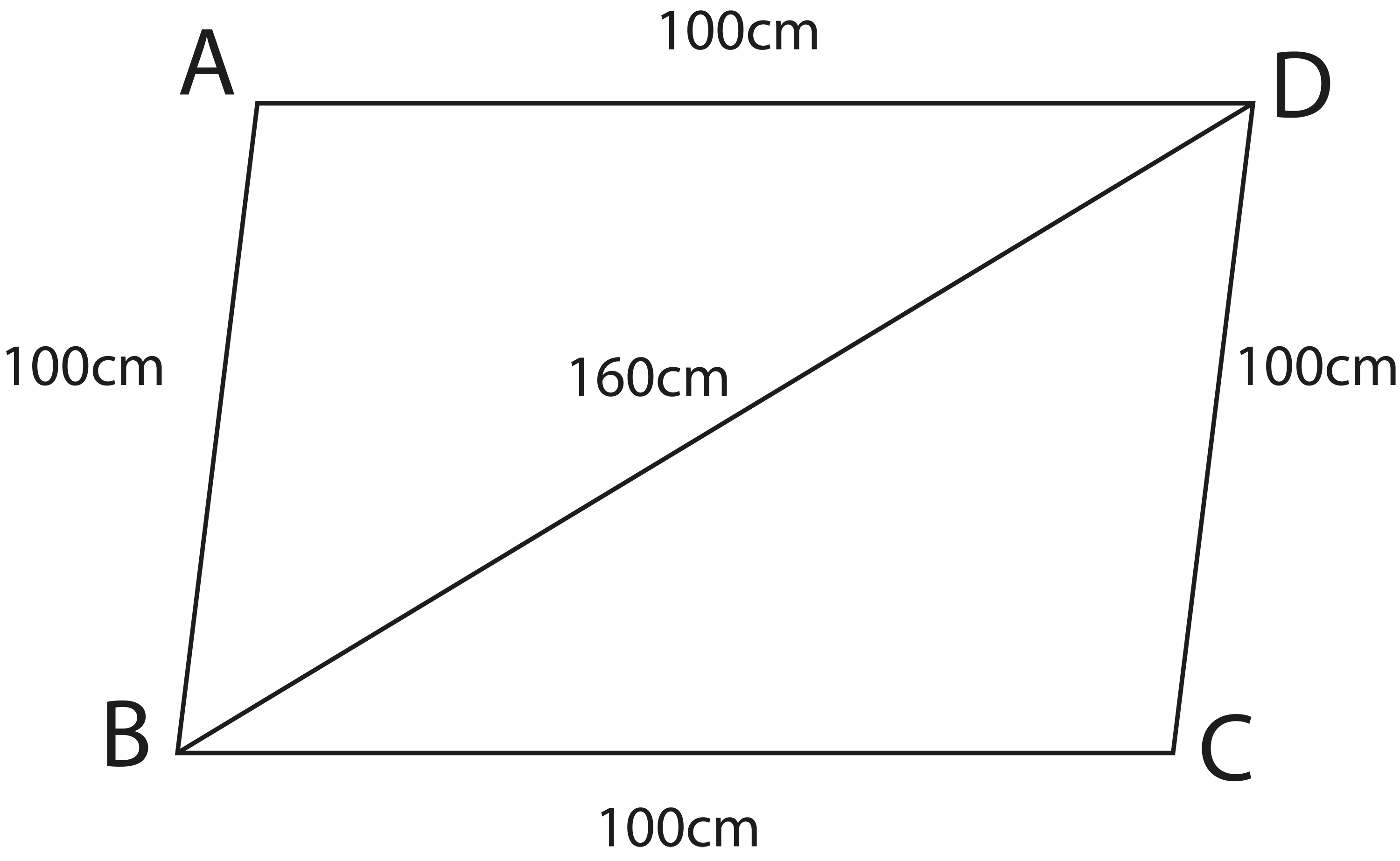
2. What is the semi-perimeter of a triangle?
Semi-perimeter of a triangle is the half of the perimeter of a triangle, and it is given as:
$S=\frac{a+b+c}{2}$
3. Who has introduced Herons formula?
Herons formula to find the area of a triangle is given by Mathematician Heron around 60 CE.
4. How will the revision notes help the students in their exams?
Students can revise calmly from our notes before the exam. It will not only be a revision but an effective revision. Before the exam going through the chapter becomes tedious and time-consuming, they can resort to these capsuled notes.
5. From where can I get the Class 9 Maths Chapter 10 revision notes?
Class 9 Maths Chapter 10 revision notes can be downloaded free from the pdf provided here. Students are given free access to our revision material owing to the needs of the revision.