Class 9 Maths Revision Notes for Introduction to Euclids Geometry of Chapter 5 - Free PDF Download
FAQs on Introduction to Euclids Geometry Class 9 Notes CBSE Maths Chapter 5 (Free PDF Download)
1. What is Euclid's geometry Class 9?
The term geometry consists of geo (earth) and metry (measure). Euclid’s geometry is a brief introduction to the world of solid figures and planes. Euclid, a famous Greek mathematician, is considered the father of geometry. In Euclid’s geometry, there are numerous axioms and postulates. It teaches you about the relationships between various things. It is the study of planes, geometrical shapes and figures. Having a basic understanding of geometry will also help you in your higher classes and various competitive exams. To know more students can refer to the vedantu app.
2. What is a point in geometry Class 9?
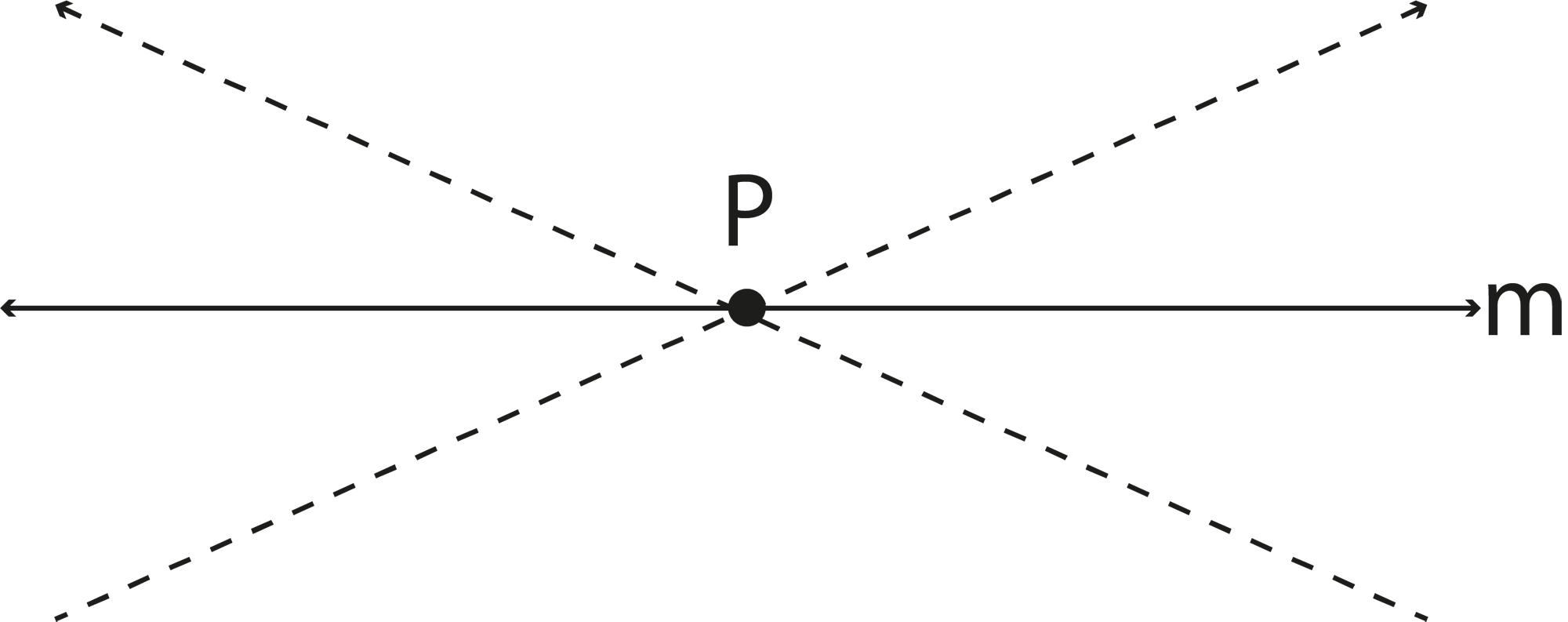
A point has no part. It is an element that has no breadth. Various points make a line. A point is characterised by the absence of dimensions and magnitude. It has no thickness. A point only occupies a space. A point can be defined only by geometric properties. A point in the domain of geometry is represented by various coordinates such as x and y in a two-dimensional plane and by x, y and z in a three-dimensional plane.
3. What are Euclid's 5 postulates for geometry?
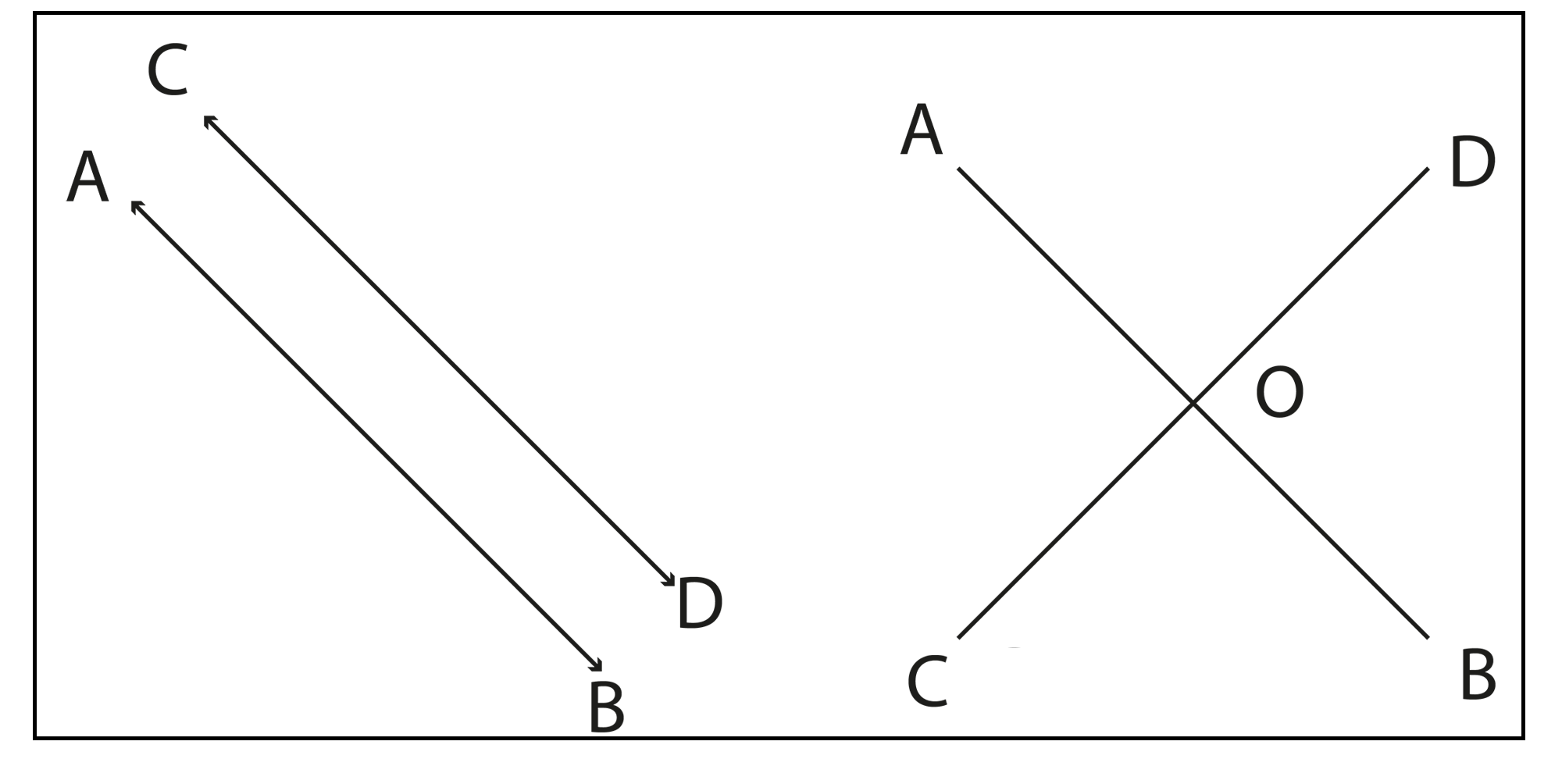
Euclid’s geometry is the study of various geometrical shapes and figures. He gave 5 postulates for plane geometry. First one among them is that if two or more things are equal to the same thing, then they are also equal to one another. Second is that if equals are added to equals then their whole is also equal. Third postulate states that if equals are subtracted from equals then their remainder will also be equal. Fourth, if things coincide with one another they are equal. Lastly, as parts make up the whole, the whole will be greater than par.
4. Where can I find Class 9 Maths Chapter 5 answers?
Chapter 5 of Class 9 Maths introduces you to Euclid’s geometry. The textbook provides you with all the basic information and all the necessary questions. Vedantu provides NCERT Solutions to all the questions from Chapter 5 of Class 9 Maths. These solutions are prepared by experts. When you have completed solving a set of questions, you can search for the solutions to make sure that your answer is correct and that you have followed all the necessary steps. Make sure to study revision notes before the exam to score good marks.
5. How many questions of Class 9 Geometry should I practice?
Geometry is a vast area. From a single concept, hundreds of questions can be framed. The most important thing before you start practising geometry questions is to have a strong understanding of the basics. If your basics are clear and strong, you will be able to answer any kind of question that may be asked from this chapter. Practice all your textbook questions. You can find various questions online as well. Write regular tests to check how well prepared you are and work on your shortcomings. To study more and revise the topics students can download the Class 9 maths notes free of cost from the vedantu website (vedantu.com).