Class 6 Maths NCERT Exemplar Solutions Chapter 4 Fractions & Decimals
Free PDF download of NCERT Exemplar for Class 6 Mathematics Chapter 4 - Fractions & Decimals solved by expert Mathematics teachers on Vedantu.com as per NCERT (CBSE) Book guidelines. All Chapter 4 - Fractions & Decimals exercise questions with solutions to help you to revise the complete syllabus and score more marks in your examinations.
You can also Download NCERT Solutions for Class 6 Mathematics to help you to revise the complete Syllabus and score more marks in your examinations.
Access NCERT Exemplar Solutions for Class 6 Mathematics Chapter 4 - Fraction and Decimals
In examples 1 and 2, write the correct answer from the given four options:
Example 1. Which of the following fractions is the smallest?
(A)
(B)
(C)
(D)
Ans: Option (C) is correct
The following fraction of
For,
For,
For,
In the following fractions
So, the option (C) is correct.
Example 2: 0.7625 lies between
(A)
(B)
(C)
(D)
Ans: Option (D) is correct.
By using the number line the
Example 3: Fill in the blanks so that the statement is true:
Decimal
Ans:
fraction for the decimal point is
Example 4: Fill in the blanks so that the statement is true:
Ans:
The subtract the numbers get
Example 5: State true or false:
The fraction
Ans: The given statement is false.
For fraction is
Example 6: Fill in the blanks using > or <
Ans: For the fraction
Now,
Therefore,
Example 7: Express
Ans: The fraction for
Decimal for the fraction
Example 8: Convert
Ans: Since
Example 9: Round off
Ans: For rounding off to tenths place, we look at the hundredths place.
Here the digit is
Hence, rounding off
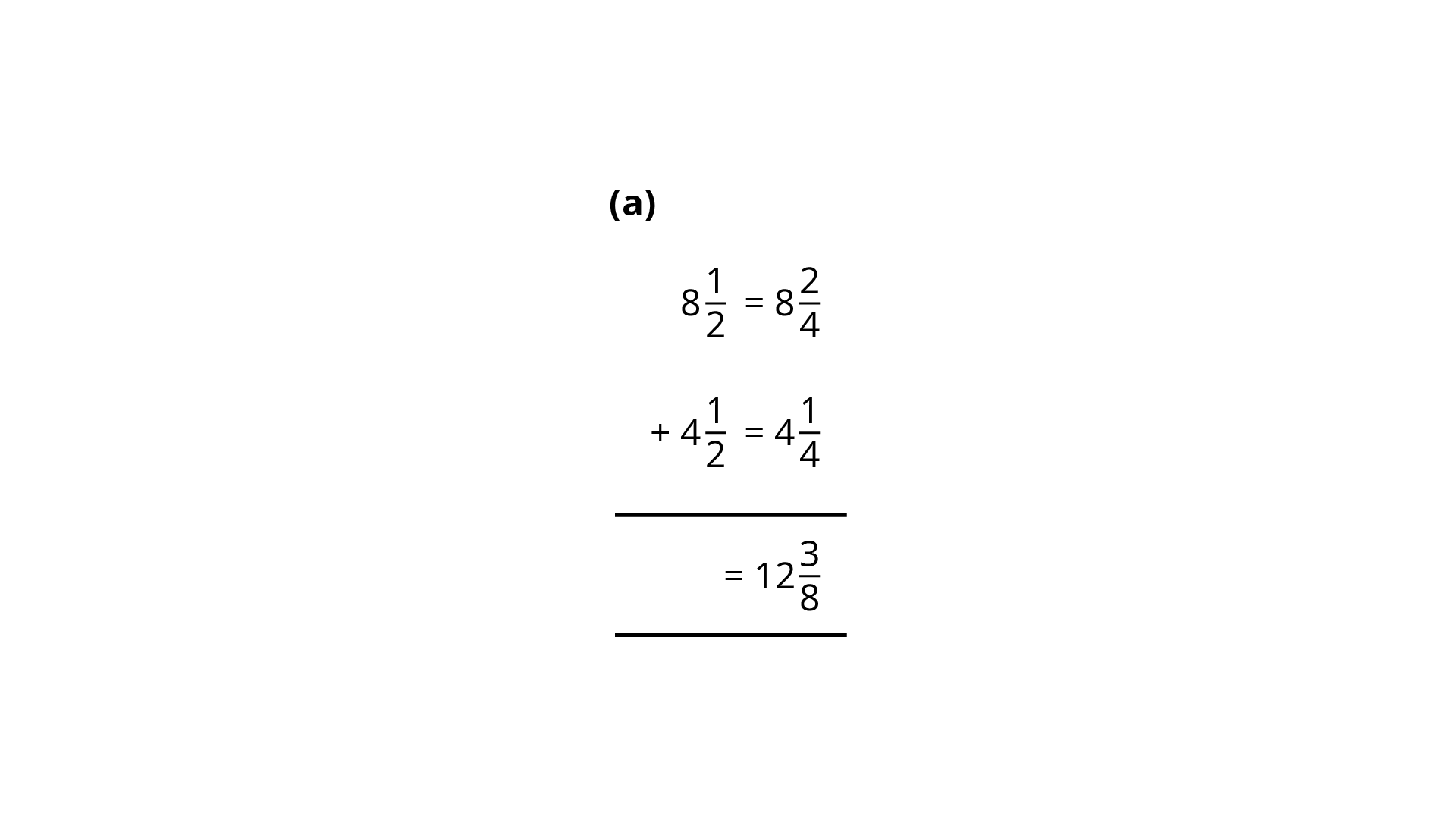
Example 10: Add the fractions
Ans: For the addition of fraction
Rewrite the mixed fraction
Example 11: What should be added to
Ans: Let the missing number is
So,
Hence, the required number to be added to
Example 12: Arrange the following in ascending order.
Ans: To arrange them from the smallest to the greatest number.
We arrange them as follows
using the idea of place value and comparing their digits at different places
Example 13: Gorang purchased
Ans: Since
Weight of apples
Weight of bananas
Weight of grapes
Weight of oranges
Total weight
Thus, total weight
Example 14: What is wrong in the following?
Ans: Writing
Converting into like fractions
Only numerators are added
In Questions 1 to 20, out of the four Options, only one Answer is Correct Choose the correct answer
1. The fraction which is not equal to
(A)
(B)
(C)
(D)
Ans: Option D is right
Check option
Check option
Check option
Check option
2. The two consecutive integers between which the fraction
(A)
(B)
(C)
(D)
Ans: Option B is right
Fundamental from fraction, if the value of a numerator of each fraction implies less than this value of denominator, ever lies between and
Hence,
3. When
(A)
(B)
(C)
(D)
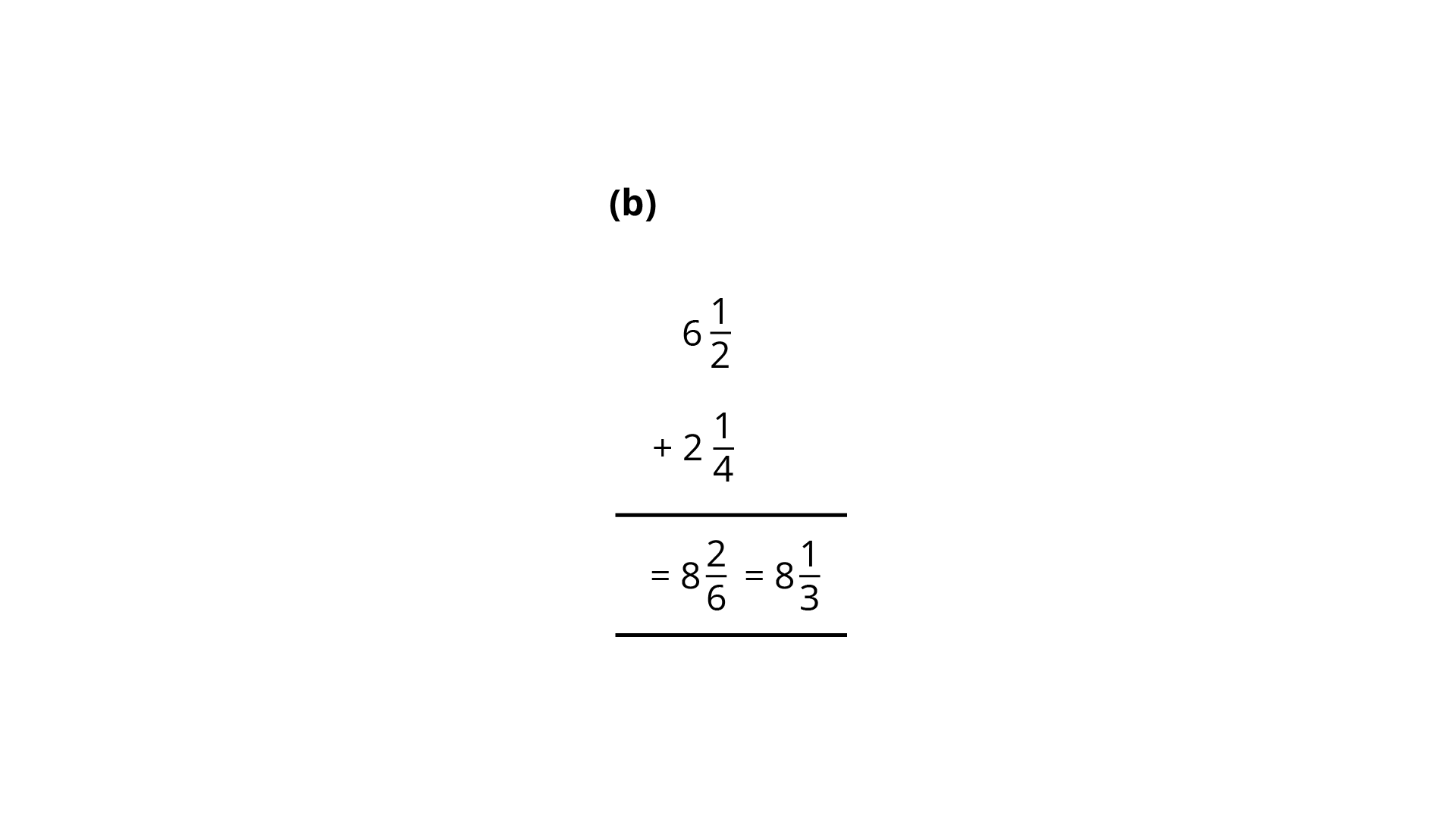
Ans: Option A is right
Since,
4. Which of the following is not in the lowest form?
(A)
(B)
(C)
(D)
Ans: Option B is right
In case of
Hence, already given in the simplest form.
In case of
In case of
Hence, already given in the simplest form.
In case of
Hence, already given in the simplest form.
Hence,
5. If
(A)
(B)
(C)
(D)
Ans: Option
Hence, Value of
6. Which of the following is not equal to the others?
(A)
(B)
(C)
(D)
Ans: Option
Check option
Check option
Check option
Check option D
Thus, Option
7. Which of the following fractions is the greatest?
(A)
(B)
(C)
(D)
Ans: Option B is right
In the above option given that, among the entire fraction with same numerator, the one with smaller denominator will be greatest.
Thus, required fraction is
8. Which of the following fractions is the smallest?
(A)
(B)
(C)
(D)
Ans: Option C is right
In the above option given that, among the entire fraction with same denominator, the one with smaller numerator will be smallest.
Thus, required fraction is
9. Sum of
(A)
(B)
(C)
(D)
Ans: Option A is right
Take the LCM of denominators
Thus, required fraction is
10. On subtracting
(A)
(B)
(C)
(D)
Ans: Option B is right
Take the LCM of denominators
Thus, required fraction is
11.
(A)
(B)
(C)
(D)
Ans: Option
Thus,
12.
(A)
(B)
(C)
(D)
Ans: Option B is right
Thus,
13.
(A)
(B)
(C)
(D)
Ans: Option
The mix fraction of
14. The mixed fraction
(A)
(B)
(C)
(D)
Ans: Option B is right
15.
(A)
(B)
(C)
(D)
Ans: Option
Sum of
16. Which of the following decimals is the greatest?
(A)
(B)
(C)
(D)
Ans: Option
Convert the above option into like decimal as
Thus, comparing the value of like decimal value,
17. Which of the following decimals is the smallest?
(A)
(B)
(C)
(D)
Ans: Option
Convert the above option into like decimal as
Thus, comparing the value of like decimal value,
18.
(A)
(B)
(C)
(D)
Ans: Option D is right
Round off the number
19.
(A)
(B)
(C)
(D)
Ans: Option B is right
Subtraction of
20. The decimal
(A)
(B)
(C)
(D)
Ans: Option A is right
Thus,
fraction for
Thus, required option
In Questions 21 to 44, fill in the blanks to make the Statements True
21. A number representing a part of a_____is called a fraction.
Ans: A number representing a part of a whole is called a fraction.
22. A fraction with denominator greater than the numerator is called a ____fraction.
Ans: A fraction with denominator greater than the numerator is called a proper fraction
23. fractions with the same denominator are called ____ fractions.
Ans: fractions with the same denominator are called like fractions.
24.
Ans:
25.
Ans:
26.
Ans:
27.
Ans:
28.
Ans:
29. The fraction
Ans: Given fraction is
The fraction
30. The fraction
Ans: Given fraction is
The fraction
31.
Ans:
32.
Ans: Given fraction is
33.
Ans: By long division the quotient and reminder will be
34.
Ans: The LCM of
Hence,
35. Decimal
Ans: Now,
Decimal
36. fraction
Ans: Given fraction is
fraction
37.
Ans: Explanation,
For the fractions denominator is same so add only numerator
So,
38.
Ans: Explanation,
For the fractions denominator is same so subtract only numerator
Hence,
39.
Ans: Explanation,
Rewrite the mixed fraction and add the numerator
Hence,
40.
Ans: Explanation,
Rewrite the mixed fraction and subtract the numerator
Therefore,
41.
Ans: Add the numbers
42.
Ans: Subtract the numbers
43. The value of 50 coins of 50 paisa
Ans:
The value of 50 coins of 50 paisa
44. 3 Hundredths
Ans: 3 Hundredths
3 tenths
3 Hundredths
In Each of the Questions 45 to 65, state Whether the Statement is True or False
45. fractions with the same numerator are called like fractions.
Ans: The given statement is False
The correct statement is fractions with the same denominator are called like fractions.
46. fraction
Ans: The statement is False
Because the fraction is in lowest form is
47. fractions
Ans: The statement is True
It is divide by same number
So, Both are equivalent.
48. The sum of two fractions is always a fraction.
Ans: The statement is True
Let, two fraction
Explanation,
This is a fraction.
49. The result obtained by subtracting a fraction from another fraction is necessarily a fraction.
Ans: The statement is False
Let, two fraction
Explanation,
which is not a fraction.
50. If a whole or an object is divided into a number of equal parts, then each part represents a fraction.
Ans: The statement is True
51. The place value of a digit at the tenths place is 10 times the same digit at the one's place.
Ans: The given statement is False
The place value of a digit at the tenths place is
52. The place value of a digit at the hundredths place is
Ans: The given statement is True
53. The decimal
Ans: The given statement is False
Since,
54. In the decimal form, fraction
Ans: The statement is True
55. The decimal
Ans: The given statement is False
By Long division method the quotient and reminder is
The correct statement
The decimal
56. The fraction represented by the shaded portion in the adjoining figure is
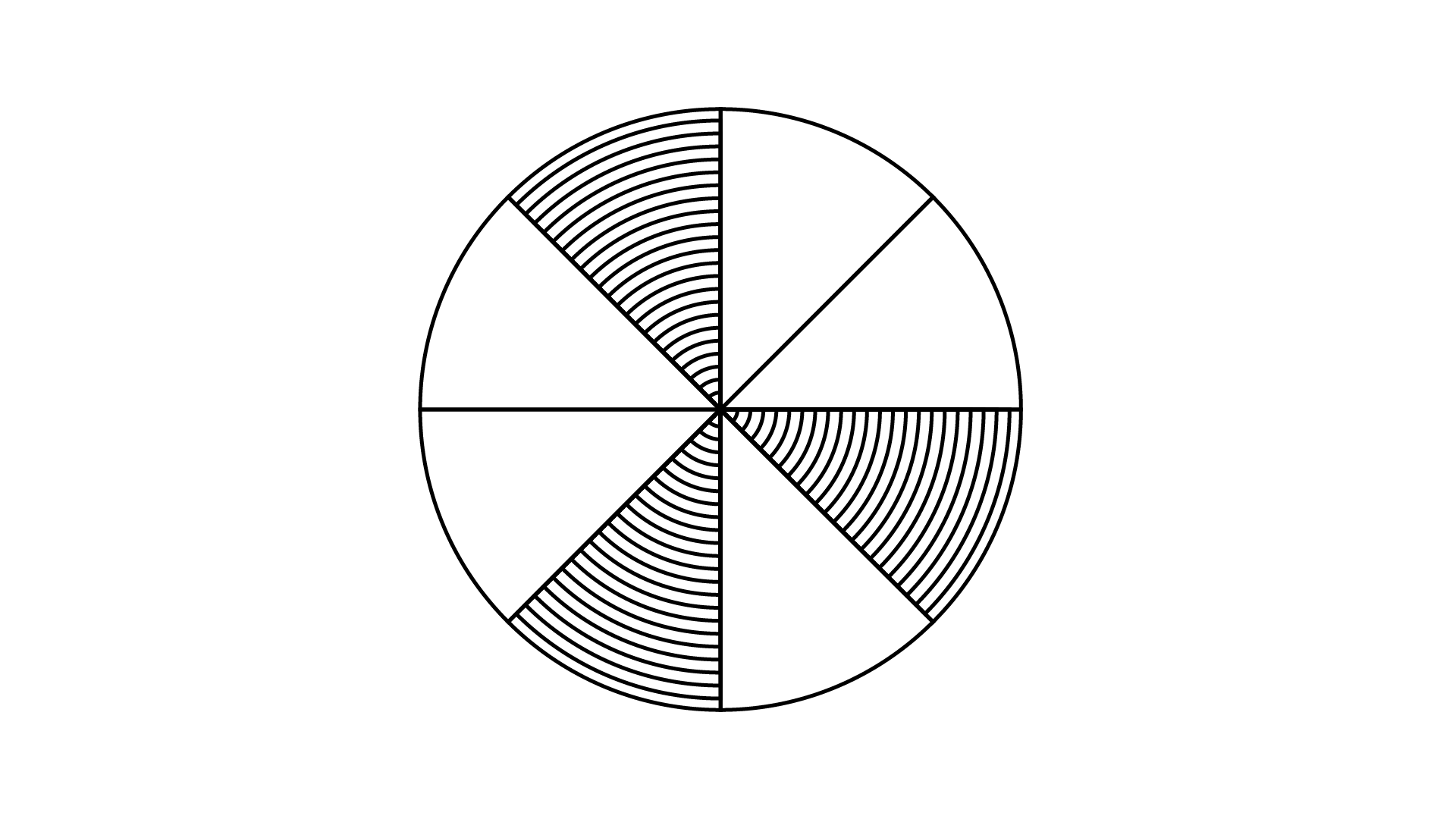
Ans: The given explanation is true.
The figure shows that, number of total part is
The required fraction is
57. The fraction represented by the un-shaded portion in the adjoining figure is
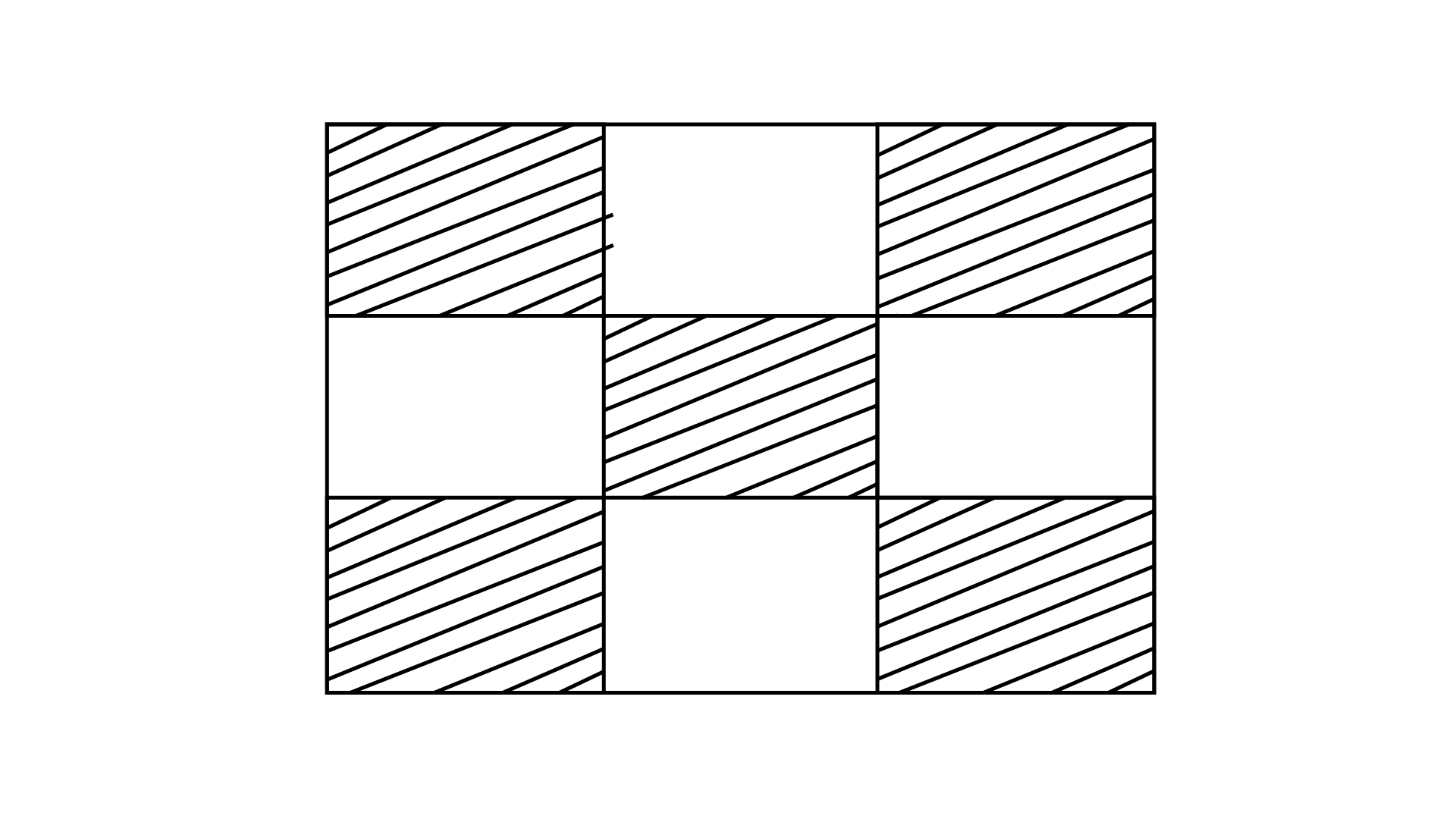
Ans: The given statement for the figure is false.
The figure shows that, number of total part is
The required fraction is
58.
Ans: The addition of the fraction is False
Denominator is same for the fractions so by adding the numerator
59.
Ans: The subtract of the fraction is False
Cross multiply the both fraction with each other.
60.
Ans: The addition of the fraction is True
61.
Ans: The addition of the numbers is False
62.
Ans: The subtraction of the two numbers is True
63.
Ans: The statement is True
Given fraction are like fraction.
Comparing the numerator, we get
64.
Ans: The given fraction type is False
Since, convert the
Compare the place value
Hence, the statement is
65.
Ans: The given number is True
Since, convert the
So,
In Each of the Questions 66 to 71, fill in the Blanks Using '>', '<' or '=':
66.
Ans: The L.C.M. of
Make it as like a fraction, multiply in numerator and denominator with the same digit
67.
Ans: The L.C.M of
Make it as like a fraction, multiply in numerator and denominator with the same digit
Therefore,
68.
Ans: The L.C.M of
Make it as like a fraction, multiply in numerator and denominator with the same digit
Hence,
69.
Ans: Since, convert the
Compare the place value of
Hence,
70.
Ans: Since, convert
The L.C.M. of
Make it as like a fraction, multiply in numerator and denominator with the same digit
71.
Ans: Since, convert
Therefore,
72. Write the fraction represented by the shaded portion of the adjoining figure:
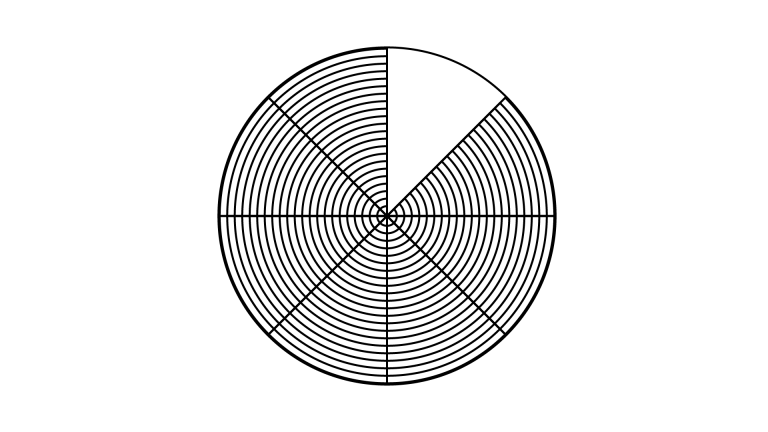
Ans: In the given figure, total parts in the figure is divided into
Total parts
Total shaded parts
Therefore,
fraction of unshaded portion
The required fraction is
73. Write the fraction represented by the un-shaded portion of the adjoining figure:
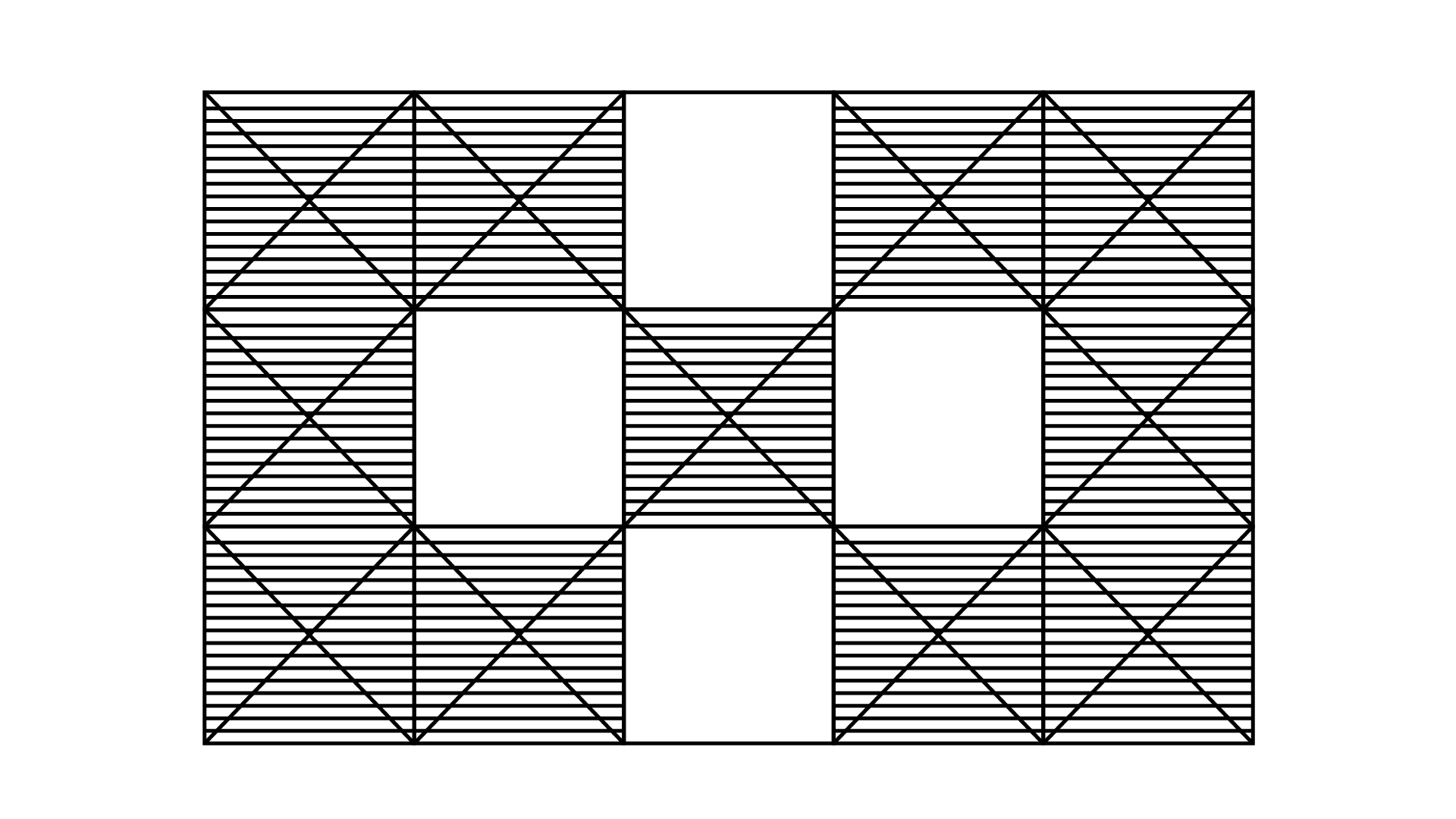
Ans: In the given figure, total parts in which figure has been divided into 15 and out of which 4 parts are unshaded.
Total parts
Total unshaded parts
Therefore,
fraction of unshaded portion
The required fraction is
74. Ali divided one fruit cake equally among six persons. What part of the cake he gave to each person?
Ans: Given data
Number of cake
Total no. of persons
Since,
Ali has to divide one fruit cake among 6 people,
75. Arrange
Ans: Given number
So, compare the place value of the digit and arrange them,
76. Write the largest four-digit decimal number less than1 using the digits
Ans: Given whole numbers are
Arrange them in descending order to make them large, after arranging the number will be
To make it a decimal number we will keep the whole number 8531 in the decimal point.
The required number is 0.8531, which is the largest four - digit decimal number less than 1.
77. Using the digits
Ans: For converting
After arranging whole numbers in ascending form it will be
To make the number decimal we will keep the numbers in decimal point.
So, the required number is
78. Express
Ans:
To get the denominator as whole number we will multiply denominator by
79. Express
Ans: We will convert the mixed fraction
We have,
Now we got
80. Express
Ans: We will convert the mixed fraction
We have,
Now,
81. Express
Ans: We can write 0.041 as
So,
Multiply numerator and denominator by
fraction for the decimal is
82. Express
Ans: We have,
The fraction for the decimal is
83. Convert
Ans: We know that,
84. Convert
Ans: We know that,
1 rupee
1 paisa
2009 paisa
The mixed fraction is Rs.
85. Convert
Ans: We know that,
1 meter
1 centimeter
86. Convert
Ans: We know that
Dividing numerator and denominator by
By long division method quotient and reminder is
The mixed fraction is
87. Arrange the fractions
Ans: Given fraction is
Take the LCM of
The
Now, arrange the following the ascending order
The ascending order of the function is
88. Arrange the fractions
Ans: The given fraction is
Take the LCM of
The
Now, arrange the following the descending order
The descending order of the fraction is
89. Write
Ans: Given fraction
If multiply 4 by 11 it become 44
Now,
90. Write
Ans: Given fraction
If multiply
Now,
91. Write
Ans: Given fraction is
Now divide the numbers and quotient and reminder of the numbers is
So, the mixed fraction of the
92. Round off
Ans:
93. Round off
Ans:
94. Round off
Solution:
95. Add the fractions
Ans: Given fraction
Denominator is different so need to cross multiply
Cross multiply the both fraction with each other.
The addition of fraction
96. Add the fractions
Ans: Given fraction is
Now, rewrite the mixed fraction
Add the following fraction
Cross multiply the both fraction with each other.
The addition of fractions
97. Subtract
Ans: Given fraction
Cross multiply the both fraction with each other.
The subtraction of
98. Subtract
Ans: Given fraction
Now, rewrite the mixed fraction
Subtract the following fraction,
Cross multiply the both fraction with each other.
The subtraction of
99. Subtract
Ans: Given fraction
Now, rewrite the mixed fraction
Now,
Now,
Subtract the following fraction,
The subtraction of the
100. Add
Ans: The given fraction
Now, rewrite the mixed fraction
Now,
The addition of the
101. Katrina rode her bicycle
Ans: The distance covers by Katrina in the morning
The distance covers by Katrina in the evening
Total distance travelled by her
Distance travelled altogether by Katrina in a day is
102. A rectangle is divided into a certain number of equal parts. If
Ans: Let, the number of parts in which the rectangle has been divided be
According to the question,
Cross- multiplication,
64 are the parts in which the rectangle has been divided.
103. Grip size of a tennis racquet is
Ans: Given, Grip size of a tennis racquet is
Now, rewrite the mixed fraction
104. On an average
Let that total food for the eaten is 1 and out of these,
Now, available for the next level of the consumer in a food chain is
The fraction of food eaten is not available for next level is
105. Mr. Rajan got a job at the age of 24 years and he got retired from the job at the age of
Ans: Given,
Mr. Rajan got a job at the age of 24 years
He got retired from the job at the age of
Total working year
The required fraction
The fraction of his age till retirement was he in the job is
106. The food we eat remains in the stomach for a maximum of 4 hours. For what fraction of a day, does it remain there?
Ans: Given,
The food we eat remains in the stomach for a maximum of 4 hours
Total number of hours in a day is
The required fraction
The fraction of the day food remains in the stomach is
107. What should be added to
Ans: Let
Now,
The required number is
The number that should be added to
108. Alok purchased
Ans: Convert all the data in a signal unit,
Given,
Alok purchased
Total weight is
Total weight of the purchase is
The total weight of purchase of Alok in kilograms is
109. Arrange in ascending order:
Ans: Since, all the decimals are already given in like fractions,
Arranging them in ascending order, we get
110. Add the following:
Ans: We have,
To add the above decimals, we must convert them into like decimals first.
Writing
So,
Addition of
111. It was estimated that because of people switching to Metro trains, about
(i) The quantity of diesel saved to the quantity of petrol saved.
Ans: Given,
By the end of the year 2007 Metro trains was saved
(ii) The quantity of diesel saved to the quantity of CNG saved.
Ans:
The quantity of diesel saved to quantity of petrol is
The quantity of diesel saved to the quantity of CNG is
112. The energy content of different foods are as follows
Food | Energy Content per kg |
Wheat | |
Rice | |
Potatoes (Cooked) | |
Milk |
Which food provides the least energy and which provides the maximum?
Express the least energy as a fraction of the maximum energy
Ans: From the given table it is clear that Milk provides the least energy and Rice provides the maximum
Ratio of the least energy to the maximum energy
The least energy as a fraction of maximum energy is
113. A cup is
Ans: Total fill required of a cup of milk is 1
Given that Cup is
The remaining part of the cup which is still to be filled by milk
The part of cup is still to be filled by milk to make it full is
114. Mary bought
Ans: Mary bought
She used
Left lace
Rewrite the fraction in same denominator
The lace left with her is
115. When Sunita weighed herself on Monday, she found that she had gained
Ans: Sunita had gained
Earlier her weight was
Her total weight on Monday
Her weight on Monday was
116. Sunil purchased
Ans: Monday Sunil purchased
Tuesday Sunil purchased
Total quantity of juice Sunil purchased in two days
Litres of juice he purchased in two days is
117. Nazima gave
Ans: Total quantity of juice
Nazima gave to her friends
The required quantity of juice she is left with
The litre of juice left with her is
118. Roma gave a wooden board of length
Ans: Total length of wood
Wood used by carpenter
Remaining piece of wood length
The length of the remaining piece is
119. Nasir travelled
Ans: Nasir travel
Walked
Total distance covered by Nasir
The distance he travelled to reach the town is
120. The fish caught by Neetu was of weight
Ans: The weight of fish caught by Neetu
The weight of fish caught by Narendra
Neetus fish weigh more than that of Narendra by
Neetu fish weighed
121. Neelam's father needs
Ans: Neelam's father needs
Total cloths buy by her father
The total cloth Neelam should buy is
122. What is wrong in the following additions?
Ans: Equal denominators added.
Ans: Numerator and denominator are added.
123. Which one is greater?
Ans: Know that
Now,
So,
124. Match the fractions of Column I with the shaded or marked portion of figures of Column II:
Column I | Column II |
(i) | (A) 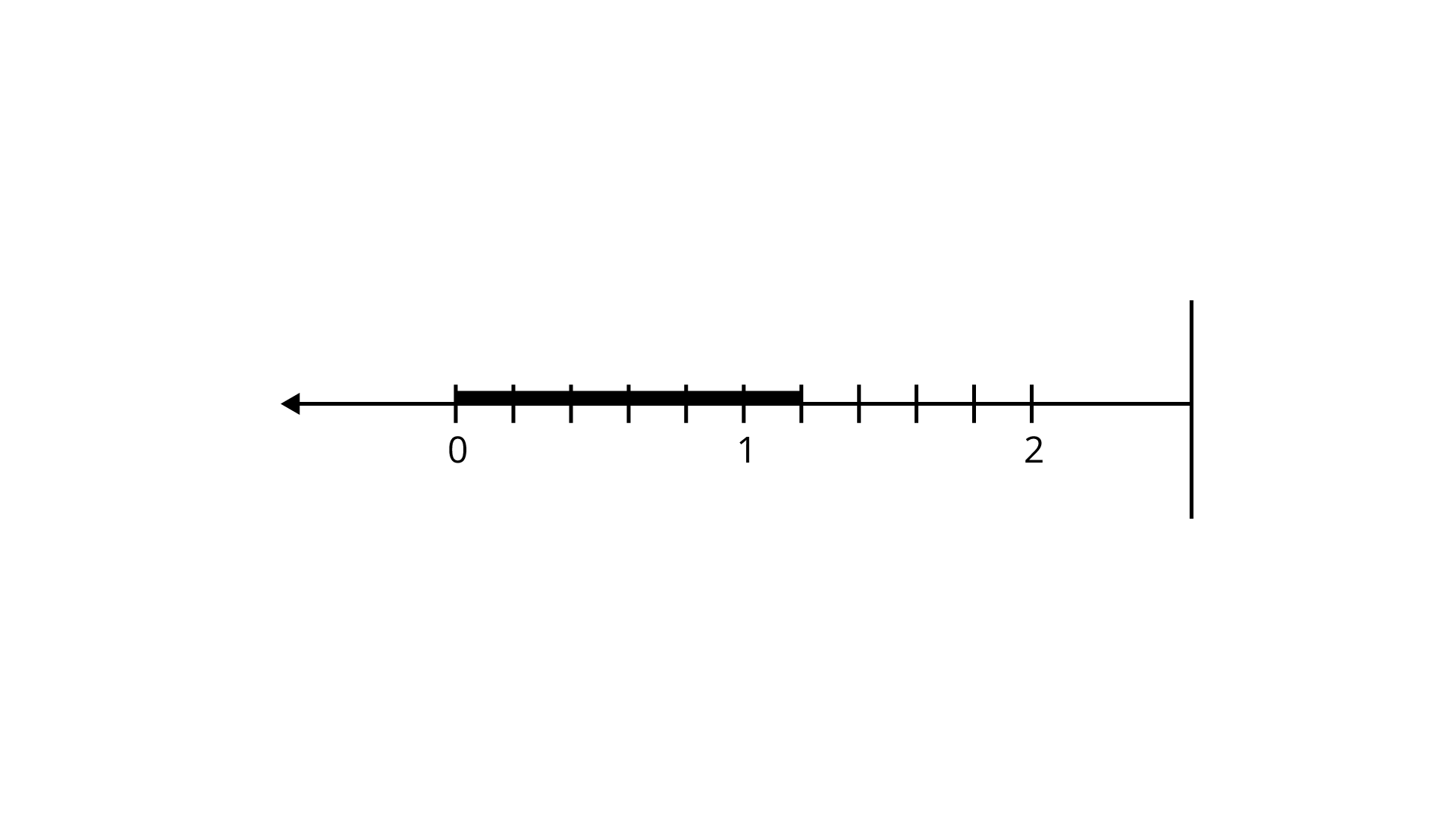
|
(ii) | (B) 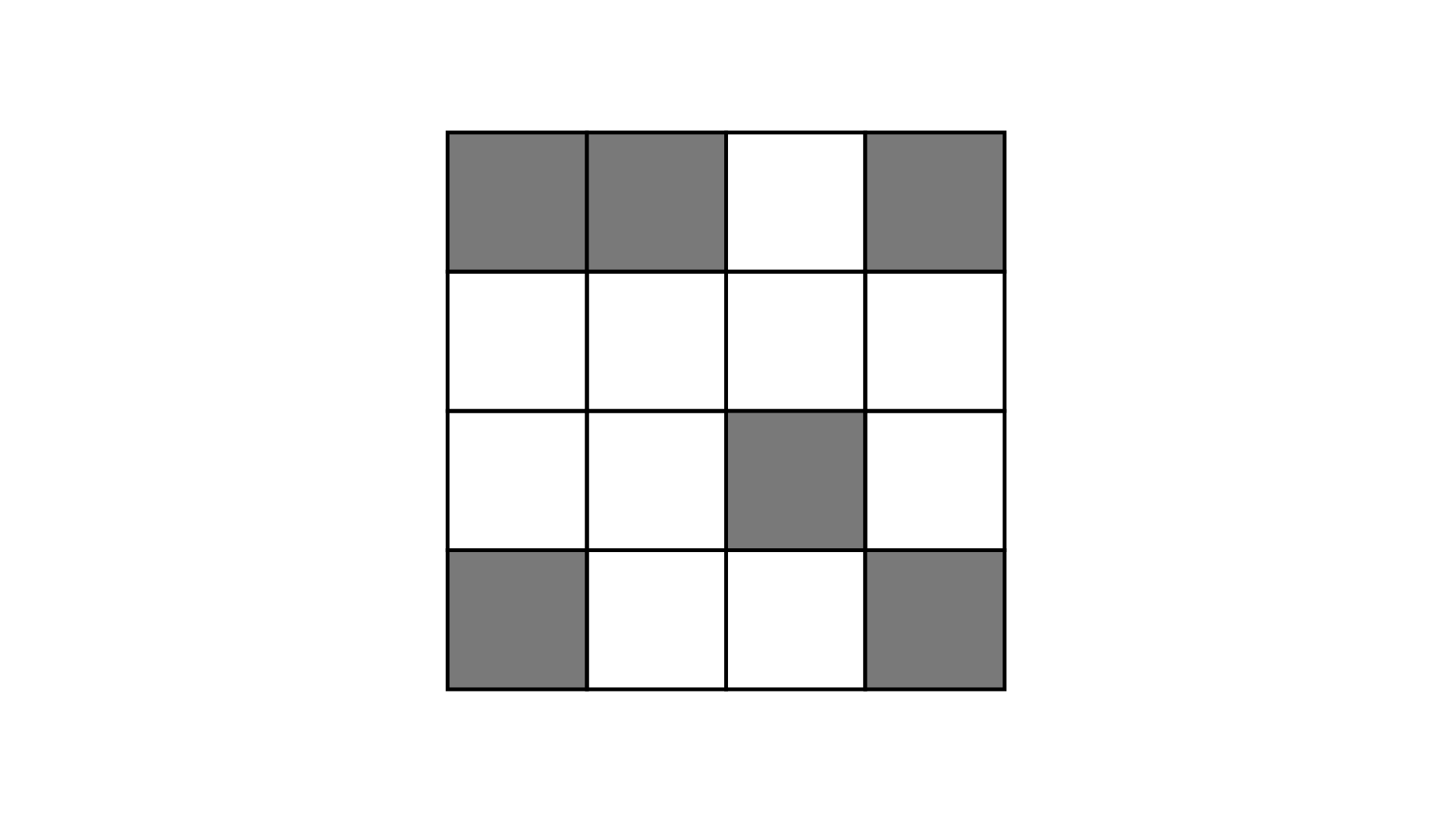
|
(iii) | (C) 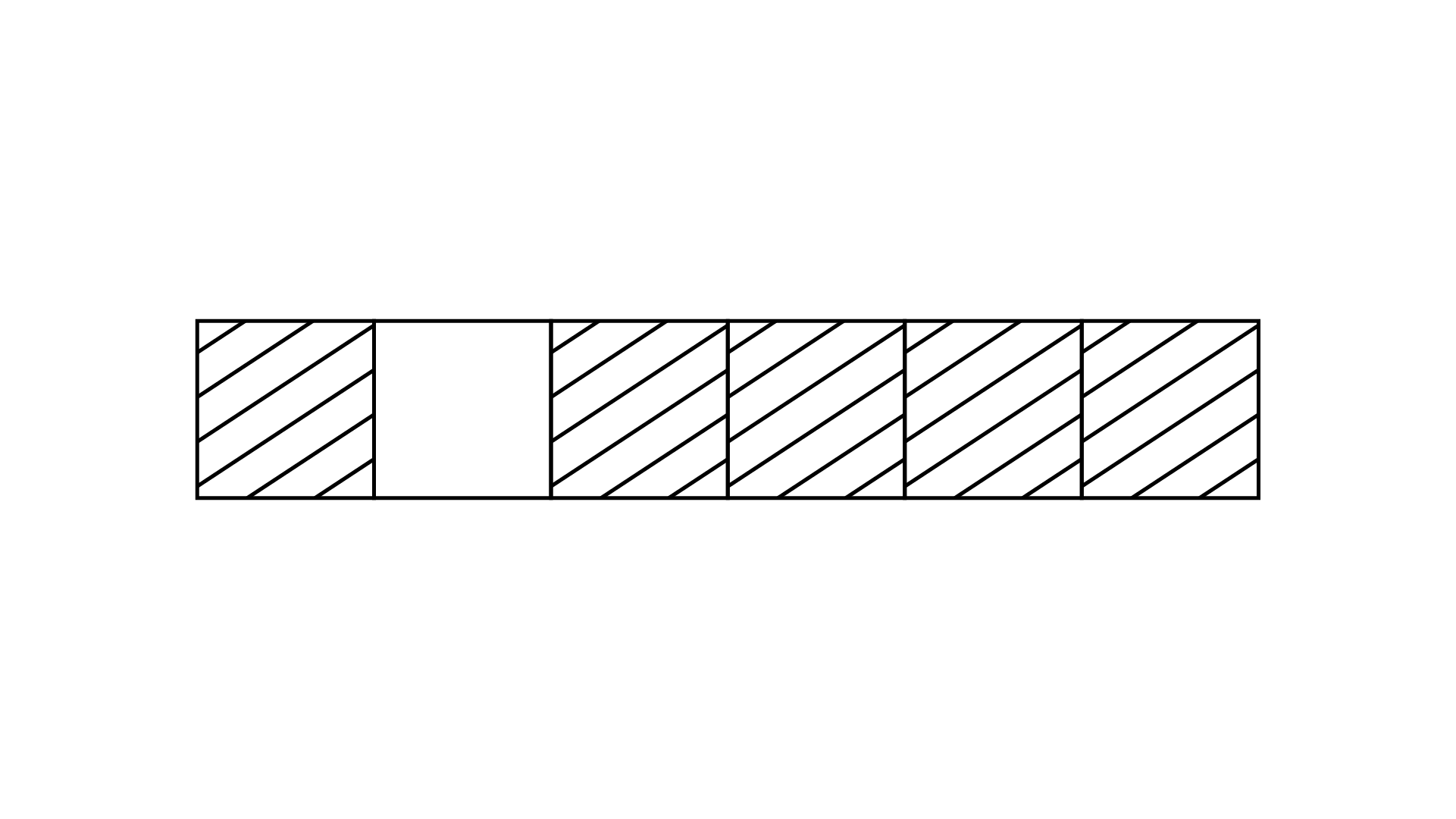
|
(iv) | (D) 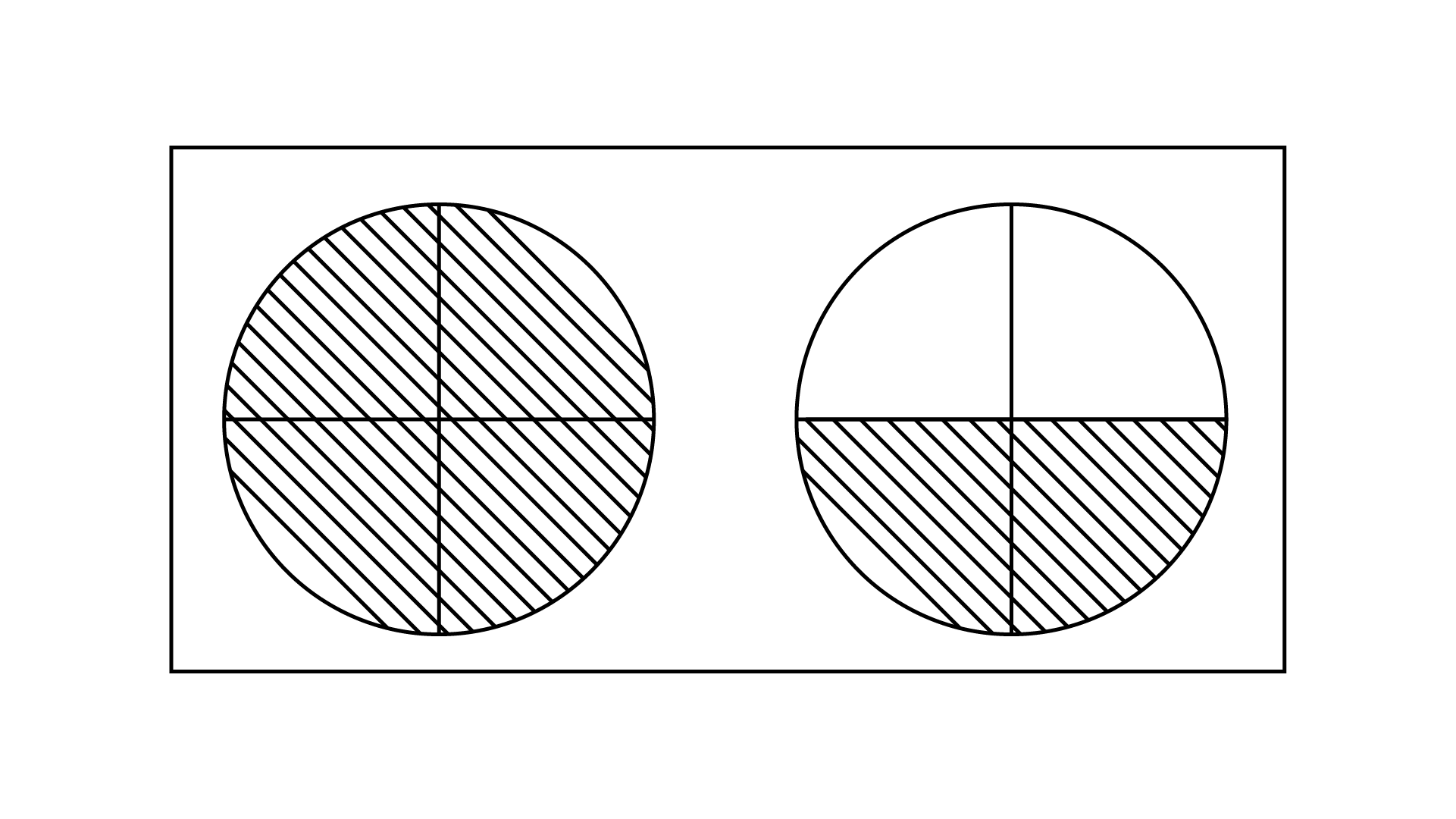
|
(E) 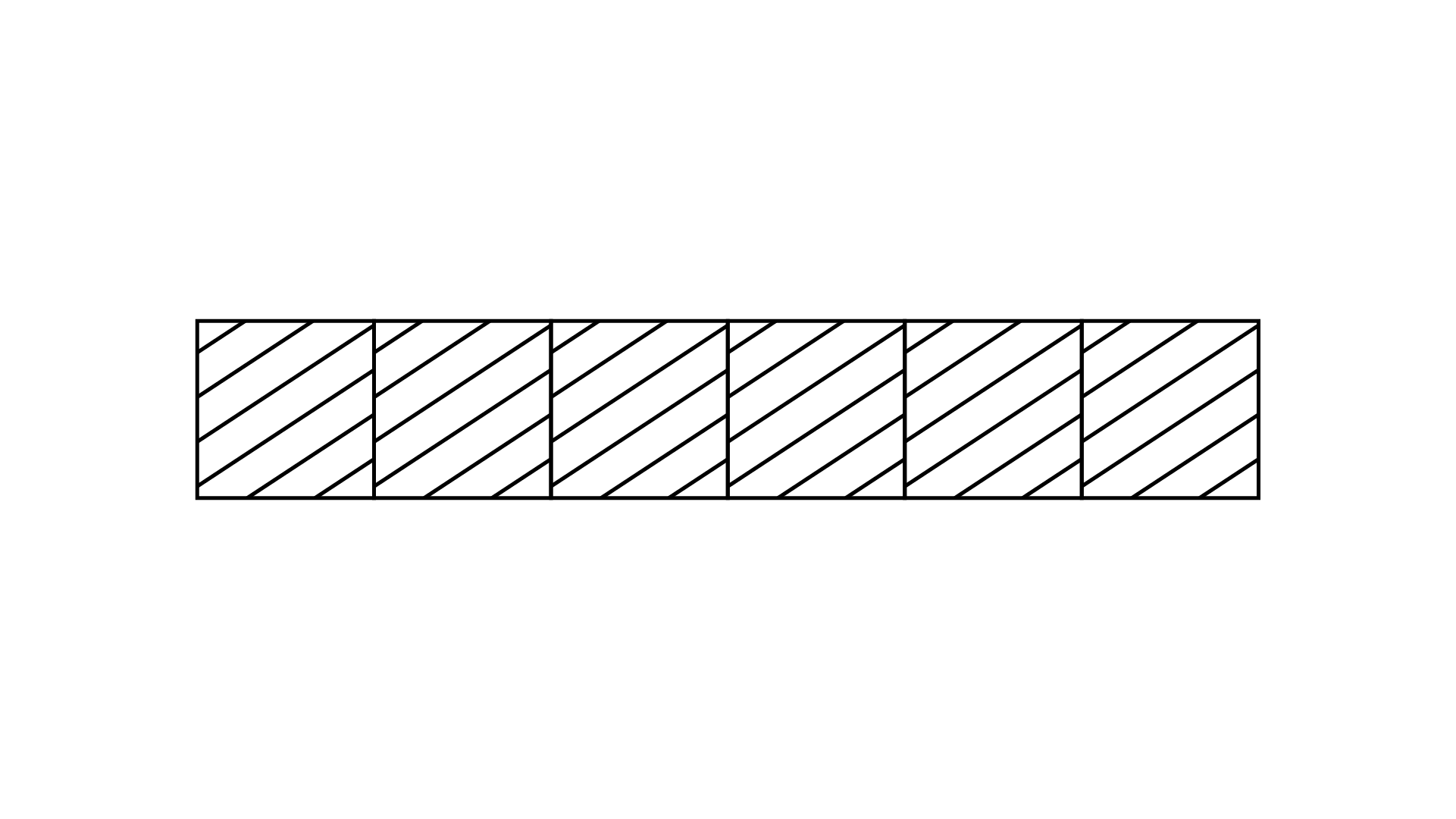
|
Ans:
Match the correct following
Column I | Column II |
(i) | (D) 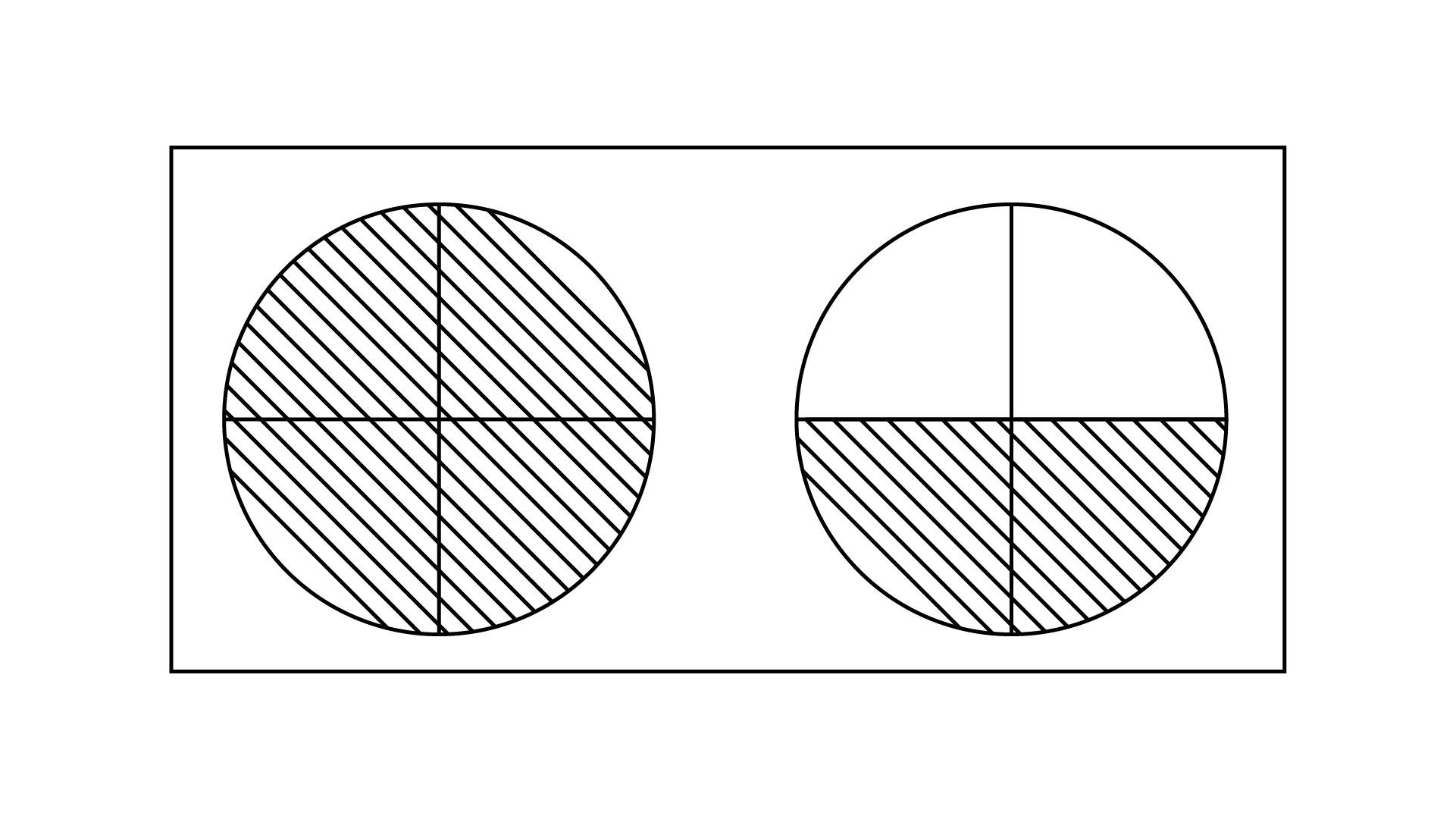
|
(ii) | (A) 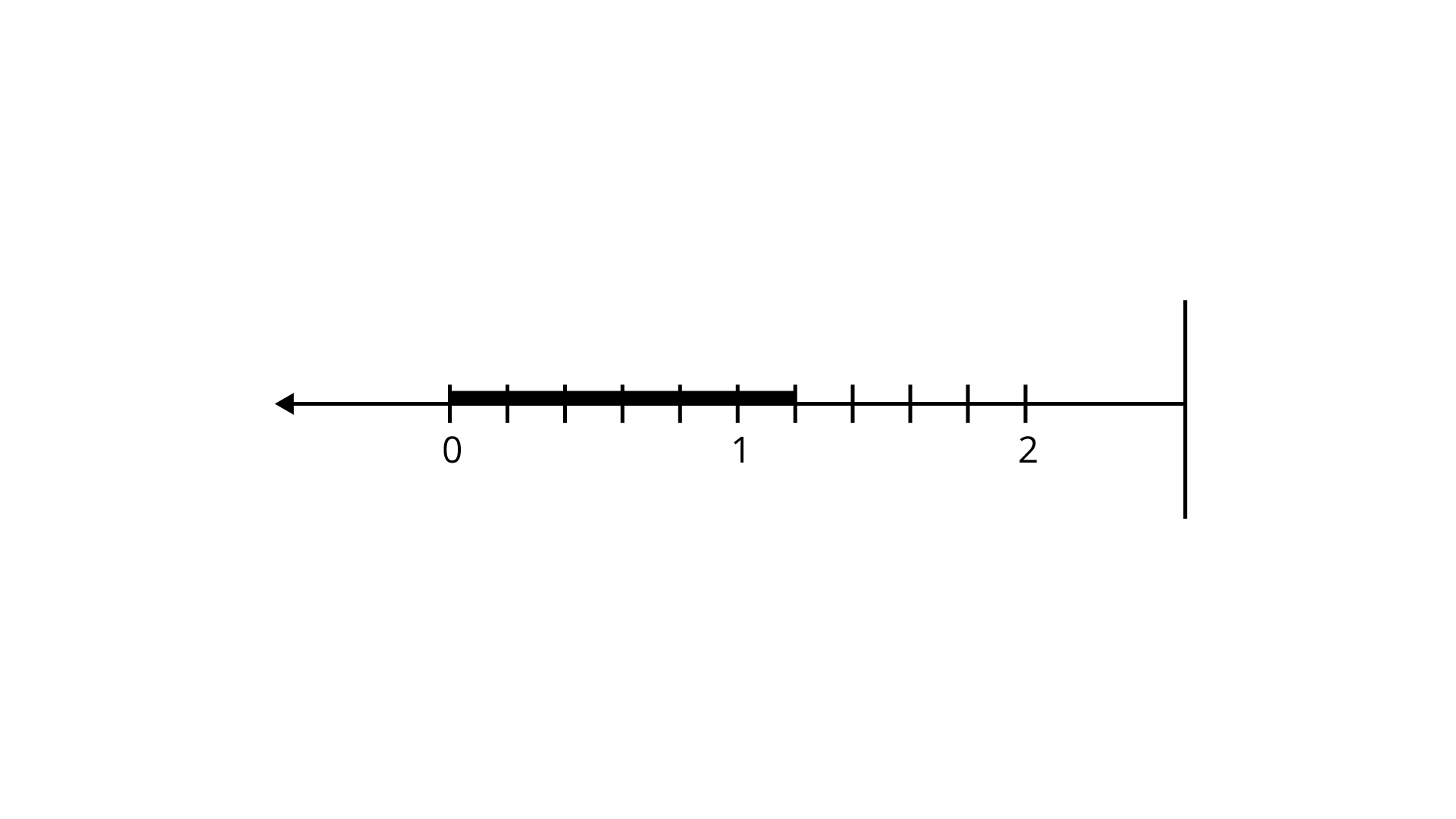
|
(iii) | (E) 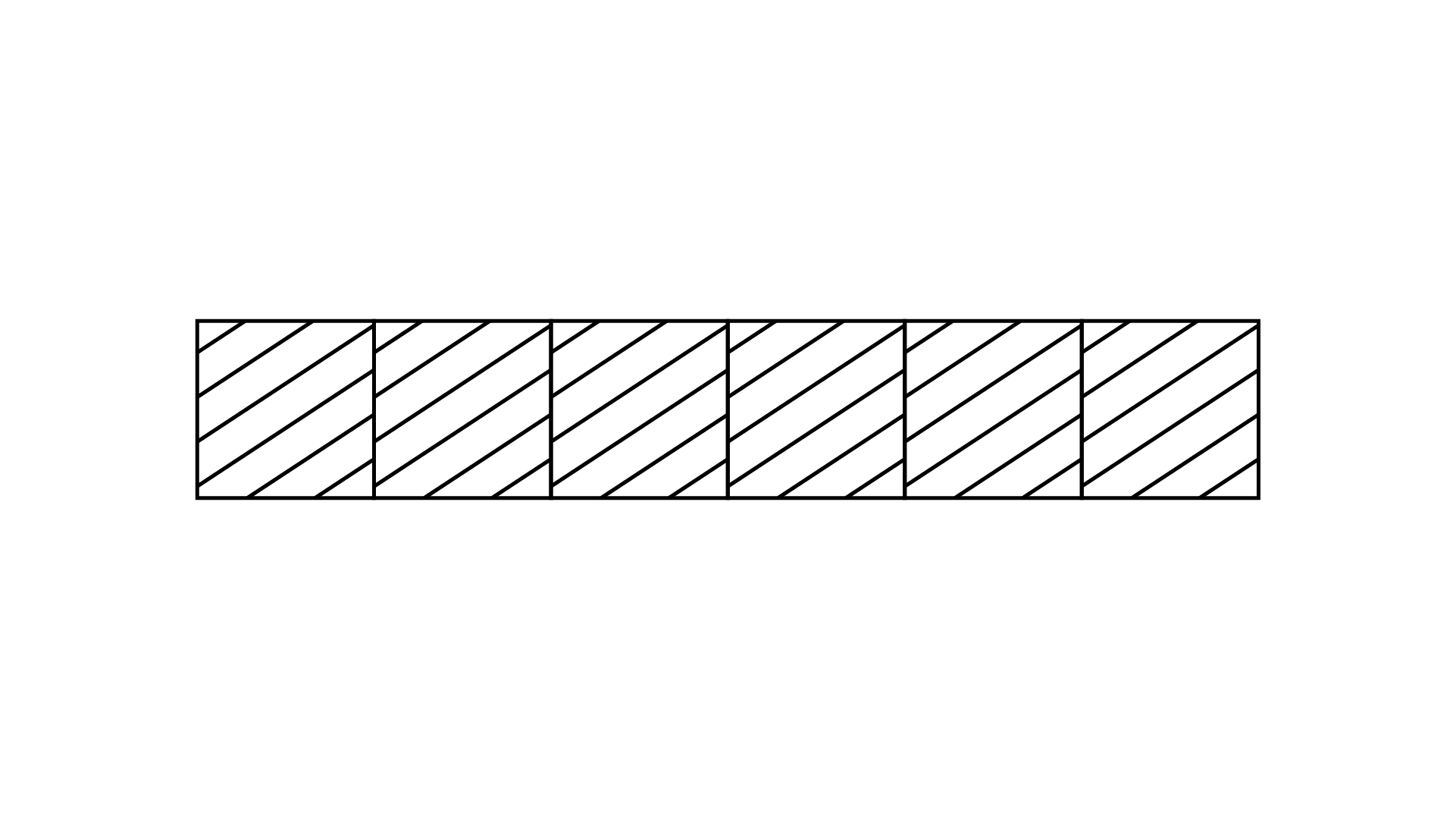
|
(iv) | (B) 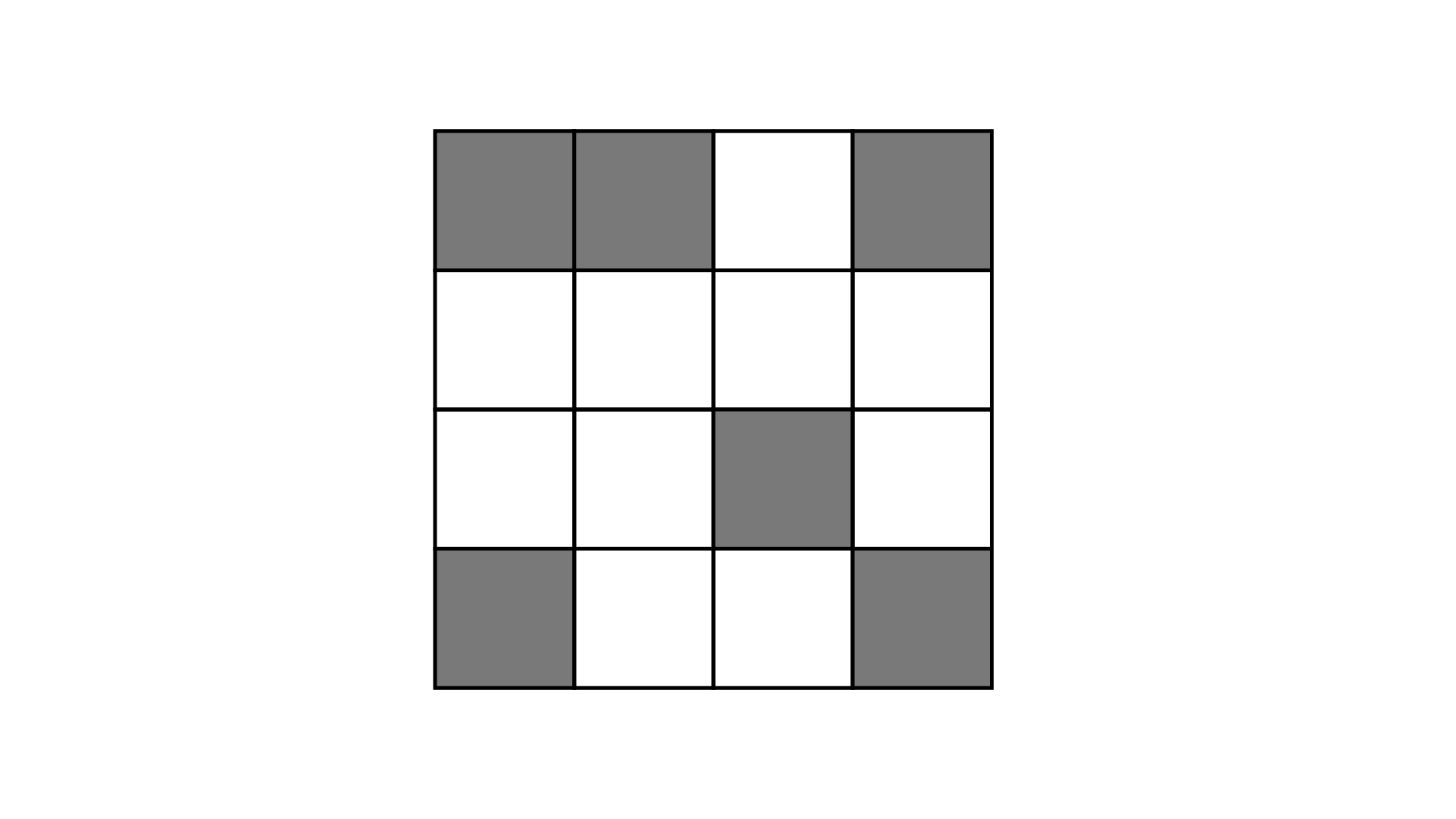
|
125. Find the fraction that represents the number of natural numbers to total numbers in the collection
Ans: From the of collection
The fraction that represents the number of natural numbers to the total numbers
The whole numbers are
The fraction that represents the number of whole numbers to the total numbers
126. Write the fraction representing the total number of natural numbers in the collection of numbers
Ans: Total collection of numbers
127. Write a pair of fractions whose sum is
Ans: Let one fraction be
Another fraction be
Thus, the fraction is
Pair of fractions are
128. What fraction of a straight angle is a right angle?
Ans: Since, we know that the measurement of a straight angle is
The fraction of straight angle is a right angle is
129. Put the right card in the right bag
Cards | Bags |
(i) (ii) (iii) (iv) (v) (vi) (vii) (viii) (ix) (x) | 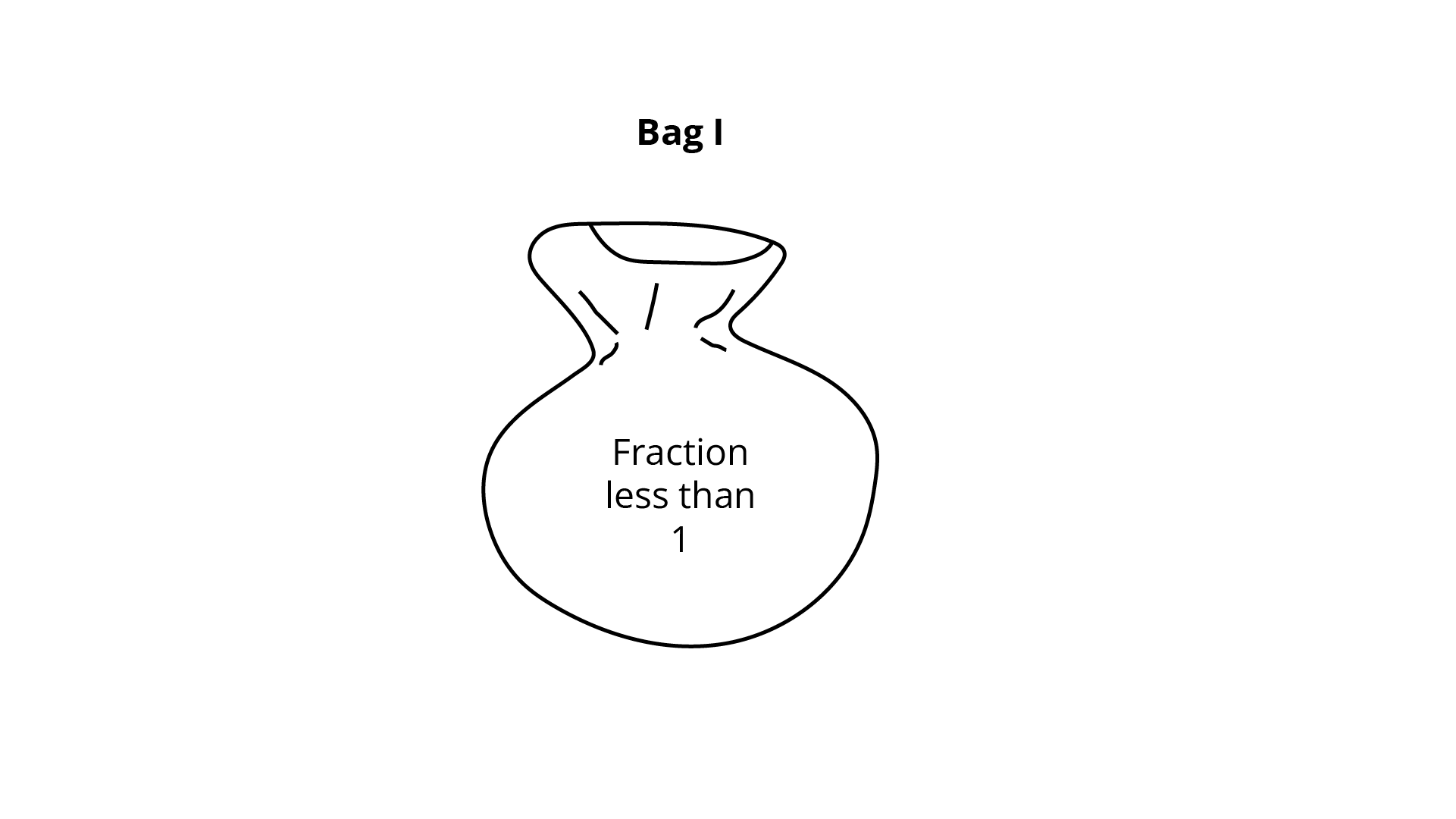

|
Ans:
fractions whose numerators are less than the denominators are called proper fractions. (Numerator < denominator) and fraction is less than one.
fractions with the numerator either equal to or greater than the denominator are called improper fractions. (Numerator = denominator or, Numerator > denominator) and the fraction is greater and equal than one.
Now, cards in Bag -I
(i)
(iv)
(v)
(vi)
(viii)
(ix)
Cards in Bag-II
(ii)
Cards in Bag -III
(iii)
What do we Learn in NCERT Exemplar Solutions for Class 6 Mathematics Chapter 4 Fractions and Decimals?
NCERT Exemplar solutions for Class 6 Mathematics Chapter 4 Fractions and Decimals consists of a fraction which is a number that represents a fraction of a total. This complete may be a single item or a set of items. Fractions with 10, 100 denominators, etc. can be written in the correct order, using a decimal point, called decimal or decimal numbers. Let us now consider some of the concepts discussed in this Chapter.
Multiplication and division of fractions and other concepts, such as types of fractions.
How to change different fractions to like fractions
How to compare more than two fractions.
How to turn a decimal into a fraction.
Converting a fraction into a decimal, adding, and subtracting decimal.
Decimal multiplication by 10, 100, 100, etc.
Decimal multiplication by a whole number, decimal multiplication by the decimal.
Key Topics for NCERT Exemplar Class 6th Mathematics Solutions Chapter 4: Components and Decimals
Introduction:
A fraction is a number that represents a fraction of a sum. A theme can be a single object or a set of objects. The parts are equal in quantity.
Proper Fraction:
In the right fraction, the number indicates the number of parts considered and the denominator indicates the number of parts in which the total is divided. Therefore, the denominator is always greater than the number in the correct fraction.
Improper Fraction:
Fractions where the number is greater than the denominator, are known as Improper fractions.
Mixed Fraction:
The component numbers that consist of both, part and total, are known as Mixed Fractions. The mixed part can be shown as the wrong part.
Equivalent Fraction:
Equivalent fractions represent the same part of the whole. To find the equal part of a given fraction, you can multiply or divide the number and the denominator of a given fraction by the same number.
Simplest Form of Fraction:
If there are no common features between a number and a denominator, the component should be the simplest.
Like and Unlike Fractions:
Like fractions with the same denominator while different fractions have different denominators. Fractions can be compared by turning them into parallel pieces and arranging them in an ascending or descending order.
Adding and Subtraction:
Adding and subtracting similar fractions is done by adding their numbers.
Adding and subtracting different fractions is done by translating them into the same fractions.
Decimals:
One-tenth of a block is divided into 10 parts and 100 parts can be written as 0.1 and 0.01 by the decimal point, where the dot is the decimal point and reaches the tenth place respectively.
All decimals can also be represented on a number line.
All decimals can be written as fractions.
When decimals are compared, every part is first compared, then tens and so on.
Addition and subtraction of decimals can be done in the same way as whole numbers by adding/subtracting one hundredth from one hundred and one-tenth out of ten, one in one and so on.
Decimals have many applications in our daily lives such as representing different units of measurement or representing parts of every business.
FAQs on NCERT Exemplar for Class 6 Maths Solutions Chapter 4 Fractions & Decimals
1. Why should I follow NCERT Exemplar solutions for Class 6 Mathematics Chapter 4 Fractions and Decimals?
NCERT Exemplar Solutions for Class 6 Mathematics Chapter 4 Fractions and Decimals PDF is available for download on the Vedantu website. Our professional teachers formulate these exemplar questions to help you prepare for the exams, to get good marks in Mathematics. Students who wish to get good marks in Mathematics actively practice NCERT Exemplar Solutions for Grade 6 Mathematics. This book is one of the best when it comes to giving a quiz bank to practice. Therefore, to acquire good marks a student should never ignore practicing Exemplar questions.
2. Why will a student choose Vedantu among all other websites for downloading Exemplar questions?
3. What are the important topics for NCERT Exemplar Class 6 Mathematics solutions Chapter 4 Fractions and Decimals?
Chapter 4 of the NCERT Exemplar contains a variety of tests such as multiple-choice questions, fill in the blanks, true or false and other very dependent questions.
Examples of NCERT exercise solutions are professionally prepared in such a way that these questions will be easily understood and will help you to practice firmly.
There are optional questions where you have to do addition, subtraction and multiplication of fractions and decimals.
For other questions, you must indicate whether the given pieces are right or wrong. You should also find the area value and the face value of the digits.
The NCERT Class 6 Mathematics Exemplar Solutions Chapter 4 prepared by Vedantu is a very important resource for your 6th-grade exams.
Vedantu provides you with simple language solutions and easy to understand Chapter concepts.
The solutions provided by Vedantu’s subject matter experts will also help you prepare for advanced ideas in the upper Classes.
These exemplary 6th-grade math solutions will provide you with a solid foundation for completing tests at an advanced level such as the NTSE exams.
Fractions can be compared by turning them into equal parts and arranging them in an ascending or descending order.
Addition (or subtraction) of the same fractions can be done by adding (or deleting) their numbers.
Fractions with 10,100 denominators, etc. can be written in the correct order, using a decimal point, called decimal or decimal numbers.
Decimal numbers can be compared using the concept of place value and can then be arranged in an ascending or descending order.
Decimals can be added (or removed) by writing them in equal numbers of decimal places.
Many problems of daily life can be solved by changing the units of various measurements such as amount, length, weight, etc. in the form of decimal and then adding (or subtracting).
















