Download the Important Questions for Class 12 Ray Optics And Optical Instruments PDF
Ray Optics and Optical Instruments, Chapter 9 of Class 12 Physics, delves into the behavior of light as rays and its interaction with various optical devices. This chapter's important questions include critical concepts like reflection, refraction, lens formula, and the working of optical instruments such as microscopes and telescopes and also these questions are also created by our Vedantu experts and master teachers.
Download the Class 12 Physics Syllabus for detailed coverage and explore our exclusive Class 12 Physics Important Questions PDF to solidify your understanding and crack your exams.



Access Class 12 Physics Chapter 9: Ray Optics And Optical Instruments Important Questions
1 Mark Questions
1. A person standing before a concave mirror cannot see his image unless he is beyond the centre of curvature. Why?
Ans: Let a man stand beyond focus i.e., between focus and centre of curvature, then the image formed will be real and inverted and is formed beyond C (beyond him). Thus, he cannot see the image.
But if he stands beyond C, the image will be formed between focus and centre of curvature which is in front of him and thus he will be able to see his reflected image.
2. For what angle of incidence, the lateral shift produced by a parallel-sided glass plate is maximum?
Ans: We know that lateral shift
For lateral shift
Then, we can say that lateral shift is maximum.
3. You read a newspaper, because of the light it reflects. Then why do you not see even a faint image of yourself in the newspaper?
Ans: We know that image is formed due to the regular reflection of light.
However, when we read a newspaper, there is diffused (irregular) reflection of light, thus we are not able to see even a faint image of ourselves on the newspaper.
4. A substance has a critical angle of
Ans: We know that the refractive index is given as below:
Substituting the values, we have
5. An object is placed between the pole and the focus of a concave mirror produces a virtual and enlarged image. Justify using mirror formula.
Ans: We know that the mirror formula is as given below,
Now magnification,
For a concave mirror,
Given
6. A converging and diverging lens of equal focal lengths are placed coaxially in contact. Find the focal length and power of the combination.
Ans: We know that the lens formula is as given below,
For converging lens
For diverging lens
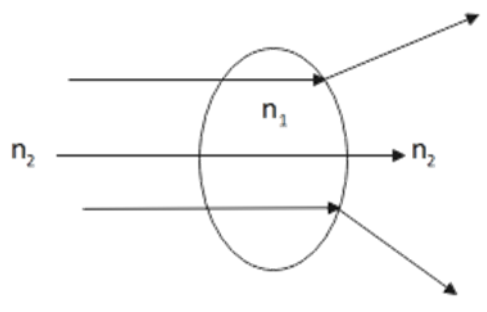
Since
Hence, the power of the combination of the lens is
7. The refractive index of a material of a convex lens is
Ans: We know that for
8. In a telescope the focal length of the objective and the eye piece are
Ans:
We know that magnification
The tube length is
9. Show the variation of
Ans: The variation of
10. Two lenses having focal length
Ans: The formula for the focal length of the combination of two lenses when placed coaxially at a distance
11. Does short-sightedness (myopia) or long-sightedness (hypermetropia) imply necessarily that the eye has partially lost its ability of accommodation? If not, what might cause these defects of vision?
Ans: Myopia and hypermetropia are common eye defects.
A myopic or hypermetropic person need not necessarily suffer a partial loss in their eyes’ ability of accommodation.
Myopia occurs when the eye-balls engage in elongation from the front to the back whereas hypermetropia occurs when the eye-balls shorten themselves.
On the other hand, when the eye-lens completely loses its ability of adjusting itself, then the defect is called presbyopia.
2 Mark Questions
1. What are optical fibres? Give their one use.
Ans: Optical fibres are thin and long strands of fine quality glass or quartz coated with a thin layer of material with refractive index less than that of the strands.
They work on the principle of total internal reflection and thus, they avoid any loss in transfer of information.
Uses
Optical fibres are often used in medical investigations i.e., one can examine the inside view of stomach and intestine by a method called endoscopy.
2. How do the focal lengths of a lens change with increase in the wavelength of the light?
Ans: We know that
Also,
Clearly, when wavelength
Similarly,
Clearly, as refractive index
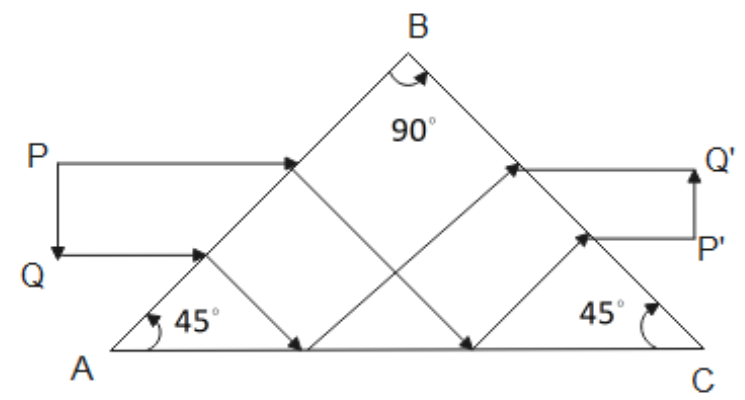
3. Show with a ray diagram, how an image is produced in a total reflecting prism?
Ans: Consider the two rays from the object PQ, as shown below.
They undergo total internal reflection firstly at the face AB and then at BC forming the final image
4. The radii of the curvature of the two spherical surfaces which is a lens of required focal length are not the same. It forms an image of an object. The surfaces of the lens facing the object and the image are interchanged. Will the position of the image change? Ans: As we know that,
When the radii of curvature
5. A thin converging lens has focal length (f) when illuminated by violet light. State with reason how the focal length of the lens will change if violet light is replaced by red light.
Ans: We know that,
Since,
6. Thin prism of angle
Substituting the given values, we have
7. Although the surfaces of a goggle lens are curved it does not have any power. Why? Ans: Since the two surfaces of a goggle lens are parallel i.e., one surface convex and the other concave, the resultant power of the two surfaces is zero as powers on both surfaces are equal but opposite in sign.
8. A ray of light is incident normally on one face of the prism of apex angle
Ans: Let us assume that the ray PQ falls normally on AB.
Then, it goes straight to AC without any refraction (QR) as shown in the figure.
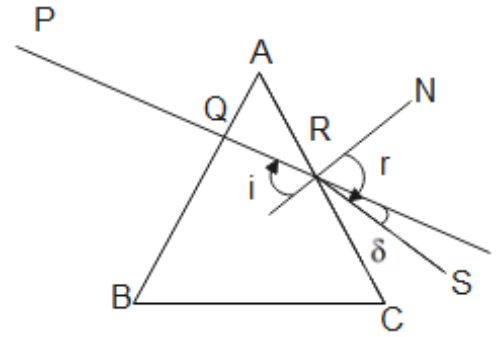
Also given that
Applying Snell’s law for face AC,
Now angle of deviation,
9. Following data was recorded for values of object distance and corresponding values of image distance in the experiment on study of real image formation by a convex lens of power
Sl.No: | 1 | 2 | 3 |
Object distance (cm): | 25 | 30 | 35 |
Image distance (cm): | 97 | 60 | 37 |
Ans: Given that
We know that
Then,
To find, which of the following observations are incorrect, consider the ray diagram as shown below, where it can be seen that when the object is placed between focus and twice the focus, the image is obtained after the measure if twice the focus.
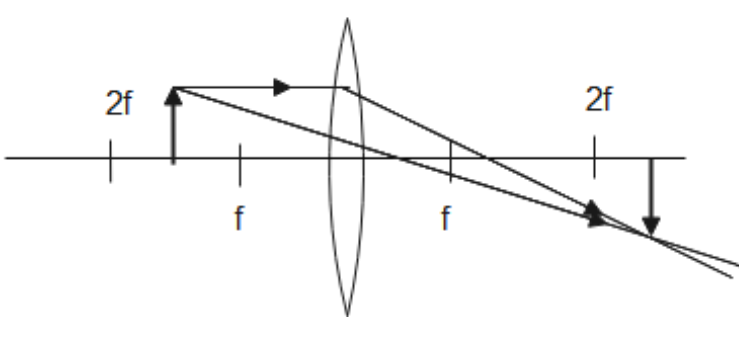
When the object is placed between
When the object is placed between
Here, when the object is placed between
Clearly, we can conclude that observation (3) is incorrect because both object and the image here lie between
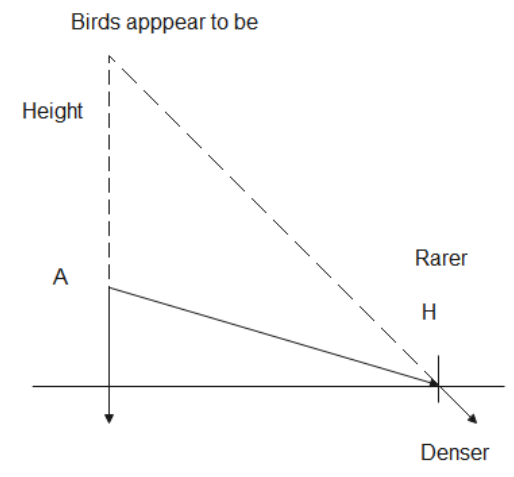
10. Birds flying high in the air appear to be higher than in reality. Explain why?
Ans: Birds fly in air, which is a rarer medium when compared to the ground, which is denser.
The light from the birds when viewed will undergo refraction towards the normal. Thus, the birds appear to fly at a higher point. i.e.,
Apparent height > Real height
11. What is the focal length of a convex lens of focal length
Ans: Given that,
Focal length of the convex lens,
Focal length of the concave lens,
Focal length of the system of lenses
Then the equivalent focal length of a system of two lenses in contact is given as:
Hence, the focal length of the combination of lenses is
12. The image of a small electric bulb fixed on the wall of a room is to be obtained on the opposite wall 3m away by means of a large convex lens. What is the maximum possible focal length of the lens required for the purpose?
Ans: Given that,
Distance between the object and the image,
Maximum focal length of the convex lens
For real images, the maximum focal length is given as:
Hence, for the required purpose, the maximum possible focal length of the convex lens is 0.75 m.
13. A screen is placed
Ans: Given that,
Distance between the image (screen) and the object,
Distance between two locations of the convex lens,
Focal length of the lens
Focal length is related to
Therefore, the focal length of the convex lens is
14. You are given prisms made of crown glass and flint glass with a wide variety of angles. Suggest a combination of prisms which will
Deviate a pencil of white light without much dispersion,
Ans: Place the two given prisms next to each other.
Make sure that their bases are on the opposite sides of the incident white light, with their faces touching each other.
When the white light is incident on the first prism, it will get dispersed. This acts as the incident light for the second prism and the dispersed light this time will recombine to give white light as a result of the combination of the two prisms.
Disperse (and displace) a pencil of white light without much deviation.
Ans: Take the system of the two prisms as suggested in answer (a).
Adjust (increase) the angle of the flint-glass-prism so that the deviations due to the combination of the prisms become equal.
This combination will now disperse the pencil of white light without much deviation.
15. A myopic person has been using spectacles of power
Ans: Given that the power of the spectacles used by the myopic person is
Focal length of the spectacles,
Hence, the far point of the person is
When he uses the spectacles, the objects placed at infinity produce virtual images at
He uses the ability of accommodation of the eye-lens to see the objects placed between
During old age, the person uses reading glasses of power,
The ability of accommodation is lost in old age. This defect is called presbyopia. As a result, he is unable to see clearly the objects placed at
16. A person looking at a person wearing a shirt with a pattern comprising vertical and horizontal lines is able to see the vertical lines more distinctly than the horizontal ones. What is this defect due to? How is such a defect of vision corrected?
Ans: In the given case, the person is able to see vertical lines more distinctly than horizontal lines.
This means that the refracting system (cornea and eye-lens) of the eye is not working effectively in different planes. This defect is called astigmatism.
The person's eye has enough curvature in the vertical plane. However, the curvature in the horizontal plane is insufficient.
Hence, sharp images of the vertical lines are formed on the retina, but horizontal lines appear blurred. This defect can be corrected by using cylindrical lenses.
17. A small telescope has an objective lens of focal length
The telescope is in normal adjustment (i.e., when the final image is at infinity)?
Ans: Given that,
Focal length of the objective lens,
Focal length of the eyepiece,
Least distance of distinct vision,
When the telescope is in normal adjustment, its magnifying power is given as:
Thus, the magnifying power is
The final image is formed at the least distance of distinct vision 25cm?
Ans: When the final image is formed at
given as:
Thus, the magnifying power is
18. Light incident normally on a plane mirror attached to a galvanometer coil retraces backwards as shown in figure below. A current in the coil produces a deflection of
Ans: Given that,
Angle of deflection,
Distance of the screen from the mirror,
The reflected rays get deflected by an amount twice the angle of deflection i.e.,
The displacement (
Hence, the displacement of the reflected spot of light is
3 Marks Questions
1. Find the radius of curvature of the convex surface of a plane convex lens, whose focal length is
Ans: Given that,
For plane convex lens,
Substituting these values in the formula for focal length,
Thus, the radius of curvature is
2. Show that the limiting value of the angle of prism is twice its critical angle. Hence define critical angle.
Ans: We know that,
Angle of the prism is given by
In a case of a triangular prism where
where,
Clearly,
Therefore, the angle of incidence for which angle of refraction is
3. Draw a labelled diagram of telescope when the image is formed at the least distance of distinct vision? Hence derive the expression for its magnifying power.
Ans: We know that,
magnifying power

For eye piece,
Multiply by D,
Substituting in (i),
4. Drive the expression for the angle of deviation for a ray of light passing through an equilateral prism of refracting angle A.
Ans: Consider the given diagram:
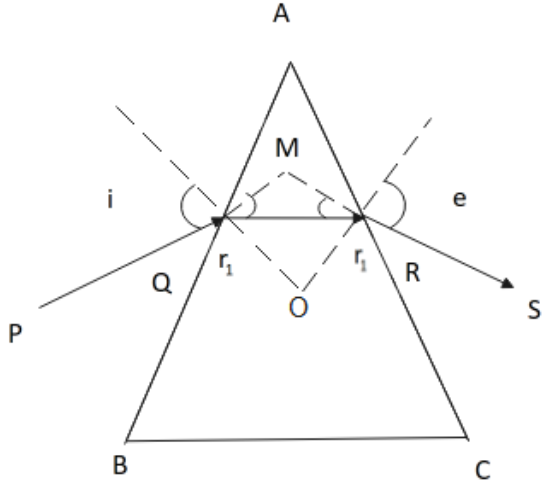
At the surface AB,
At the surface AC,
Thus,
Now, in quadrilateral AQOR,
Also, in
Or
From (2) and (3),
Substituting equation (4) in equation (1)
Or
5. Draw a ray diagram to illustrate image formation by a Newtonian type reflecting telescope. Hence state two advantages of it over refracting type telescopes.
Ans: The ray diagram of the Newtonian type reflecting telescope is as shown below.
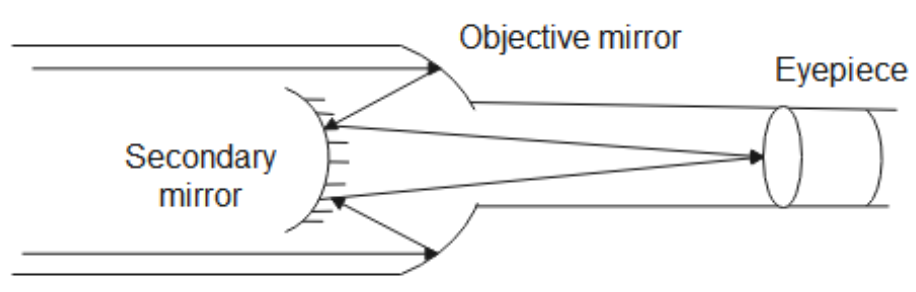
Advantages
The image formed in a reflecting type telescope is free from chromatic aberrations.
The image formed is very bright due to its large light-gathering power.
6. The magnifying power of an astronomical telescope in the normal adjustment position is
Ans: Given that,
Substituting equation (2) in equation (1),
Substituting
Thus, the focal length of the eye-piece is 1cm whereas the focal length of the objective is 100cm.
7. A convex lens made up of refractive index
Ans: When
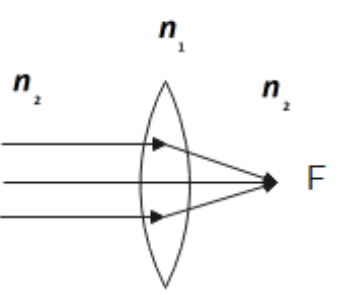
Ans: When
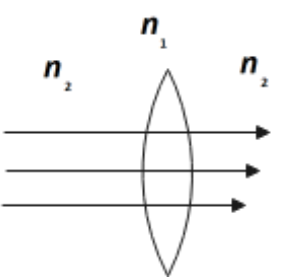
Ans: When
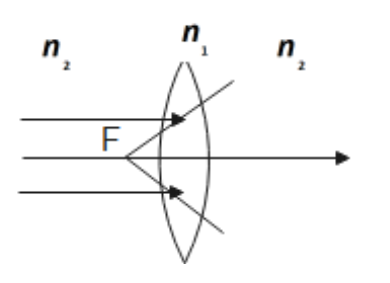
8. Derive the relation
Ans: Consider two thin lenses in contact having focal length
For the first lens,
For the second lens,
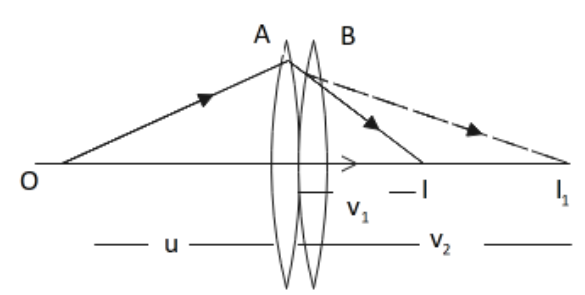
Clearly,
Adding equations (1) & (2)
Using lens formula
For
Hence the derivation.
9. A convex lens has a focal length
Ans: Given,
It is known that
Now,
Also, the focal length of the lens when immersed in water would be
Thus, the change in focal length is given by
10. A reflecting type telescope has a concave reflector of radius of curvature
Ans: Given that
Thus, the focal length of the eye-piece is 3cm.
11. Show that a convex lens produces an N time magnified image, when the object distances from the lens have magnitude
Ans: We know that the magnifying power is given by
For real image
For virtual image
From equation (1) & (2) we can say that magnification produced by a lens can be N if
Now, power of the lens
It is given that
These are the two values of object distances.
12. Define total internal reflection of light. Hence write two advantages of total reflecting prisms over a plane mirror.
Ans: The phenomenon of reflection of light when a ray of light traveling from a denser medium getting reflected back into the same denser medium provided the angle of incidence is greater than the angle called critical angle is called total internal reflection.
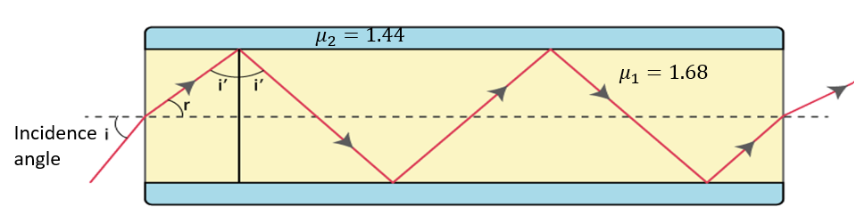
Advantages
It does require silvering.
Multiple reflections do not take place in a reflecting prism. Due to this, only one image is formed, which is very bright.
13. An equiconvex lens of radius of curvature R is cut into two equal parts by a vertical plane, so it becomes a plano-convex lens. If f is the focal length of equiconvex lens, then what will be focal length of the planoconvex lens?
Ans: We know that
For equiconvex lens
For plano-convex lens,
Clearly,
From (1) & (2)
14. A converging lens of focal length
Distance of Object From the Lens.
Ans: It is known that,
Given,
Substituting these values in the first expression,
Angular Magnification
Ans: Angular magnification is given by
Angular Magnification When Final Image is Formed at Infinity.
Ans: When the image is formed at infinity,
15. Draw a graph to show that variation of angle of deviation
Ans: For the minimum deviation position,
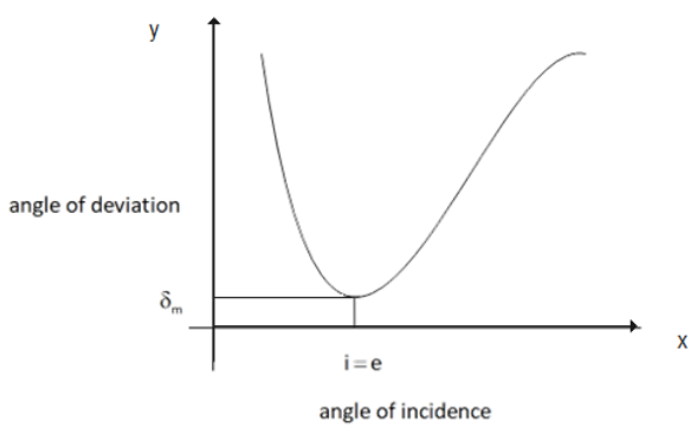
Also,
Now,
We know that:
Also,
Applying minimum deviation condition is equation (1);
Applying Snell’s law,
16. An object of size
Ans: Given that,
Size of the object,
Object distance,
Focal length of the concave lens,
Let image distance
According to the lens formula, we have the relation:
Substituting the given values,
Hence, the image is formed on the other side of the lens, 8.4 cm away from it. The negative sign shows that the image is erect and virtual.
The magnification of the image is given as:
Hence, the height of the image is
If the object is moved further away from the lens, then the virtual image will move toward the focus of the lens, but not beyond it. Also, the size of the image will decrease with the increase in the object distance.
17. A beam of light converges at a point P. Now a lens is placed in the path of the convergent beam
A convex lens of focal length
Ans: In the given situation, the object is virtual and the image formed is real.
Object distance,
Focal length of the convex lens,
Image distance
According to the lens formula, we have the relation:
Hence, the image is formed
A concave lens of focal length
Ans: Focal length of the concave lens,
Image distance
Object distance,
According to the lens formula, we have the relation:
Hence, the image is formed
18. Double-convex lenses are to be manufactured from a glass of refractive index 1.55, with both faces of the same radius of curvature. What is the radius of curvature required if the focal length is to be
Ans: Given that,
Refractive index of glass,
Focal length of the double-convex lens,
Radius of curvature of one face of the lens
Radius of curvature of the other face of the lens
Let radius of curvature of the double-convex lens
The value of R can be calculated as:
Hence, the radius of curvature of the double-convex lens is
19. A small telescope has an objective lens of focal length
Ans: Given,
Focal length of the objective lens,
Focal length of the eyepiece,
The magnifying power of the telescope is given as:
The separation between the objective lens and the eyepiece is calculated as:
Hence, the magnifying power of the telescope is
20.
A giant refracting telescope at an observatory has an objective lens of focal length 15 m. If an eyepiece of focal length 1.0 cm is used, what is the angular magnification of the telescope?
Ans: Given that,
Focal length of the objective lens,
Focal length of the eyepiece,
The angular magnification of a telescope is given as:
Hence, the angular magnification of the given refracting telescope is 1500.
If this telescope is used to view the moon, what is the diameter of the image of the moon formed by the objective lens? The diameter of the moon is
Ans: Given that,
Diameter of the moon,
Radius of the lunar orbit,
Let
The angle subtended by the diameter of the moon is equal to the angle subtended by the image.
Hence, the diameter of the moon's image formed by the objective lens is
21. A small pin fixed on a table top is viewed from above from a distance of 50 cm. By what distance would the pin appear to be raised if it is viewed from the same point through a 15 cm thick glass slab held parallel to the table? Refractive index of glass
Ans: Given that, actual depth of the pin,
Refractive index of glass,
Let the apparent depth of the pin
Ratio of actual depth to the apparent depth is equal to the refractive index of glass, i.e.,
Now, the distance by which the pin appears to be raised
For a small angle of incidence, this distance does not depend upon the location of the slab.
22. A man with normal near point (25 cm) reads a book with small print using a magnifying glass: a thin convex lens of focal length 5 cm.
What is the closest and the farthest distance at which he should keep the lens from the page so that he can read the book when viewing through the magnifying glass?
Ans: Given that,
Focal length of the magnifying glass,
Least distance of distance vision,
Let the object distance
Image distance,
According to the lens formula, we have:
Hence, the closest distance at which the person can read the book is
For the object at the farthest possible distance, the image distance
According to the lens formula, we have:
Hence, the farthest distance at which the person can read the book is
What is the maximum and the minimum angular magnification (magnifying power) possible using the above simple microscope?
Ans: Maximum angular magnification is given by the relation:
Minimum angular magnification is given by the relation:
23. A card sheet divided into squares each of size
What is the magnification produced by the lens? How much is the area of each square in the virtual image?
Ans: Given that,
Size of each square,
Object distance,
Focal length of a converging lens,
For image distance
Magnification,
What is the angular magnification (magnifying power) of the lens?
Ans: Magnifying power of the lens
Is the magnification in (a) equal to the magnifying power in (b)? Explain.
Ans: The magnification in (a) is not the same as the magnifying power in (b).
The magnification magnitude is
The two quantities will be equal only when the image is formed at the near point.
24.
At what distance should the lens be held from the figure in Exercise 9.29 in order to view the squares distinctly with the maximum possible magnifying power?
Ans: The maximum possible magnification is obtained when the image is formed at the near point
Here,
Image distance,
Focal length,
Object distance
According to the lens formula, we have:
Hence, to view the squares distinctly, the lens should be kept
What is the magnification in this case?
Ans: Magnification
Is the magnification equal to the magnifying power in this case? Explain.
Ans: Here,
Magnifying power
Thus, it can be concluded that since the image is formed at the near point (25 cm), the magnifying power is equal to the magnitude of magnification.
25. What should be the distance between the object in Exercise 9.30 and the magnifying glass if the virtual image of each square in the figure is to have an area of
Ans: Given,
Area of the virtual image of each square,
Area of each square,
The linear magnification of the object can be calculated as:
However,
Now,
Focal length of the magnifying glass,
According to the lens formula, we have the relation:
and
The virtual image is formed at a distance of
26.
For the telescope described in Exercise 9.34 (a), what is the separation between the objective lens and the eyepiece?
Ans: Given,
Focal length of the objective lens,
Focal length of the eyepiece,
In normal adjustment, the separation between the objective lens and the eyepiece is the sum of focal length of the objective lens and that of the eye piece.
If this telescope is used to vie a 100m tall tower 3km away, what is the height of the image of the tower by the objective lens?
Ans: Given,
Height of the tower,
Distance of the tower (object) from the telescope,
The angle subtended by the tower at the telescope is given as:
The angle subtended by the image produced by the objective lens is given as:
Where,
Therefore, the objective lens forms a
What is the height of the final image of the tower if it is formed at 25cm?
Ans: Given, the image is formed at a distance,
The magnification of the eyepiece is given by the relation:
Height of the final image
Therefore, the height of the final image of the tower is
5 Mark Questions
1. Prove that
When refraction occurs of a convex spherical refracting surface and the ray travels from rarer to denser medium.
Ans: The ray is travelling from a rarer medium to a denser medium as shown in the figure.
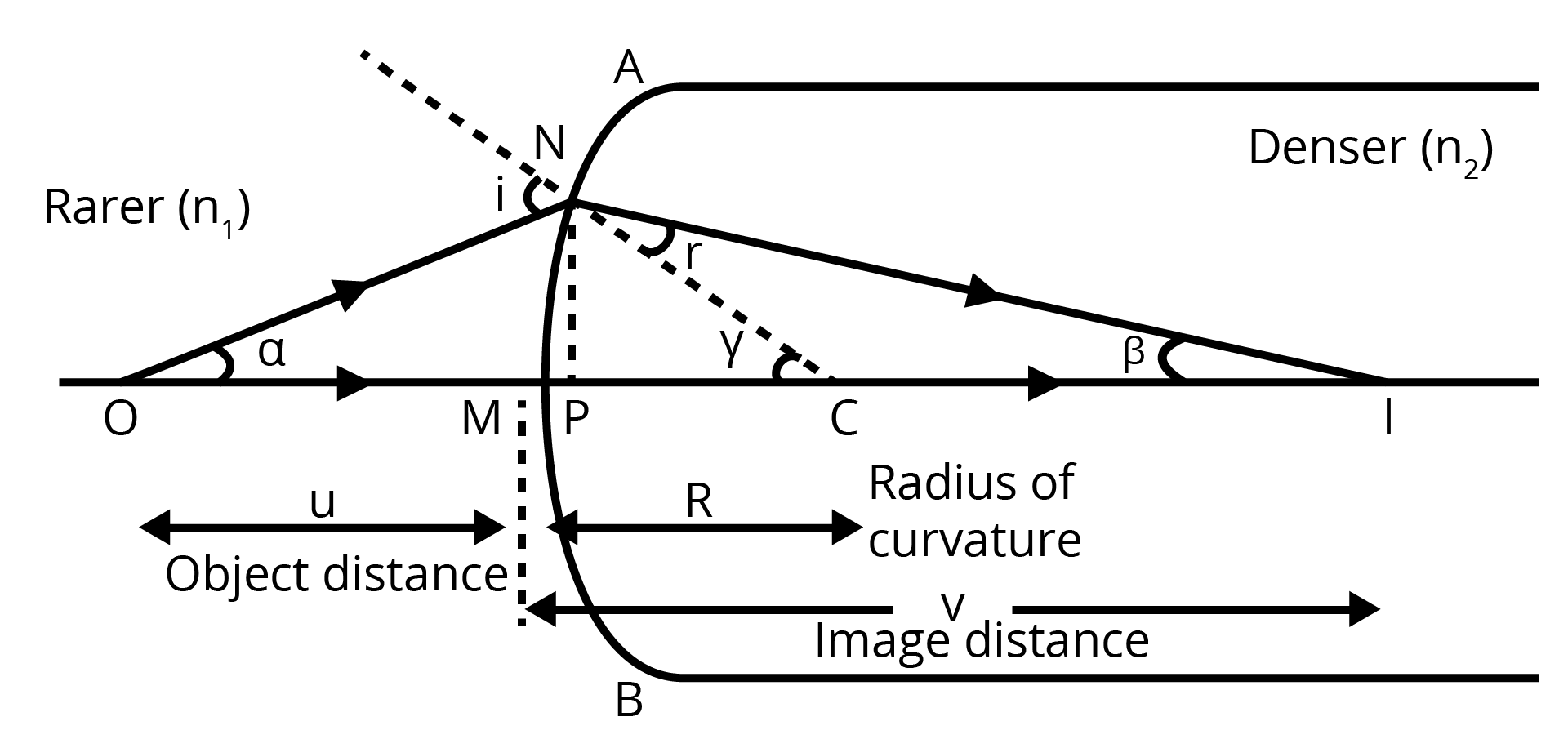
Now, we have
We get,
From figure,
For small angle,
Now equation (1) becomes,
Similarly,
Using Snell’s Law,
For small angle,
Now, we put the value of i and r in the above equation,
Put
Hence, Proved.
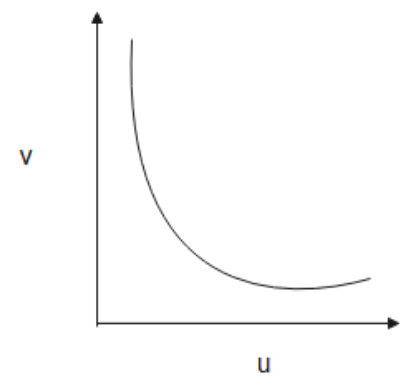
2. A lens forms a real image of an object. The distance of the object. From the lens is u cm and the distance of the image from the lens is v cm. The given graph shows the variation of v and u
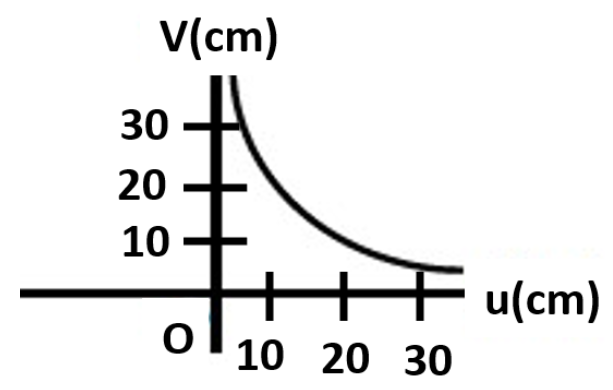
What is the nature of the lens?
Ans: The lens is a convex lens.
Using the graph find the focal length of the lens?
Ans: We use lens formula,
When
Draw a ray diagram to show the formation of image of same size as that of object in case of converging lens hence derive lens equation?
Ans: The below figure shows the formation of same size of image as that of object.
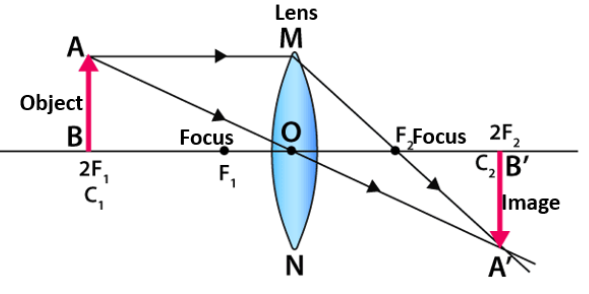
From above figure,
And, we know,
From (1) & (2),
Put
Divide by
This is derived.
3. By stating sign conventions and assumptions used derive the relation between
Ans: Sign Conventions:
All distances are estimated from the mirror's pole.
Distance measured in the incident light direction is positive, and those measured in the direction opposite to the incident light are negative.
Height estimated upwards is positive and height estimated downwards is negative.
Assumptions:
Spherical mirror's aperture is supposed to be very small.
The incident and refracted rays form small angles with the principal axis.
This figure shows an image produced by the concave mirror.

This gives,
Also,
Combining (1) and (2),
We are considering that E is close to P, so,
From figure,
Now,
Put
Dividing by
Hence, derived.
4.
A person looking at a mesh of crossed wires is able to see the vertical lines more distinctly than the horizontal wires. What is the effect due to? How is such a defect of vision corrected?
Ans: In the given case, the person can see vertical lines more precisely than horizontal lines.
This means that the eye's refracting system (cornea and eye-lens) is not working similarly in different planes. This defect is named astigmatism.
The person's eye has sufficient curvature in the vertical plane. However, the horizontal plane's curvature is insufficient. Hence, sharp vertical lines are made on the retina, but horizontal lines seem blurred.
This defect can be fixed by using cylindrical lenses.
A man with normal near point (
What is the closest and the farthest distance at which he can read the book when viewing through the magnifying glass?
Ans: Given that,
Focal length of the magnifying glass,
Least distance of distance vision,
Closest Object distance
Image distance,
Using lens formula,
Hence, the nearest distance at which the person can read the book is
For the object at the farthest distance
Using lens formula,
Hence, the farthest distance at which the person can read the book is
What is the maximum and minimum angular magnificent (magnifying power) possible using the above simple microscope?
Ans: The formula for maximum angular magnification is given by:
The formula for minimum angular magnification is given by:
Thus, the maximum and the minimum angular magnification (magnifying power) possible using the given simple microscope are
5. Four double convex lens with following specifications are available?
Lens Focal Length | Aperture | |
A | 100 cm | 10 cm |
B | 100 cm | 5 cm |
C | 10 cm | 2 cm |
D | 5 cm | 2 cm |
Which of the given four lenses should be selected as objective and eyepiece to construct an astronomical telescope and why? What will be the magnifying power and length of the tube of this telescope?
Ans: The telescope’s objective should be of large aperture so that it can receive maximum light and should be of large focal length so that it can produce maximum magnification.
From table, we choose lens A as objective and lens D as eyepiece due to small aperture and small focal length.
Magnification,
Normal length,
The magnification is
An object is seen with the help of a simple microscope, firstly in red light and then is blue light. Will the magnification be same in both the cases? Why?
Ans: The focal length of lens is small for violet light than red light and magnifying power is inversely dependent on the focal length. So, magnification for violet light will be larger.
6. A small candle,
Ans: We have,
Size of the candle,
Let image size
Object distance,
concave mirror’s curvature radius,
Focal length of the concave mirror,
Using mirror formula,
Where, u is the object distance,
v is the image distance and
f is the focal length.
Now, we put given values,
Therefore, the screen should be 54 cm away from the mirror to get a sharp image.
The formula for magnification of image is given by:
The height of the image of the candle is
If the candle is moved nearer to the mirror, then the screen will have to be moved far from the mirror in order to get the image.
7. A
Ans: Given that,
Height of the needle,
Object distance,
Focal length of the convex mirror,
Image distance
Using mirror formula,
Now, we put given values,
Hence, the needle’s image is
The formula for magnification of image is given by:
Hence, magnification,
The image’s height is 2.5cm. The positive sign shows that the image is virtual, erect, and diminished.
If the needle is moved away from the mirror, the image will also move farther from the mirror, and the size of the image will decrease gradually.
8. A tank is filled with water to a height of
Ans: Given that,
Actual depth of the needle in water,
Apparent depth of the needle in water,
Refractive index of water
The formula for refractive index is given by:
Put the given values,
Hence, the water’s refractive index is about
When water is replaced by a liquid of refractive index,
The actual depth of the needle will be the same, but it's apparent depth will vary.
Let
We will use the relation given below:
We get the new apparent depth of the needle to be
The distance by which the microscope would be moved up
9. Figures (a) and (b) show refraction of a ray in air incident at
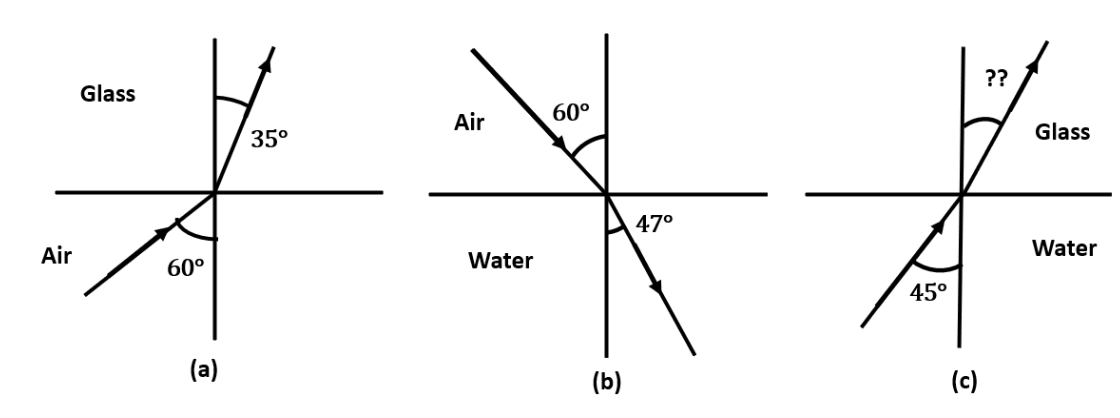
Ans: Given that,
For the glass -air interface,
Angle of incidence,
Angle of refraction,
We can use Snell’s law,
For the air-water interface,
Angle of incidence,
Angle of refraction,
We can use Snell’s law,
Using (i) and (ii), the relative refractive index of glass with respect to water can be derived as:
For the glass - water interface,
Angle of incidence,
Angle of refraction,
We can use Snell’s law,
Hence, the angle of refraction at the water – glass interface is
10. A small bulb is placed at the bottom of a tank containing water to a depth of
Ans: Provided that,
Bulb’s actual depth in water,
Water’s refractive index,
The following diagram represents the given setup:
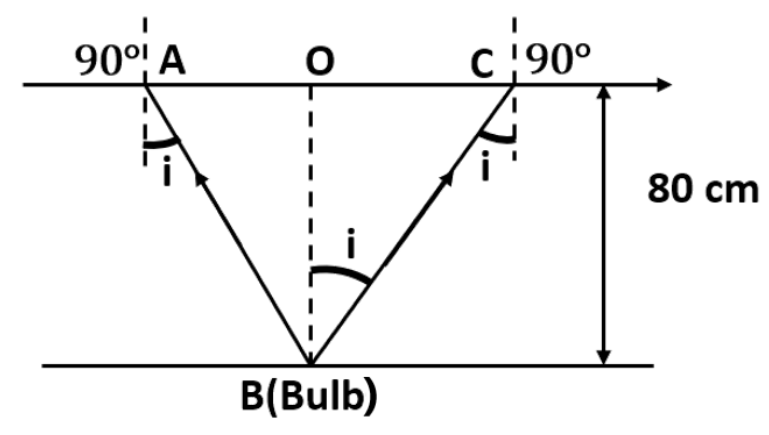
Where, i is the Angle of incidence
r is the Angle of refraction,
As the bulb acts as a point source, the emergent light would be considered as a circle of radius,
Snell’s law may be used as follows:
Considering the given diagram, we have the relation:
Area of the surface of water
Clearly, the area of the water surface through which the light from the bulb could project is about
11. A prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. The angle of minimum deviation is measured to be
Ans: The minimum deviation angle and the angle of prism is as shown in figure given below:
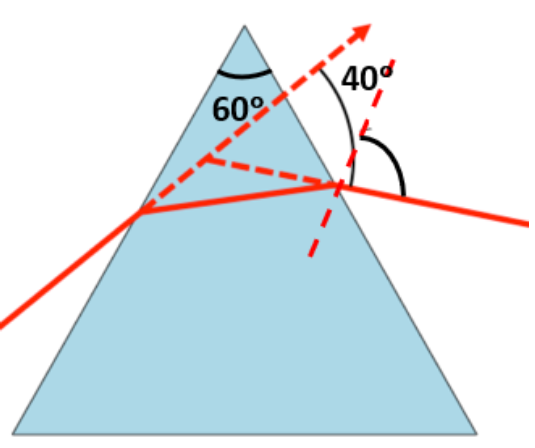
Angle of minimum deviation,
Angle of the prism,
Refractive index of water,
Refractive index of the material of the prism
The relation between angle of deviation with refractive index is given by:
Hence, the refractive index of the prism is
Since the prism is placed in water, let
The below figure shows the angle of prism and the unknown minimum deviation angle.

The refractive index of glass with respect to water is given by the relation:
Hence, the new minimum angle of deviation is
12. A compound microscope consists of an objective lens of focal length
The least distance of distinct vision (
Ans: Given that,
Focal length of the objective lens,
Focal length of the eyepiece,
Distance between the objective lens and the eyepiece,
Least distance of distinct vision,
lmage distance for the eyepiece,
Object distance for the eyepiece
Using lens formula,
The distance of image for the objective lens,
The distance of object for the objective lens
Using lens formula,
Magnitude of the object distance,
The compound microscope’s magnifying power is given by the relation:
Hence, the magnifying power of the microscope is
At infinity? What is the magnifying power of the microscope?
Ans: Given that, the final image is formed at infinity.
The distance of image of the eyepiece,
The distance of object of the eyepiece
Using lens formula,
The distance of image for the objective lens,
The distance of object for the objective lens
Using lens formula,
Magnitude of the object distance,
The compound microscope’s magnifying power is given by the relation:
Hence, the magnifying power of the microscope is
13. A person with a normal near point (
Ans: Given that,
Focal length of the objective lens,
Focal length of the eyepiece,
The distance of the object for the Objective lens,
Least distance of distant vision,
Image distance for the eyepiece,
Object distance for the eyepiece,
Using lens formula,
Using lens formula, we can obtain image distance for the objective lens,
The distance between the objective lens and the eyepiece,
The microscope’s magnifying power is given by the relation:
Hence, the magnifying power of the microscope is
14. Use the mirror equation to deduce that:
an object placed between
Ans: For a concave mirror,
When the object is placed on the left side of the mirror, then
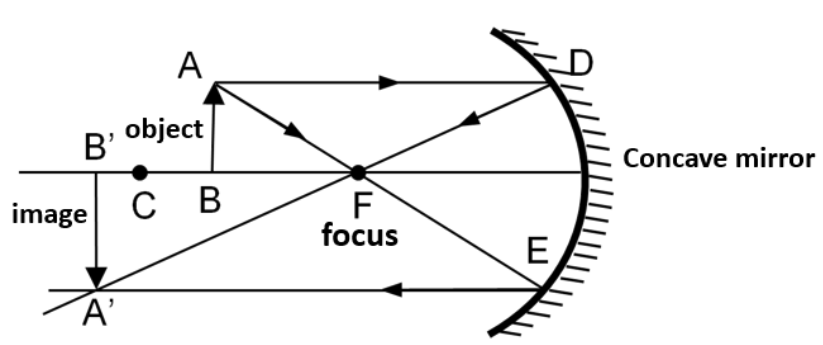
Using mirror formula,
If the object placed between
Using equation (1), we get
Since
Therefore, image will lie beyond
a convex mirror always produces a virtual image independent of the location of the object.
Ans: For a convex mirror,
When the object is placed on the left side of the mirror, then
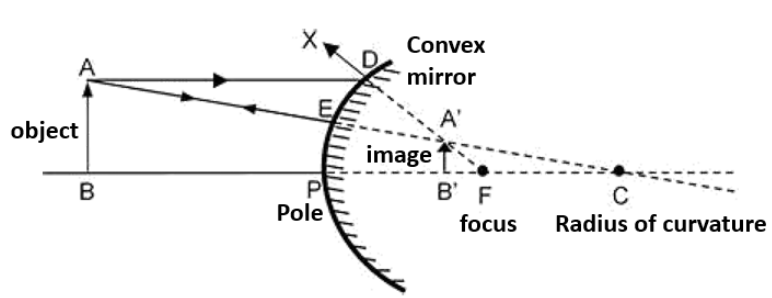
Using mirror formula,
Using equation (2), we get:
Thus, the image is formed on the mirror’s back side.
Hence, a convex mirror always gives a virtual image, regardless of the object distance.
The virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.
Ans: For a convex mirror,
Using mirror formula,
But we have,
Hence, the image formed is diminished and is located between the focus and the pole.
An object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.
Ans: For a concave mirror,
When the object is placed on the left side of the mirror, then
The object is placed between the focus and the pole.
The image is formed on the mirror’s right side. Hence, it is a virtual image.
For
Magnification,
Hence, the formed image is enlarged.
15.
Figure shows a cross-section of a 'light pipe' made of a glass fibre of refractive index

Ans: Given that,
Refractive index of the glass fibre,
Refractive index of the outer covering of the pipe,
Angle of incidence
Angle Of refraction
Angle of incidence at the interface
The refractive index (
For the critical angle, total internal reflection (TIR) takes place only when
That is, when
Maximum angle of reflection,
Let
The refractive index at the air – glass interface,
We can use the relation for the maximum angles of incidence and reflection as:
Thus, all the ray’s incident at angles lying in the range
What is the answer if there is no outer covering of the pipe?
Ans: If the outer covering of the pipe is not present, then:
Refractive index of the outer pipe
Refractive index of air
For the angle of incidence
Since,
16. Answer the following questions:
You have learnt that plane and convex mirrors produce virtual images of objects. Can they produce real images under some circumstances? Explain.
Ans: Yes. Plane and convex mirrors can provide real images as well.
If the light rays converging at a point behind a plane mirror (or a convex mirror) are reflected on a screen placed in front of the mirror, then an actual image will be formed.
A Virtual image, we always say, cannot be caught on a screen. Yet when we ‘see' a virtual image, we are obviously bringing it on to the ‘screen' (i.e., the retina) of our eye. Is there a contradiction?
Ans: No. A virtual image is made when light rays diverge.
The convex lens of the eye makes these divergent rays converge at the retina. The virtual image works as an object for the lens to provide an actual image in this situation.
A diver underwater, looks obliquely at a fisherman standing on the bank of a lake. Would the fisherman look taller or shorter to the diver than what he actually is?
Ans: The diver is in the water, and the fisherman is on the land. Water is a denser medium than air.
It is provided that the diver is viewing the fisherman. This shows that the light rays are traveling from a denser medium to a rarer medium.
Hence, the refracted rays will go away from the normal. As a result, the fisherman will seem to be taller.
Does the apparent depth of a tank of water change if viewed obliquely? If so, does the apparent depth increase or decrease?
Ans: Yes, the apparent depth decreases. The tank's apparent depth of water varies when viewed obliquely.
This is because the light deviates on traveling from one medium to another. The tank's apparent depth, when viewed obliquely, is less than the near-normal viewing.
The refractive index of diamond is much greater than that of ordinary glass. Is this fact of some use to a diamond cutter?
Ans: The diamond's refractive index is more than that of ordinary glass.
The critical angle for diamond is smaller than that for glass.
A diamond cutter utilizes a large angle of incidence to guarantee that the light entering the diamond is totally reflected from its face. This is the cause for the sparkling effect of a diamond.
17.
Determine the 'effective focal length' of the combination of the two lenses in Exercise 9.10, if they are placed
Ans: Consider the diagram below which represents the combination of two lenses.
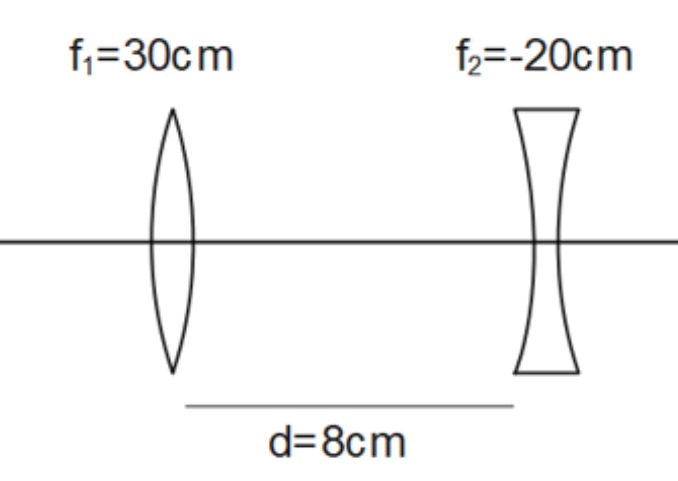
Here,
Focal length of the convex lens,
Focal length of the concave lens,
Distance between the two lenses,
First, consider the case when the parallel beam of light falls on the convex lens.
Using lens formula,
Where, object distance,
Image distance
The image will serve as a virtual object for the concave lens.
Using lens formula,
Where, object distance
Image distance
The parallel incident beam seems to diverge from a point, that is,
Secondly, when the parallel beam of light falls, from the left, on the concave lens;
Using lens formula,
Where, object distance,
Image distance
The image will serve as a real object for the convex lens.
Using lens formula,
Where, object distance,
Image distance
Hence, the parallel incident beam seems to diverge from a point, that is
Thus, the answer does depend on the combination side at which the parallel beam of light is incident. The notion of effective focal length does not appear to be useful for this combination.
An object
Ans: Consider the given diagram of the previous arrangement as follows:
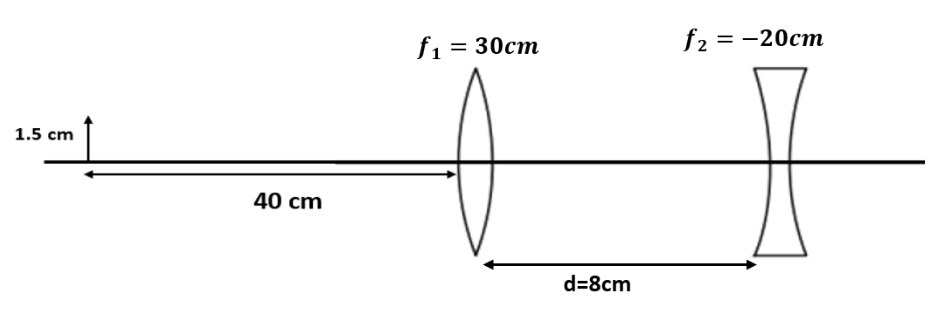
Here, it is said that,
Height of the image,
Object distance from the side of the convex lens,
Using lens formula,
Magnification,
Hence, the magnification due to the convex lens is
The image made by the convex lens acts as an object for the concave lens.
Using lens formula,
Where, object distance
Image distance
Magnification,
Hence, the magnification due to the concave lens is
The magnification due to the combination of the two lenses is calculated as:
Thus,
Clearly, the height of the image is
18. At what angle should a ray of light be incident on the face of a prism of refracting angle
Ans: Consider the given figure below:
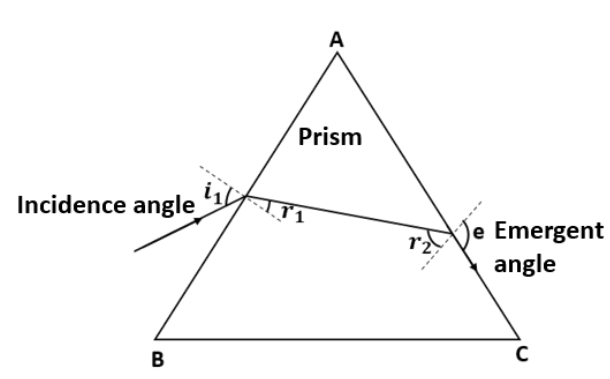
Angle of prism,
is the angle of incidence at the face AC.
Using Snell’s law,
For refraction through prism, angle
We get,
Using Snell’s law,
Hence, the incidence angle is
19. For a normal eye, the far point is at infinity and the near point of distinct vision is about
Ans: Given that,
Least distance of distinct vision,
Far point of a normal eye,
Converging power of the cornea,
Least converging power of the eye-lens,
To see the objects at infinity, the eye uses its least converging power.
Power of the eye-lens,
Power of the eye-lens is given as:
Object distance,
Image distance,
Using lens formula,
Power,
Power of the eye lens
Hence, the range of accommodation of the eye-lens is from
20.
The angle subtended at the eye by an object is equal to the angle subtended at the eye by the virtual image produced by a magnifying glass. In what sense then does a magnifying glass provide angular magnification?
Ans: Though the image size is larger than the object, the angular size of the image is equivalent to the angular size of the object.
A magnifying glass supports one seeing the objects closer than the least distance of distinct vision. A closer object produces a larger angular size.
A magnifying glass gives angular magnification. Without magnification, the object cannot be located closer to the eye. With magnification, the object can be set much closer to the eye.
In viewing through a magnifying glass, one usually positions one's eyes very close to the lens. Does angular magnification change if the eye is moved back?
Ans: Yes, the angular magnification varies.
When the length between the eye and a magnifying glass rises, the angular magnification reduces slightly because the subtended angle at the eye is imperceptibly less than the lenses.
Image distance does not have any impact on angular magnification.
Magnifying power of a simple microscope is inversely proportional to the focal length of the lens. What then stops us from using a convex lens of smaller and smaller focal length and achieving greater and greater magnifying power?
Ans: The convex lens's focal length cannot be reduced by a more significant amount.
This is because making lenses having tiny focal lengths is not easy.
Spherical and chromatic aberrations are created by a convex lens having a petite focal length.
Why must both the objective and the eyepiece of a compound microscope have short focal lengths?
Ans: The angular magnification produced by the compound microscope’s eyepiece is:
Where,
It can be seen that if
The angular magnification of the compound microscope’s objective lens is given as
The magnification is large when
In the case of a microscope, the object is placed close to the objective lens. Hence, the object distance is tiny.
Since
When viewing through a compound microscope, our eyes should be positioned not on the eyepiece but a short distance away from it for best viewing. Why? How much should be that short distance between the eye and eyepiece?
Ans: We cannot collect much-refracted light when we put our eyes too close to the compound microscope's eyepiece.
As a result, the field view reduces substantially. Hence, the image's clarity gets blurred.
The best view of the eye for seeing through a compound microscope is at the eye-ring connected to the eyepiece. The exact location of the eye depends on the separation between the objective lens and the eyepiece.
21. An angular magnification (magnifying power) of
Ans: Given that,
Focal length of the objective lens,
Focal length of the eyepiece,
Least distance of distinct vision,
Angular magnification of the compound microscope
Total magnifying power of the compound microscope,
The eyepiece’s angular magnification is given by:
The objective lens angular magnification is given by:
We have,
Using lens formula,
And,
The object should be placed
Using lens formula,
Where,
Separation between the eyepiece and the objective lens
Therefore, the separation between the eyepiece and the objective lens should be
22. A Cassegrain telescope uses two mirrors as shown in figure. Such a telescope is built with the mirrors
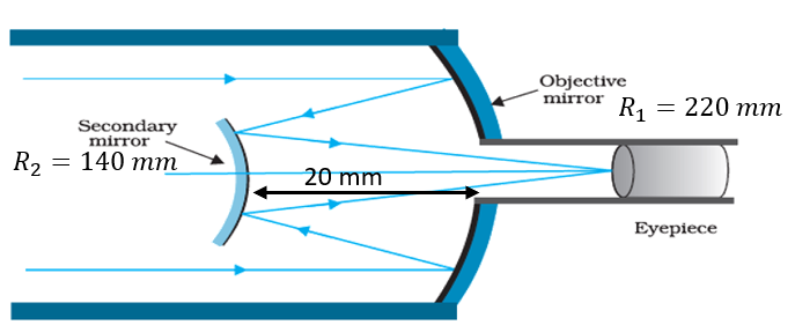
Ans: A Cassegrain telescope contains two mirrors, one is objective mirror and second one is secondary mirror. We need to find the distance of final image from secondary mirror.
Given: distance between the objective mirror and the secondary mirror,
Radius of curvature of the objective mirror,
Hence, objective mirror’s focal length,
Secondary mirror’s radius of curvature,
Secondary mirror’s focal length,
The image of an object set at infinity, made by the objective mirror, will serve as a virtual object for the secondary mirror.
Hence, the secondary mirror’s virtual object distance,
Using mirror formula,
Now, we put given values,
Clearly, the final image will be made
23. Figure shows an equiconvex lens (of refractive index
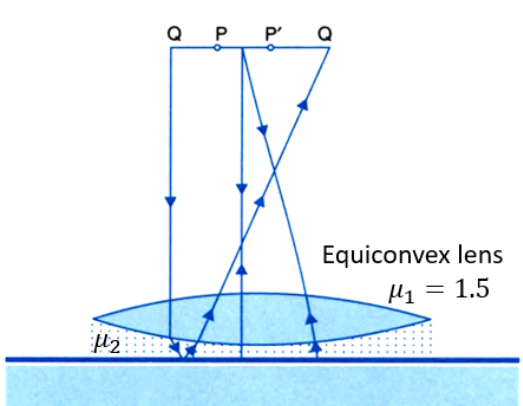
Ans: An equiconvex lens is in touch with a liquid layer on a plane mirror top. A small needle placed on the principal axis is moved along the axis until its inverted image will be at the position of the needle.
Convex lens’ focal length,
The liquid will behave as a mirror.
Liquid’s focal length
System’s focal length,
The equivalent focal length is given as:
Let the lens’s refractive index be
Hence, the curvature radius of the other surface is
We use,
Let
Liquid’s curvature radius on the side of the plane mirror
Curvature radius of the liquid on the side of the lens,
We use,
Clearly, the liquid’s refractive index is
Important Formulas from Class 12 Physics Chapter 9 Ray Optics and Optical Instrument
Mirror Formula:
where:
Lens Formula:
where,
Magnification (Mirror):
where,
Magnification (Lens):
where,
Power of a Lens:
where,
Combination of Lenses:
where,
Critical Angle:
where,
Refractive Index:
where,
Angular Magnification of Telescope:
where,
Resolving Power of Microscope:
where,
Benefits of Class 12 Physics Chapter 9 Ray Optics and Optical Instruments
Provides a foundation for advanced optical studies in engineering and physics.
Explains the working principles of daily-use optical devices like lenses, microscopes, and telescopes.
Enhances problem-solving skills with numerical questions and derivations.
Strengthens concepts needed for competitive exams like JEE, NEET, and more.
Prepares students for practical applications in fields like photography, astronomy, and medical imaging.
Tips to Study Class 12 Physics Chapter 9 Ray Optics and Optical Instruments Important Questions
Understand and practice all derivations for better conceptual clarity.
Memorise key formulas and practice applying them in numericals.
Focus on ray diagrams for mirrors, lenses, and optical instruments.
Solve NCERT textbook exercises thoroughly.
Practice problems involving real-life applications like lens combinations and optical power.
Watch animations or simulations for a better understanding of light behavior.
Related Study Materials for CBSE Class 12 Physics Chapter 9
S.No. | Important Study Material Links for Class 12 Physics Chapter 9 |
1. | |
2. | |
3. | Class 12 Ray Optics And Optical Instruments Exemplar Questions |
Conclusion
Ray Optics and Optical Instruments is an essential chapter 9 that bridges physics concepts with practical applications. By mastering its principles and formulas, students can excel in board exams and competitive exams. Use the FREE PDF of important questions provided by Vedantu also practice regularly, and achieve good score in your exams.
CBSE Class 12 Physics Chapter-wise Important Questions
CBSE Class 12 Physics Chapter-wise Important Questions and Answers cover topics from all other chapters, helping students prepare thoroughly by focusing on key topics for easier revision.
S.No | Chapter-wise Important Questions for Class 12 Physics |
1 | |
2 | Chapter 2 - Electrostatic Potential and Capacitance Important Questions |
3 | |
4 | Chapter 4 - Moving Charges and Magnetism Important Questions |
5 | |
6 | |
7 | |
8 | |
9 | |
10 | Chapter 11 - Dual Nature of Radiation and Matter Important Questions |
11 | |
12 | |
13 | Chapter 14 - Semiconductor Electronic: Material, Devices and Simple Circuits Important Questions |
Additional Study Materials for Class 12 Physics
S.No | Study Materials for Class 12 Physics |
1 | |
2 | |
3 | |
4 | |
5 | |
6 | |
7 | |
8 |
FAQs on Ray Optics And Optical Instruments Class 12 Important Questions: CBSE Physics Chapter 9
1. Why does a bird flying high in the air appear to be higher than in reality?
When the bird is flying high in the air, it is in a rare medium whereas the air near the ground is denser medium. When we see the bird, the light rays from the bird’s body travel from a rarer to denser medium, hence, the light rays bend towards the normal. This refraction of light causes the bird to appear flying higher in the air, than in reality.
2. What is meant by the power of a lens in Class 12 Chapter 9 Physics ?
The power of a lens is the inverse of its focal length. The S.I. unit for measuring the power of a lens is Dioptre (D). The focal length is the measure of distance from the principal foci of the lens to the center of the lens. From the definition of the power of a lens, it can be concluded that the focal length and the power of a lens are inversely proportional. The formula for the power and the focal length of a lens is given as follows:
Power = 1/focal length
Focal length, 1/f = 1/u + 1/v
3. As discussed in Class 12 Chapter 9 Physics What are the defects of a human eye?
The common defects of a human eye are as follows.
Myopia: This defect makes the nearby objects to be seen clearly and the far away objects to be seen as blurred. It is called short-sightedness. It can be corrected with the help of concave lenses.
Hyperopia: This defect causes the far away objects to be seen easily and the nearby objects to be seen as blurred. It is called farsightedness. It can be corrected with the help of convex lenses.
Astigmatism: This defect caused impaired vision due to which some parts of a picture may appear clear whereas some other parts of the same picture may appear blurred. It can be corrected with the help of spherical or cylindrical lenses.
4. Are the important questions for CBSE Class 12 Physics Chapter 9 - Ray Optics and Optical Instruments on Vedantu helpful for exam preparation?
Yes, the important questions for CBSE Class 12 Physics Chapter 9 - Ray Optics and Optical Instruments are very helpful for exam preparation. These questions are curated and consolidated by the in-house team of subject matter experts at Vedantu, in close reference with the NCERT textbook. These important questions are available in PDF format on our mobile application as well. The best part is that you can download and refer to the PDF of important questions for Class 12 Ray Optics for free from Vedantu.
5. As discussed in Class 12 Chapter 9 what is the lens formula, and how is it applied?
The lens formula 1f=1v−1u\frac{1}{f} = \frac{1}{v} - \frac{1}{u}f1=v1−u1 relates the focal length, object distance, and image distance for a lens.
7. What are the most important questions from Chapter 9 Class 12 Physics?
Focus on derivations, numerical problems involving lens and mirror combinations, and working principles of optical instruments.
9. How to calculate the power of a lens in Chapter 9 of Class 12 Physics?
Power PPP of a lens is calculated as P=100fP = \frac{100}{f}P=f100 (in diopters, fff in cm).
10. Are Class 12 NCERT exercises apart from important questions sufficient for Chapter 9 preparation?
NCERT exercises provide a strong foundation, but additional reference resources like important questions by Vedantu are helpful for advanced problem-solving.
11. Where can I download the FREE PDF of important questions for Chapter 9?
The FREE PDF is available on Vedantu websites created by our Vedantu master teachers and experts.

















