




What is a Collision?
A collision is a one-time event in which a strong force acts between two or more bodies for a brief period of time, causing the interacting particle's energy and momentum to change. Although the most common meaning of the word collision is an incident in which two or more objects clash violently, the scientific meaning of the phrase has nothing to do with the degree of the force. All collisions have something in common i.e. they all conserve the momentum. There are different types of collisions, and we’ll have a look at them.
In a collision, the physical contact between the bodies that collide is not really necessary. For example, when two billiard balls or a ball and bat collide, there is physical contact, yet when an alpha particle collides with a nucleus (i.e. Rutherford scattering experiment), there is no physical contact. When a boxer throws a punch at their opponent, we can say that their fist collides with the opponent. Similarly, when any astronomical object merges with a black hole in space, we can say that they collide. There are many such examples of collisions.
Types of Collisions
There are three main stages that can be identified in a collision. These are before, during, and after a collision. The interaction forces are zero in the before and after stages. The interaction forces between these two phases are enormous, and they are frequently the governing forces guiding body motion. Since the size of the interacting force is frequently unknown, Newton's second law cannot be applied.
However, the law of conservation of momentum can be used to relate the beginning and final velocities. As we have already mentioned before, the collisions always obey the law of conservation of momentum. They may or may not conserve kinetic energy, but momentum is always conserved.
Before moving on to the types of collisions, we will discuss the coefficient of restitution.
Coefficient of Restitution
The coefficient of restitution is the ratio of final velocity to initial velocity between two objects after they collide. The restitution coefficient abbreviated 'e,' is a unitless number with values ranging from 0 to 1. It is also known as the coefficient of resilience.
Collisions can be divided into three types depending on the conservation of kinetic energy. The three types of collisions based on the conservation of kinetic energy are:
Perfectly Elastic Collision
The collision is said to be perfectly elastic if the kinetic energy after the collision equals the kinetic energy before the collision. The coefficient of restitution for a perfectly elastic collision is equal to 1. This means that no kinetic energy is dissipated in the collision, and the objects will rebound from one another. The initial relative velocity will be equal to the final relative velocity.
Inelastic Collision
The collision is considered to be inelastic if the kinetic energy after the collision is not equal to the kinetic energy before the impact. The coefficient of restitution for an inelastic collision is between zero and one. The final kinetic energy might be greater than the initial energy, or it could be vice versa in this case. The majority of collisions are actually inelastic collisions.
Perfectly Inelastic Collision
The collision is considered to be perfectly inelastic if two bodies collide and stick together or travel at the same velocity after the contact. Since the final relative velocity of the particles is zero, the coefficient of restitution in a perfectly inelastic collision is zero. Although practically not all the kinetic energy is lost, it just means that the loss of kinetic energy is as large as it can be.
Line of Impact
The line of impact is the common normal at the point of contact between the bodies. The impact is called central impact if the mass centres of both colliding bodies are on the line of impact, and eccentric impact if the mass centres of both or any one of the colliding bodies are not on the line of impact. The line of impact in a collision is extremely important.
Oblique Collisions
If a two-particle collision is glancing, i.e. their directions of motion after a collision are not along the initial line of motion, the collision is called oblique. If in the oblique collision the particles before and after the collision are in the same plane, the collision is called 2-dimensional, otherwise 3-dimensional.
It can also be defined as the impact that occurs when the velocity vectors of both or any of the bodies are not parallel to the line of impact. The direction will be termed oblique direction. In an oblique elastic collision apart from the conditions mentioned above, the kinetic energy of the system will also be conserved.
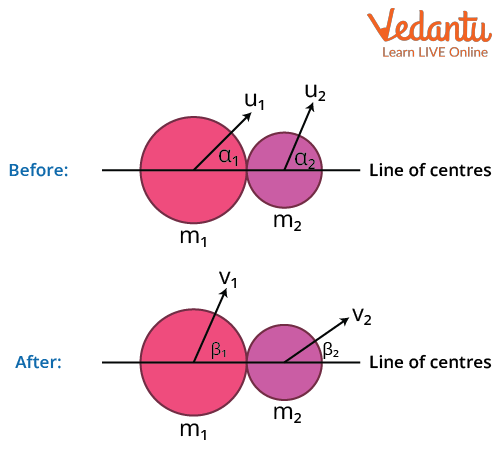
Image: Oblique Collision
Two-Dimensional Oblique Collision
We can write the law of conservation of momentum for the above system as:
$m_{1} v_{1} \cos \beta_{1}+m_{2} v_{2} \cos \beta_{2}=m_{1} u_{1} \cos \alpha_{1}+m_{2} u_{2} \cos \alpha_{2}$
If we assume that the balls are smooth, i.e. there are no forces perpendicular to the line of centres and that the balls are not rotated, then the component of momentum perpendicular to the line of centres of each ball is also conserved. So, we can write:
$\begin{align} &v_{1} \sin \beta_{1}=u_{1} \sin \alpha_{1} \\ &v_{2} \sin \beta_{2}=u_{2} \sin \alpha_{2} \end{align}$
The relative speed after the collisions in the horizontal direction can be written as:
$v_{2} \cos \beta_{2}-v_{1} \cos \beta_{1}$
Similarly, the relative speed before the collisions between the balls can be written as:
$u_{1} \cos \alpha_{1}-u_{2} \cos \alpha_{2}$
The coefficient of restitution can then be written as:
$\begin{align} &\dfrac{v_{2} \cos \beta_{2}-v_{1} \cos \beta_{1}}{u_{1} \cos \alpha_{1}-u_{2} \cos \alpha_{2}}=e \\ &v_{2} \cos \beta_{2}-v_{1} \cos \beta_{1}=e\left(u_{1} \cos \alpha_{1}-u_{2} \cos \alpha_{2}\right) \end{align}$
These formulas can be termed as the oblique collision formula. These formulas can be used to find the velocity after the collision.
Conclusion
A collision is a one-time event in which a strong force acts for a brief period of time between two or more bodies, changing the energy and momentum of the interacting particles. The law of conservation of momentum is always followed in collisions and the kinetic energy may or may not be conserved. If the kinetic energy is conserved, then the collision is called an elastic collision. If the kinetic energy is not conserved, then the collision is known as an inelastic collision. A perfectly elastic collision is the one in which the coefficient of restitution is zero. The impact that occurs when the velocity vectors of both or any of the bodies are not parallel to the line of impact is known as an oblique collision.
FAQs on Oblique Collisions - Types and Formula for JEE
1. Is oblique collision important for JEE?
Working knowledge of the topic is important for any student as it will help them solve other collision problems. Collisions are an important part of the chapter on Work, Energy, and Power. Collisions always follow the law of conservation of momentum and are an integral part of other topics apart from just mechanics. They are extensively used in modern physics. Oblique collisions help improve the conceptual framework and are thus important. Work, Power and Energy has a weightage of 3.3% in JEE.
2. What are the three collisions that occur in a car crash?
There are three sorts of collisions in a motor vehicle accident: vehicle collision, human collision and internal collision. The initial collision occurs when a vehicle collides with another item, which is most commonly linked with an automobile accident. The "human collision" is the name given to the second collision. In a car accident, the body may be damaged, even if it is not evident. This is the internal collision, which is the third collision.












