
A small roller of diameter 20 cm has an axle of diameter 10 cm (see figure below on the left). It is on a horizontal floor and a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle (see figure on the right). The scale is now pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved 50 cm, the position of the scale will look like this (figures are schematic and not drawn to scale)

A. 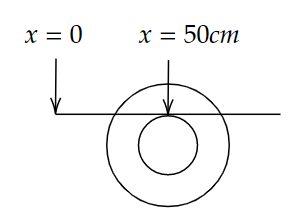
B. 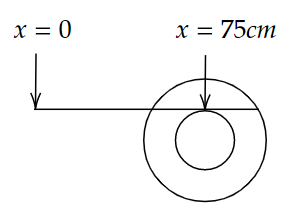
C. 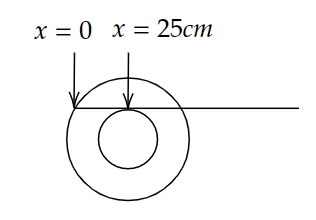
D. 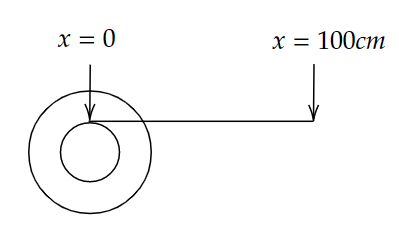
Answer
141k+ views
Hint: Before we start addressing the problem, we need to know about the linear and angular velocity. The velocity at which the body moves in a linear motion is known as linear velocity. The velocity at which the body moves in a rotational motion is known as angular velocity.
Formula Used:
The formula for the relation between linear and angular velocity is,
Where, v is linear velocity, r is radius and
Complete step by step solution:
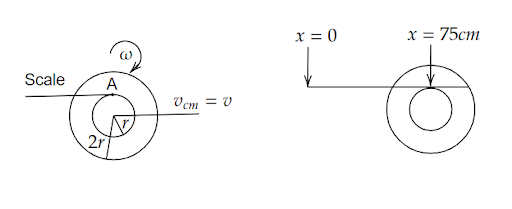
Consider a small roller placed on the horizontal floor of diameter 20 cm has an axle of a diameter of 10cm, a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle. Now the scale is pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved 50 cm, then we need to find how the position of the scale will look.
So,
Now,
If the ratio of the radius of the axle to the roller is
Since,
Now, we want to find the velocity at point A. Here, the axle will roll as well as move forward having both translational and rotational velocity that is,
After some time the roller has moved 50 cm on the ground that is,
Therefore, the position of the scale is 75 cm.
Hence, Option B is the correct answer
Note:Remember that, if a sphere of some radius r is rolling with some speed v, then it has both linear and angular velocity and the relation between these two is given by,
Formula Used:
The formula for the relation between linear and angular velocity is,
Where, v is linear velocity, r is radius and
Complete step by step solution:

Consider a small roller placed on the horizontal floor of diameter 20 cm has an axle of a diameter of 10cm, a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle. Now the scale is pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved 50 cm, then we need to find how the position of the scale will look.
So,
Now,
If the ratio of the radius of the axle to the roller is
Since,
Now, we want to find the velocity at point A. Here, the axle will roll as well as move forward having both translational and rotational velocity that is,
After some time the roller has moved 50 cm on the ground that is,
Therefore, the position of the scale is 75 cm.
Hence, Option B is the correct answer
Note:Remember that, if a sphere of some radius r is rolling with some speed v, then it has both linear and angular velocity and the relation between these two is given by,
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
EMI starts from ₹2,775 per month
Recently Updated Pages
Difference Between Circuit Switching and Packet Switching

Difference Between Mass and Weight

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

JEE Main Maths Paper Pattern 2025 – Marking, Sections & Tips

Sign up for JEE Main 2025 Live Classes - Vedantu

JEE Main 2025 Helpline Numbers - Center Contact, Phone Number, Address

Trending doubts
Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Electron Gain Enthalpy and Electron Affinity for JEE

Physics Average Value and RMS Value JEE Main 2025

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Collision - Important Concepts and Tips for JEE

Introduction to Dimensions With Different Units and Formula for JEE

Other Pages
Displacement-Time Graph and Velocity-Time Graph for JEE

The dimensional formula of k Coulombs Constant is Take class 11 physics JEE_Main

NCERT Solutions for Class 11 Physics Chapter 4 Laws of Motion

NCERT Solutions for Class 11 Physics Chapter 3 Motion In A Plane

NCERT Solutions for Class 11 Physics In Hindi Chapter 1 Physical World

The thickness of the depletion layer is approximately class 11 physics JEE_Main




