NCERT Solutions for Class 11 Physics Chapter 3 Motion in a Plane - FREE PDF Download
FAQs on NCERT Solutions for Class 11 Physics Chapter 3 Motion In A Plane
1. What is the difference between scalar and vector quantities?
A quantity that has a magnitude but lacks a direction is termed as a scalar quantity. A quantity that has both magnitudes, as well as a direction, is termed as a vector quantity. A scalar quantity is always a dot product of two quantities, whereas the vector is always the cross product of two vector quantities. Examples of scalar quantities are mass, density and current. Some examples of vector quantity are acceleration, velocity and displacement. The difference between these two is very easy to understand.
2. What is linear motion?
Linear motion is also referred to as rectilinear motion. The motion is termed as a one-dimensional motion along a straight line, and thus can be explained using only one spatial dimension. There are two types of linear motion: uniform linear motion with a constant velocity and zero acceleration and non-uniform linear motion with variable velocity and non-zero acceleration. It is referred to as the most basic among all the motions. Linear motion, to some extent, can be compared with a general motion. An example of linear motion is an athlete running in on a straight track.
3. What type of questions are present in Chapter 4 ‘Motion in a Plane’ of the NCERT Solutions for Class 11 Physics?
There are a variety of questions present in Chapter 4 ‘Motion in a Plane’ in NCERT Solutions for Class 11 Physics. The questions will range from detailed to short answer-type. This will contain pick/choose the one out, true or false, reason stating questions, numerical or problem questions, long answer questions etc. Therefore, you will find everything in one place and don’t have to seek other places to study thoroughly.
4. Define uniform circular motion according to Chapter 4 of the NCERT Solutions for Class 11 Physics.
Circular motion is the path that leads to the circular movement of the body. Likewise, when the motion of the body in the circular path is followed by a constant speed, it is called uniform circular motion. The speed of the body is the same but with a change in velocity. When the object is moving around the circle, it is continuously changing its direction. The object touches the edge of the circle but does not cross it.
5. Can I get full marks in Chapter 4 ‘Motion in a plane’ of Class 11 Physics?
Yes, it is possible to get full marks in Class 11 Physics Chapter 4 Motion in a Plane. However, do remember that even a small mistake can lead to the deduction of the marks. Don’t try to apply the shortcuts, especially in the numerical. Try to give the full detailing about the equations used and the process for getting the answer in the numerical.
6. According to Motion in a Plane Class 11 NCERT Solutions, Is motion in a plane easy?
The difficulty of understanding motion in a plane mentioned in motion in a Plane class 11 NCERT solutions depends on the student's grasp of basic vector algebra and kinematics. For those who are comfortable with these concepts, motion in a plane can be straightforward. The topic involves understanding how to break down two-dimensional motion into components and apply the equations of motion to each component independently.
7. What law of motion is in a plane, explained in Class 11 Physics Chapter 3?
Motion in a plane NCERT class 11 physics chapter 3 exercise solutions pdf typically involves Newton's laws of motion applied to two dimensions. Key concepts include:
First Law (Inertia): An object will remain at rest or in uniform motion unless acted upon by an external force.
Second Law (F = ma): The acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass.
Third Law (Action and Reaction): For every action, there is an equal and opposite reaction.
8. How many types of motion are there in a plane, explained in Class 11 Physics Motion in a Plane Exercise Solutions.
There are primarily two types of motion in a plane class 11 physics chapter 3 exercise solutions:
Projectile Motion: The motion of an object thrown into the air, subject only to acceleration due to gravity.
Uniform Circular Motion: The motion of an object moving at a constant speed along a circular path.
9. What is motion in a plane can be treated as in Physics Chapter 3 Class 11?
Motion in a plane class 11 can be treated as the combination of two independent linear motions in perpendicular directions. For example, projectile motion can be analysed as two separate motions: horizontal motion with constant velocity and vertical motion with constant acceleration due to gravity.
10. How to solve motion in a plane problem explained in Motion In Plane Class 11 NCERT Solutions?
To solve problems involving motion in a plane mentioned in Class 11 Physics Chapter 3 Exercise Solutions:
Break Down the Motion: Separate the motion into horizontal and vertical components.
Apply Kinematic Equations: Use the appropriate kinematic equations for each component.
Combine Results: Use vector addition to combine the horizontal and vertical components of displacement, velocity, and acceleration.
Use Trigonometry: Employ trigonometric relationships to find angles and magnitudes.
11. What is the primary focus of motion in a plane class 11 ncert solutions?
The primary focus of the chapter motion in a plane class 11 ncert solutions is to introduce students to the concepts of vector and scalar quantities, and to analyse motion in two dimensions using vectors. The chapter covers topics such as displacement, velocity, acceleration, and projectile motion.
12. What are vectors, and how are they different from scalars mentioned in motion in a plane class 11 ncert solutions?
From class 11 physics chapter 3 exercise solutions, Vectors are quantities that have both magnitude and direction, such as velocity and force. Scalars, on the other hand, have only magnitude and no direction, such as temperature and mass.
13. What is the significance of resolving vectors into components mentioned in motion in a plane class 11?
Resolving vectors into components simplifies the analysis of motion in two dimensions. By breaking a vector into its horizontal and vertical components, it becomes easier to apply the equations of motion independently to each direction.
14. Can you explain the concept of relative velocity in two dimensions mentioned in ncert class 11 physics chapter 3 exercise solutions PDF download?
Relative velocity in two dimensions involves determining the velocity of one object as observed from another moving object, discussed in motion in a plane class 11 ncert solutions. It is found by vector subtraction of the velocity vectors of the two objects.
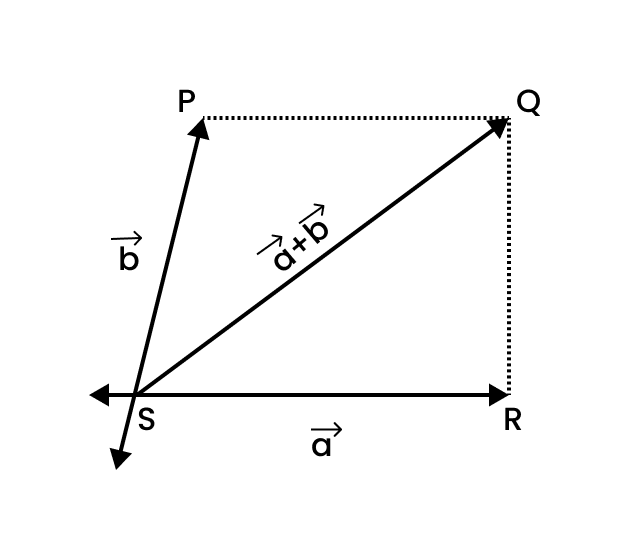
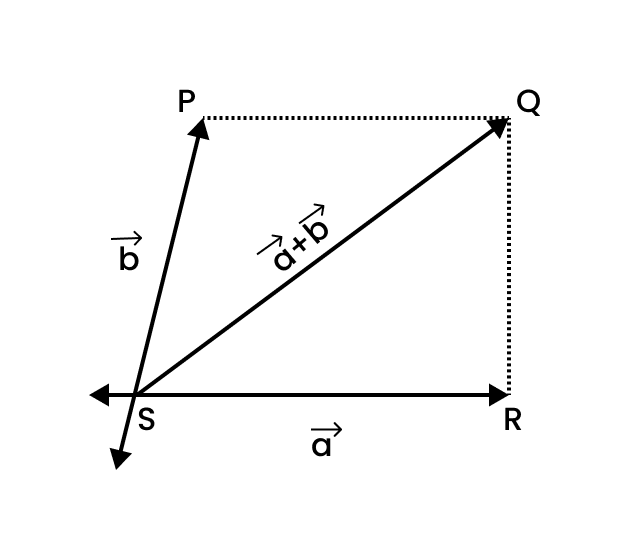
15. According to ncert class 11 physics chapter 3 exercise solutions PDF download, how does the addition of vectors work?
In class 11 physics chapter 3 exercise solutions, the addition of vectors can be done using the head-to-tail method or by resolving the vectors into their components and adding the corresponding components. The resultant vector is found by combining these components.
16. What is the significance of resolving vectors into components, discussed in class 11 physics chapter 3 exercise solutions?
In physics chapter 3 class 11, resolving vectors into components simplifies the analysis of motion in two dimensions. By breaking a vector into its horizontal and vertical components, it becomes easier to apply the equations of motion independently to each direction.