Answer
110.7k+ views
Hint: We will use the formula for critical angle, i.e., $\sin C = \dfrac{1}{{{\mu _1}}} = \dfrac{{{\mu _2}}}{{{\mu _1}}}$. refractive index of the first medium with respect to the second medium; \[{\mu _1} = \] refractive index of denser medium and \[{\mu _2} = \] refractive index of rarer medium.
Complete step by step answer:
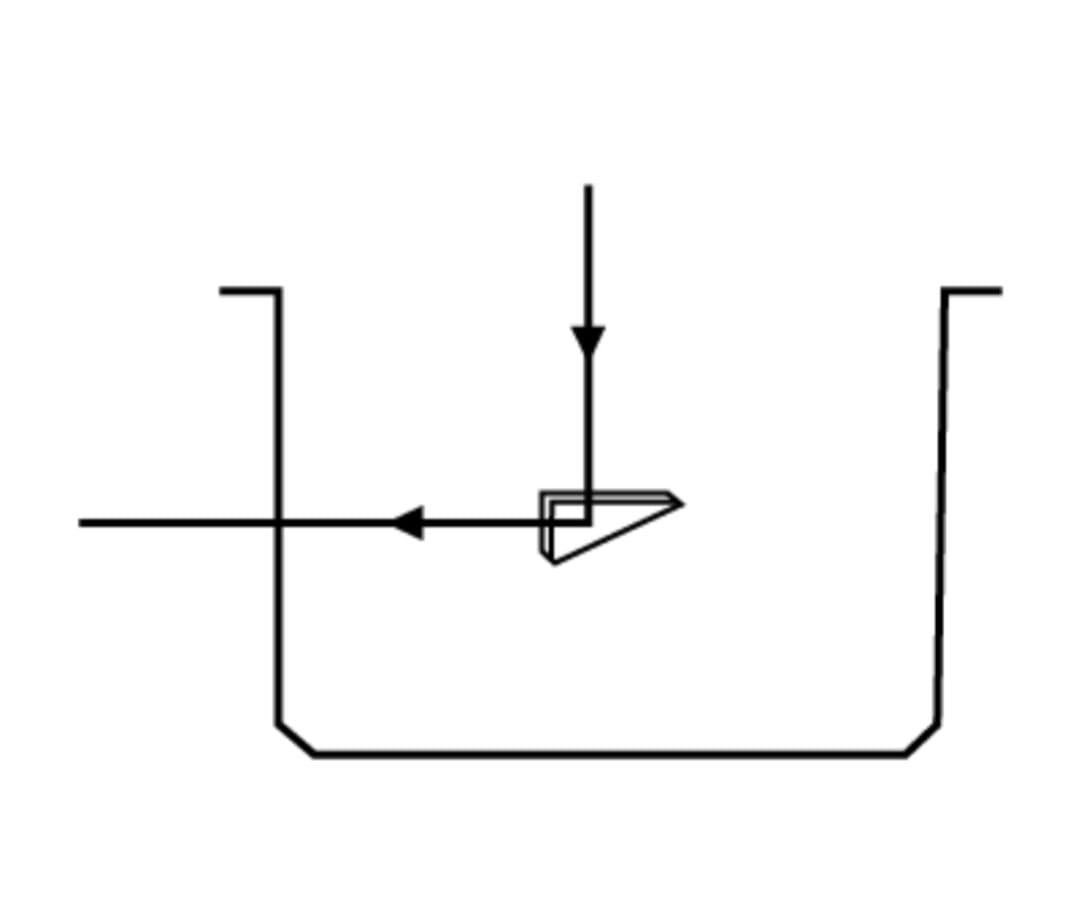
We know the formula for finding the critical angle is $\sin C = \dfrac{1}{{{\mu _1}}} = \dfrac{{{\mu _2}}}{{{\mu _1}}}$ …………………. (i)
The value of the refractive index of the liquid w.r.t the prism is given as \[\sqrt 2 \].
\[\sin C = \dfrac{1}{{\sqrt 2 }}\] (on substituting the value of \[_2{\mu _1}\] in Eq., (i)
\[ \Rightarrow C = {\sin ^{ - 1}}\left( {\dfrac{1}{{\sqrt 2 }}} \right)\]
\[ \Rightarrow C = 45^\circ \]
This means that both assertion and reason are true and the reason is the correct explanation for assertion.
Hence, option (a.) is the correct answer.
Note: The assertion and reason both hold true only for the given values of refractive index and critical angle. So, we will always have to calculate and check the answer.
Complete step by step answer:
We know the formula for finding the critical angle is $\sin C = \dfrac{1}{{{\mu _1}}} = \dfrac{{{\mu _2}}}{{{\mu _1}}}$ …………………. (i)
The value of the refractive index of the liquid w.r.t the prism is given as \[\sqrt 2 \].
\[\sin C = \dfrac{1}{{\sqrt 2 }}\] (on substituting the value of \[_2{\mu _1}\] in Eq., (i)
\[ \Rightarrow C = {\sin ^{ - 1}}\left( {\dfrac{1}{{\sqrt 2 }}} \right)\]
\[ \Rightarrow C = 45^\circ \]
This means that both assertion and reason are true and the reason is the correct explanation for assertion.
Hence, option (a.) is the correct answer.
Note: The assertion and reason both hold true only for the given values of refractive index and critical angle. So, we will always have to calculate and check the answer.
Recently Updated Pages
Write an article on the need and importance of sports class 10 english JEE_Main

Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

If x2 hx 21 0x2 3hx + 35 0h 0 has a common root then class 10 maths JEE_Main

The radius of a sector is 12 cm and the angle is 120circ class 10 maths JEE_Main

For what value of x function fleft x right x4 4x3 + class 10 maths JEE_Main