
What is current through the $5\Omega $ resistor?
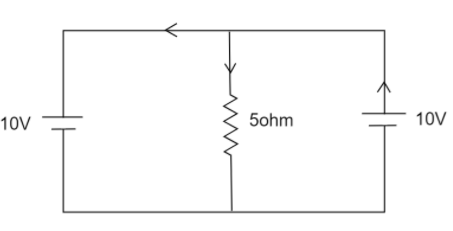
Answer
135k+ views
Hint: Here we have a closed-loop of a conductor that we can divide into two elementary loops to simplify the problem. Also, we can use one of Kirchhoff’s laws to figure out our desired answer. Once we learn physics here, then it is a fundamental problem of algebra.
Complete step by step solution:
We take the closed-loop of current and part them into two sections $A$ and $B$ as shown in the figure below-
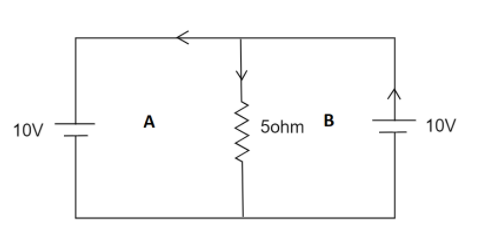
In both the current-carrying loop, the current is flowing counter-clockwise. So here we use Kirchhoff’s Voltage Law (KVL) to find out the current through the $5\Omega $ resistor.
Kirchhoff’s Second Law or Kirchhoff’s Voltage Law states that the algebraic sum of all the voltages around any closed loop in that circuit equals zero for a series of the closed-loop path.
We assume that the current through the $5\Omega $ resistor is $i$ (ampere).
According to Kirchhoff’s Voltage Law in the loop $A$-
$\Rightarrow 10 - 5i = 0$ ………$(1)$
Since the voltage difference across the resistor $10V$ and $i$ current is flowing through the resistor.
According to Kirchhoff’s Voltage Law in the loop $B$-
$\Rightarrow 10 - 5i = 0$ ………$(2)$
Since here also the voltage difference across the resistor $10V$ and $i$ current is flowing through the resistor.
So from both the above two equations, we can find out the value of $i$-
Hence,
$\Rightarrow 5i = 10$
$ \Rightarrow i = \dfrac{{10}}{5}$
$ \Rightarrow i = 2A$
Therefore, the current through the $5\Omega $ resistor is $2A$.
Note: We can use Kirchhoff’s Second Law, i.e., Kirchhoff’s Current Law, to determine the current at a junction in a closed current-carrying loop. A point of caution, we can only use these laws in a closed loop. These laws are advantageous in complex electric circuits.
Complete step by step solution:
We take the closed-loop of current and part them into two sections $A$ and $B$ as shown in the figure below-

In both the current-carrying loop, the current is flowing counter-clockwise. So here we use Kirchhoff’s Voltage Law (KVL) to find out the current through the $5\Omega $ resistor.
Kirchhoff’s Second Law or Kirchhoff’s Voltage Law states that the algebraic sum of all the voltages around any closed loop in that circuit equals zero for a series of the closed-loop path.
We assume that the current through the $5\Omega $ resistor is $i$ (ampere).
According to Kirchhoff’s Voltage Law in the loop $A$-
$\Rightarrow 10 - 5i = 0$ ………$(1)$
Since the voltage difference across the resistor $10V$ and $i$ current is flowing through the resistor.
According to Kirchhoff’s Voltage Law in the loop $B$-
$\Rightarrow 10 - 5i = 0$ ………$(2)$
Since here also the voltage difference across the resistor $10V$ and $i$ current is flowing through the resistor.
So from both the above two equations, we can find out the value of $i$-
Hence,
$\Rightarrow 5i = 10$
$ \Rightarrow i = \dfrac{{10}}{5}$
$ \Rightarrow i = 2A$
Therefore, the current through the $5\Omega $ resistor is $2A$.
Note: We can use Kirchhoff’s Second Law, i.e., Kirchhoff’s Current Law, to determine the current at a junction in a closed current-carrying loop. A point of caution, we can only use these laws in a closed loop. These laws are advantageous in complex electric circuits.
Recently Updated Pages
JEE Main 2021 July 25 Shift 2 Question Paper with Answer Key

JEE Main 2021 July 25 Shift 1 Question Paper with Answer Key

JEE Main 2021 July 20 Shift 2 Question Paper with Answer Key

JEE Main 2021 July 22 Shift 2 Question Paper with Answer Key

How to find Oxidation Number - Important Concepts for JEE

Half-Life of Order Reactions - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Degree of Dissociation and Its Formula With Solved Example for JEE

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Elastic Collisions in One Dimension - JEE Important Topic

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Displacement-Time Graph and Velocity-Time Graph for JEE

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

JEE Advanced 2024 Syllabus Weightage

JEE Main Chemistry Question Paper with Answer Keys and Solutions




