Question

Fire is caught at the height of 125m from the fire brigade. To extinguish the fire, water is coming from the pipe of cross-section 6.4 cm with a rate of 950 litres/min. Find out the minimum velocity of water exiting from fire brigade tank ($g = 10 m/{s^2}$)
A) 5 m/s
B) 10 m/s
C) 25 m/s
D) 50 m/s

Answer 1

Hint: In order to solve this problem we need to first understand the concept of the volumetric flow rate of a fluid. The volume of fluid flowing per unit time through a particular section is now as volumetric flow rate. Volumetric flow rate is measured in m3/min or litres/min.

Complete step by step answer:
 It is given in the problem that the diameter of the pipe through which the water is coming out is 6.4 cm, i.e. after conversion of the unit into meters we have, $d = 0.064m$
Also, the volumetric flow rate of water (Q) is given to be 950 litres/min,
i.e. $Q = 950l/\min = \dfrac{{950 \times {{10}^{ - 3}}}}{{60}}{m^3}/s$
$\Rightarrow Q = \dfrac{{95 \times {{10}^{ - 3}}}}{6}{m^3}/s$
The volumetric flow rate of the water can be calculated by taking the product of the cross-sectional area of the pipe and the velocity of water in a direction perpendicular to this cross-section, as shown in the following figure.


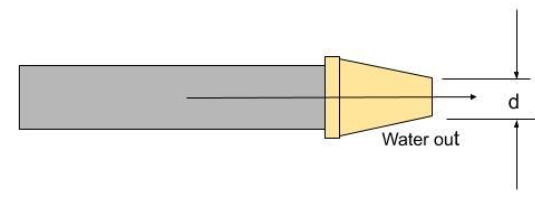
Therefore the volumetric flow rate is given by, $Q = A \times V$
Using the above equation the velocity of water is given by,
$V = \dfrac{Q}{A}$ ......... (1)
The cross-sectional area of the pipe is given by, $A = \dfrac{\pi }{4}{d^2} = \dfrac{\pi }{4}{0.064^2}$
Putting the values of area and discharge in equation (1) we get,
$V = \dfrac{{\left( {\dfrac{{95 \times {{10}^{ - 3}}}}{6}} \right)}}{{\left( {\dfrac{\pi }{4}{{0.064}^2}} \right)}}$
$\therefore V = 4.921m/s \approx 5m/s$

As the velocity of the fluid comes out to be 5 m/s, we can say that option (A) is the correct answer option.

Note: It is very important to use the term volumetric flow rate because just saying flow rate can create confusion. Flow rate can either be mass flow rate or volumetric flow rate. The mass flow rate is measured in kg/s. For kinematics of fluids (velocity and acceleration calculations), we use a volumetric flow rate.