Question

For a regular hexagon with apothem 5 m, the side length is about 5.77 m. The area of the regular hexagon is \[\left( \text{in }{{m}^{2}} \right).\]
(a) 75.5
(b) 85.5
(c) 76.5
(d) 86.5

Answer 1

Hint: To solve the given question, we will first find out what a regular hexagon is and what an apothem in a regular hexagon is. Then we will draw a rough sketch of the hexagon and we will make an apothem in it. Then, we will join the center of the hexagon with the two consecutive vertexes of the hexagon. After doing this, we will find the area of the triangle formed by the construction. Then we will multiply this area by 6 to get the total area of the regular hexagon.

Complete step-by-step answer:
Before solving the question, we must know what a regular hexagon is and what an apothem is. A hexagon is a polygon that has 6 sides and 6 interior angles. An apothem of a hexagon is a line segment from the center of a hexagon to the middle point of any one side of the hexagon. A rough sketch of a hexagon with an apothem is shown.

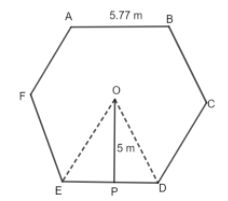
In the above figure, OP is the apothem. Now, we will find the area of triangle ODE. The area of any triangle with the base b and height h is given by the formula shown below.
\[\text{Area}=\dfrac{1}{2}\times b\times h\]
In our case, b = DE and h = OP. Thus, we have,
\[\text{Area}=\dfrac{1}{2}\times DE\times OP\]
\[\Rightarrow \text{Area of DE}=\dfrac{1}{2}\times 5.77m\times 5m\]
\[\Rightarrow \text{Area of }\Delta \text{ODE}=14.425{{m}^{2}}\]
Now, there will be a total of 6 similar triangles, so the area of the hexagon will be six times the area of triangle ODE. Thus, we have,
\[\text{Area of hexagon}=6\times \text{Area of }\Delta \text{ODE}\]
\[\Rightarrow \text{Area of hexagon}=6\times 14.425{{m}^{2}}\]
\[\Rightarrow \text{Area of hexagon}=86.55{{m}^{2}}=86.5{{m}^{2}}\]
Hence, option (d) is the right answer.

Note: An alternate way of solving the above question is shown below. The area of the hexagon with side ‘s’ is given by the formula shown.
\[\text{Area of hexagon}=\dfrac{3\sqrt{3}}{2}{{s}^{2}}\]
where s = 5.77 m in our case. Thus, we will get,
\[\text{Area of hexagon}=\dfrac{3\sqrt{3}}{2}{{\left( 5.77m \right)}^{2}}\]
\[\Rightarrow \text{Area of hexagon}=\dfrac{3\times 1.732}{2}\times 33.2929{{m}^{2}}\]
\[\Rightarrow \text{Area of hexagon}=86.4949{{m}^{2}}\simeq 86.5{{m}^{2}}\]