Question

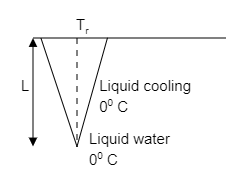
Icicles. Liquid water coats an active (growing) icicle and extends up a short, narrow tube along the central axis. Because the water ice interface must have a temperature of ${0^ \circ }\,C $, the water in the tube cannot lose energy through the sides of the icicle or down through the tip because there are no temperature changes in those directions. It can lose energy and freeze only by sending energy up through distance $L $ to the top of the icicle, where the temperature ${T_r} $ can be below ${0^ \circ }\,C $. Take $L = 0.12\,m $ and ${T_r} = - {5^ \circ }\,C $. Assume that the central tube and the upward conduction path both have cross sectional area $A $. In terms of $A $, what rate is mass converted from liquid to ice at the top of the central tube?

Answer 1

Hint The rate of the mass converted from liquid to the ice at the top of the central tube can be determined by using the heat of fusion formula and this formula is differentiated with respect to the time, then the rate of the mass converted from liquid to ice can be determined.
Useful formula
The heat of the fusion can be given by,
 $Q = {L_F}m $
Where, $Q $ is the heat of the fusion, ${L_F} $ is the constant and $m $ is the mass.

Complete step by step answer
Given that,
The length of the tube is, $L = 0.12\,m $,
The temperature of the liquid is, ${T_r} = - {5^ \circ }\,C $.
Now,
The heat of the fusion can be given by,
 $Q = {L_F}m $
By differentiating the above equation with respect to the time, then the above equation is written as,
 $\dfrac{{dQ}}{{dt}} = {L_F}\dfrac{{dm}}{{dt}} $
The above equation is also written as,
 ${P_{cond}} = \dfrac{{dQ}}{{dt}} = {L_F}\dfrac{{dm}}{{dt}} $
By rearranging the terms in the above equation, then the above equation is written as,
 $\dfrac{{{P_{cond}}}}{{{L_F}}} = \dfrac{{dm}}{{dt}} $
The value of the ${P_{cond}} = 16.7\,AW $ and the value of the ${L_F} = 3.33 \times {10^5}\,Jk{g^{ - 1}} $.
By substituting the both values in the above equation, then the above equation is written as,
 $\dfrac{{dm}}{{dt}} = \dfrac{{16.7\,AW}}{{3.33 \times {{10}^5}\,Jk{g^{ - 1}}}} $
By dividing the terms in the above equation, then the above equation is written as,
 $\dfrac{{dm}}{{dt}} = 5.02 \times {10^{ - 5}}\,kg{s^{ - 1}} $

Thus, the above equation shows the rate is mass converted from liquid to ice at the top of the central tube.

Note The rate of the mass of the liquid converted to the ice is directly proportional to the ${P_{cond}} $ and the rate of the mass of the liquid converted to the ice is inversely proportional to the ${L_F} $. As the ${P_{cond}} $ increases, the rate of the mass of the liquid converted to the ice also increases.