Straight Lines and Circles: JEE Important Topic

A straight line can be defined as a straight figure that is not curved having zero width. It can be represented by a linear equation, e.g., $ax+by+c=0$, where $a$ and $b$ are coefficients and $c$ is the constant. There are various forms of equations of a straight line that have been introduced in earlier classes, such as Point-slope form, Two-Point form, Slope-Intercept form.

A circle is defined as a two-dimensional shape that consists of all points in a plane that are equidistant from a fixed point, known as the centre of the circle.

The general equation of a circle having centre at (h,k) and radius equal to ‘r’ is given as ${{\left( x-h \right)}^{2}}+{{\left( y-k \right)}^{2}}={{r}^{2}}$. In complex plane, the equation of a straight line is given as $\left| \left. z-p \right| \right.=\left| \left. z-q \right| \right.$, where ‘p’  and ‘q’ are two distinct complex numbers.

The above line is bisecting the line segment joining the points p and q. It is the geometric way for the straight-line equation.

Forms of Equations of a Straight Line

• Point-Slope Form- Let us consider a point $A\left( {{x}_{1}},\text{ }{{y}_{1}} \right)$ through which a line is passing and its slope be $m$. Then, the equation of the line is given as $y\text{ }-\text{ }{{y}_{1}}=\text{ }m\left( x\text{ }\text{-}{{x}_{1}} \right)$.

• Two-Point Form- Let us consider two fixed points as $A\left( {{x}_{1}},\text{ }{{y}_{1}} \right)$ & $B\text{ }\left( {{x}_{2}},\text{ }{{y}_{2}} \right)$. Then, the line joining these points can be represented by the equation $y-{{y}_{1}}=\frac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}\left( x-{{x}_{1}} \right).$ 

• Slope-Intercept Form- Let $c$ be the $y-$ intercept of a line, i.e., the line passes through a point (0, c). If the slope of the line is $m$, then, the equation of the line is given as $y\text{ }=\text{ }mx\text{ }+\text{ }c.$

• Intercept Form- Consider a line L with x-intercept a and y-intercept b; the line will intersect the X- axis at $(a,0)$ and the Y- axis at $(0,b)$. Then the equation of the line is given as:

$\dfrac{x}{a}+\dfrac{y}{b}=1$

• Normal Form: Consider a perpendicular from the origin to line L with length l that forms an angle with the positive X-axis. Then the equation of the line is given as:

$xcos\beta+ysin\beta=l$

Equation of Straight Line in Complex Plane

A complex number is an expression of the form $z=x+iy$ where $x$ is the real part of $z$ and $y$ is the imaginary part. 

The complex conjugate of $z=x+iy$ is defined as $\bar{z}=x-iy$

Now, we know that $z=x+iy$ and $\bar{z}=x-iy$.

Therefore, $x=\dfrac{z+\bar{z}}{2}$ and $y=\dfrac{z-\bar{z}}{2i}$

The general equation of a straight line is given as $ax+by+c=0$.

So, using the values of x and y, we can write the equation as 

$a(\dfrac{z+\bar{z}}{2})+b(\dfrac{z-\bar{z}}{2i})+c=0$ 

As we know that $i^2 = -1$

$\Rightarrow az+a\bar{z}-bi(z-\bar{z})+2c=0$

$\Rightarrow z(a-bi)+\bar{z}(a+bi)+C=0$

Let $a'=a+bi$ and $\bar{a'}=a-bi$

Then, $\bar{a'}z+\bar{z}a’+C=0$  where $a’$ is a complex number and $C$ is a real number      …… (1)

The above equation is a general equation of a straight line in a complex plane.

The slope of the straight line $\bar{a'}z+\bar{z}a’+C=0$ is given as $-\dfrac{\text{cofficient of }\bar{z}}{\text{cofficient of }z}$, i.e., $\dfrac{-a’}{\bar{a’}}$.

Parametric Equation of a Straight Line

Let ${{z}_{1}}$ and ${{z}_{2}}$ are two points in the complex plane. And z be any arbitrary point on the line joining the two points as shown below.

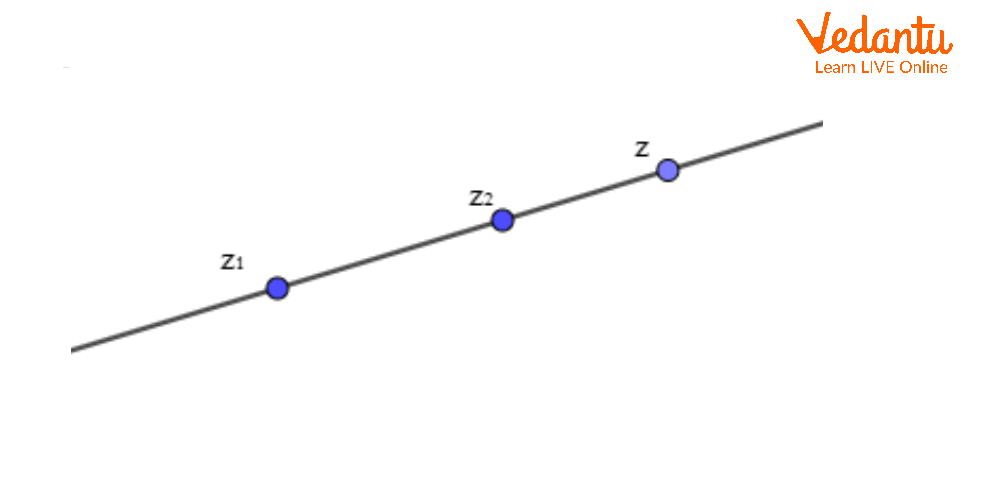
A Line in Complex Plane

If we view the complex numbers as vectors, then $z-{{z}_{1}}$needs to be in the same direction as $z-{{z}_{2}}$. Then, $z-{{z}_{1}}$ can be obtained by multiplying $z-{{z}_{2}}$ with some scalar, say $\lambda $, i.e., $z-{{z}_{1}}=\lambda \left( z-{{z}_{2}} \right),$where $\lambda \epsilon \mathbb{R}$

$\Rightarrow z-\lambda z={{z}_{1}}-\lambda {{z}_{2}}$

$\Rightarrow z=(\frac{1}{1-\lambda }){{z}_{1}}-(\frac{\lambda }{1-\lambda }){{z}_{2}}$

$\Rightarrow z=t{{z}_{1}}+(1-t){{z}_{2}}$ where $t=\frac{1}{1-\lambda} \epsilon\mathbb{R}$   …… (2)

The above equation is a parametric form of a straight line in a complex plane.

Condition for Two Lines to be Parallel

In Coordinate Geometry: If two straight lines with slope $m_1$ and $m_2$ are parallel to each other then Slope of both lines will be equal.

Therefore $m_1 = m_2$

In the Complex Plane: Let us consider two parallel lines as shown below.

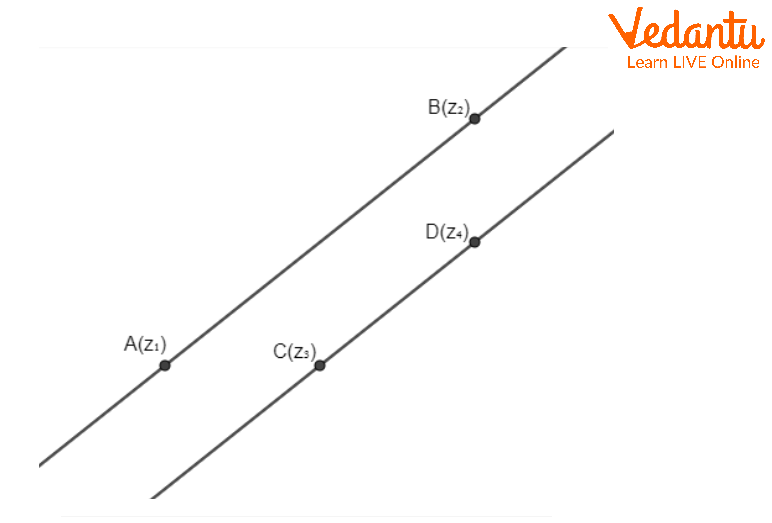
Two Parallel Lines in Complex Plane

Slope of line AB = $\dfrac{z_{1}-z_{2}}{\bar{z_{1}}-\bar{z_{2}}}$

Slope of line CD = $\dfrac{z_{3}-z_{4}}{\bar{z_{3}}-\bar{z_{4}}}$

Now, if the above two lines are parallel, then 

Arg$\left(\dfrac{z_{1}-z_{2}}{{z_{3}}-{z_{4}}}\right)$ = $0$ and $\pi$

Since its argument is 0 and $\pi$ then this point will lie on a real axis. Therefore we can write using the condition $z= \bar{z}$,

$\left(\dfrac{z_{1}-z_{2}}{{z_{3}}-{z_{4}}}\right)=\left(\dfrac{\bar{z_{1}}-\bar{z_{2}}}{{\bar{z_{3}}}-{\bar{z_{4}}}}\right) $

$\dfrac{z_{1}-z_{2}}{\bar{z_{1}}-\bar{z_{2}}}$=$\dfrac{z_{3}-z_{4}}{\bar{z_{3}}-\bar{z_{4}}}$

Therefore, if ${{w}_{1}}$ and ${{w}_{2}}$ are slopes of two lines $AB$ and $CD$ respectively, then the condition for the lines to be parallel is ${{w}_{1}}={{w}_{2}}$.

Condition for Two Lines to Be Perpendicular

In Coordinate Geometry: If two straight lines with slope $m_1$ and $m_2$ are perpendicular to each other then the relation between slope of both lines will be:

$m_1 \times m_2 = -1$

In the Complex Plane: Let us consider two perpendicular lines as shown below.

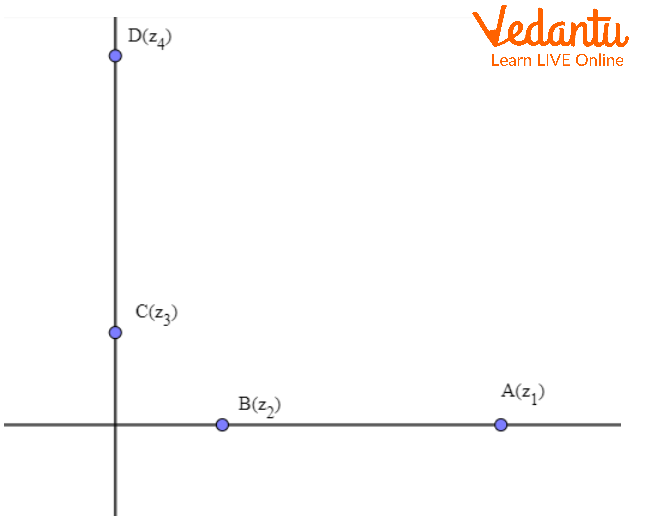
Two Perpendicular Lines in Complex Plane

Slope of line AB = $\dfrac{z_{1}-z_{2}}{\bar{z_{1}}-\bar{z_{2}}}$

Slope of line CD = $\dfrac{z_{3}-z_{4}}{\bar{z_{3}}-\bar{z_{4}}}$

Now, if the above two lines are perpendicular, then 

Arg$\left(\dfrac{z_{1}-z_{2}}{{z_{3}}-{z_{4}}}\right)$ = $\pm \dfrac{\pi}{2}$

Since its argument is  $\pm \dfrac{\pi}{2}$ then this point will lie on an imaginary axis. Therefore we can write,

$\left(\dfrac{z_{1}-z_{2}}{{z_{3}}-{z_{4}}}\right) + \left(\dfrac{\bar{z_{1}}-\bar{z_{2}}}{{\bar{z_{3}}}-{\bar{z_{4}}}}\right) = 0$

$\left(\dfrac{z_{1}-z_{2}}{{z_{3}}-{z_{4}}}\right) = - \left(\dfrac{\bar{z_{1}}-\bar{z_{2}}}{{\bar{z_{3}}}-{\bar{z_{4}}}}\right)$

$\dfrac{z_{1}-z_{2}}{\bar{z_{1}}-\bar{z_{2}}}$ = -   $\dfrac{z_{3}-z_{4}}{\bar{z_{3}}-\bar{z_{4}}}$

$\dfrac{z_{1}-z_{2}}{\bar{z_{1}}-\bar{z_{2}}}$ +   $\dfrac{z_{3}-z_{4}}{\bar{z_{3}}-\bar{z_{4}}} = 0$

Therefore, if ${{w}_{1}}$ and ${{w}_{2}}$ are slopes of two lines $AB$ and $CD$ respectively, then the condition for the lines to be perpendicular is ${{w}_{1}}+{{w}_{2}}=0$.

Equation of Circle in Various Forms

• The Simplest equation of a circle is $x^2+y^2=r^2$ whose centre is (0,0) and radius is r.

• The equation $(x-a)^2+(y-b)^2=r^2$ represents a circle whose centre is (a,b) and radius is r.

• The general equation of a circle is $x^2+y^2+2gx+2fy+c=0$ whose centre is (-g,-f) and radius is $\sqrt{g^2+f^2+c}$.

• If point P(a,b) and Q(c,d) are endpoints of the diameter then the equation of circle is $(x-a)(x-c)+(y-b)(y-d) = 0$

• Parametric Equation of Circle: If the circle is given by $x^2+y^2=r^2$ then $x=r cos \theta$ and $y=r sin \theta$ are parametric equations.

Circle- Equation in Complex Plane

A circle can be defined as the locus of points that are equidistant from a fixed point, i.e., centre; and the distance of the points from the centre is called the radius of the circle.

Let us consider a circle with centre at ${{z}_{0}}$ and $z$ be the locus of points on the circumference. Let $r$ be the radius of the circle as shown below in the diagram.

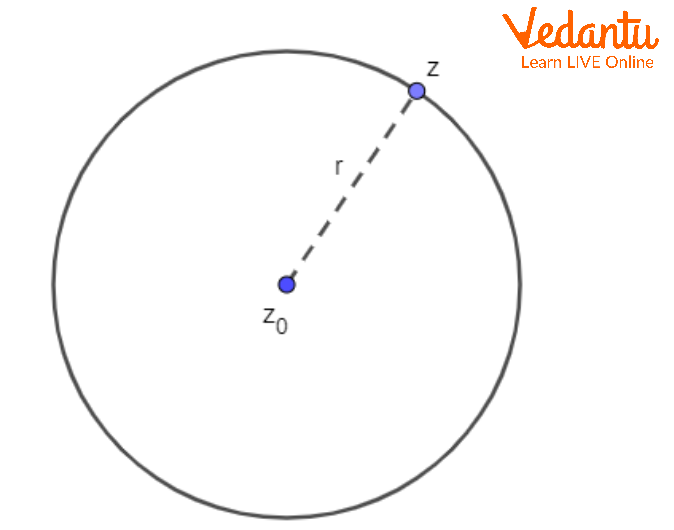
A Circle in Complex Plane

The distance between the centre, ${{z}_{0}}$ and $z$ will always be equal to the radius $r$, i.e., $\left| z-{{z}_{0}} \right|=r.$

Squaring both the sides gives 

$|z-z_{0}|^2=r^2$

$\Rightarrow (z-{{z}_{0}})(\overline{z-{{z}_{0}})}={{r}^{2}}$

$\Rightarrow z\bar{z}-z\overline{{{z}_{0}}}-\bar{z}{{z}_{0}}+|{{z}_{0}}{{|}^{2}}-{{r}^{2}}=0$

$\Rightarrow |z{{|}^{2}}+\bar{z}\alpha +z\bar{\alpha }+k=0$

where $\alpha=-z_{0}$ and $k=|z_{0}|^2-r^2$

The above equation is general equation of a circle in complex form, having centre as $-\alpha$ and radius will be $\sqrt{|z_{0}|^2-k}$.

Summary

• The general equation of a straight line in a complex plane is given as $\bar{a'}z+\bar{z}a’+c=0$  where $a’$ is a complex number and $c$ is a real number.

• The parametric form of straight line in complex plane is given as $z=t{{z}_{1}}+(1-t){{z}_{2}}$ where $t=\frac{1}{1-\lambda} \epsilon\mathbb{R}$.

• The condition for two straight lines in complex plane to be parallel is given as  $w_{1}=w_{2}$ where ${{w}_{1}}$ and ${{w}_{2}}$ are slopes of lines $AB$ and $CD$ respectively.

• The condition for two straight lines in complex plane to be perpendicular is given as $w_{1}+w_{2}=0$, where ${{w}_{1}}$ and ${{w}_{2}}$ are slopes of lines $AB$ and $CD$ respectively.

• The general equation of a circle in complex form is given as $|z{{|}^{2}}+\bar{z}\alpha +z\bar{\alpha }+k=0$ where centre of the circle is $-\alpha$ and it’s radius is $\sqrt{|z_{0}|^2-k}$.