




JEE Main 2025: What is the Formula of Area of Square?
When we talk about some plane figures, we think of their shape, region, or boundary. We compare the objects based on their size and area. We all know that we need some measures to compare them and find what. And one such measure is its area. All the objects that lie in a plane acquire some region of a flat surface. The measure of the surface enclosed by a closed figure is called its area. Here we will learn how to find area of a square, which is also important basic topic for JEE Main Exam.
Different geometrical closed shapes exist namely square, rectangle, triangle, circle, etc. In this article, we will mainly be focusing on the understanding of the area of a square with some practical examples, its calculation, units. We will discuss what is square, understanding the area of the square formula in this article. Let us start!
What is Square?
Let us first understand what a square is, the shape and structure of a square. A square is a four-sided rectangular closed figure on a plane. Square is a normal quadrilateral, which has every one of the four sides of equivalent length and each of the four points is likewise equivalent. An object to be defined in two-dimensional geometry must have been measured for length and breadth. Here, in the case of a square, its length and breadth are equal.
The points of the square are at the right-point or equivalent to 90-degrees. Likewise, the diagonals of the square approach and divide each other at 90 degrees.
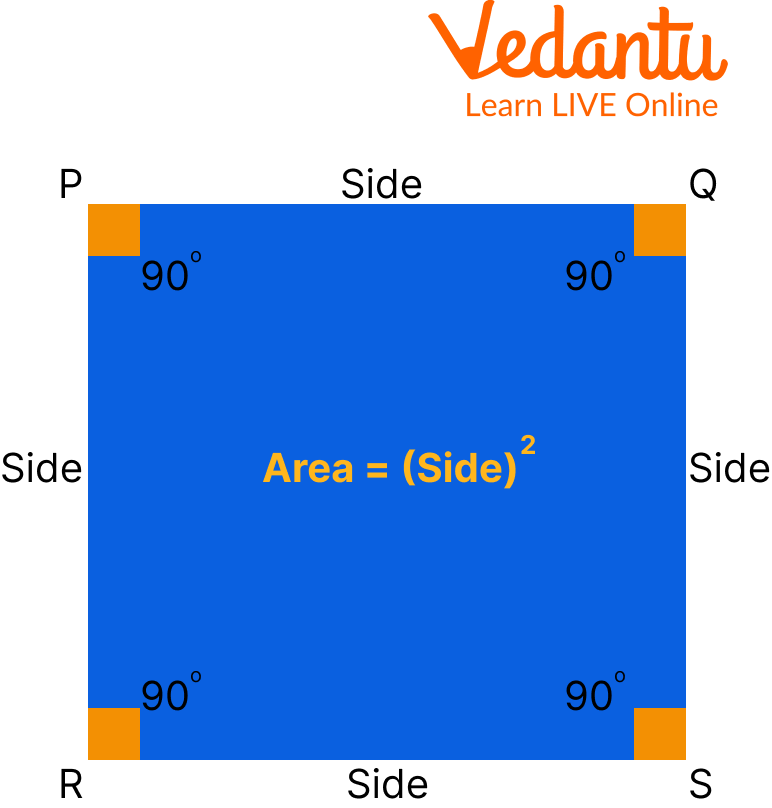
Shape of Square
A square is a four-sided polygon that has all sides equivalent in length and the proportion of the points is 90 degrees. The state of the square is, for example, assuming it is cut by a plane from the middle, then, at that point, both the parts are balanced. Every 50% of the square then, at that point, resembles a square shape with inverse sides equivalent.
What is Area?
The area is the space covered by the object. It is the region occupied by any shape. Usually, the area of a figure can be measured in a two-dimensional plane, where only the surface of the shape is considered. For example, in the case of a square, we consider only the length of its side.
In maths, the region can be characterised as the space involved by a level shape or the outer layer of an item. The space of a figure is the number of unit squares that cover the outer layer of a shut figure. The region is estimated in square units, for example, square centimetres, square feet, square inches, and so on.
The region is the space covered by the item. It is the district involved by any shape. While estimating the space of a square, we think about just the length of its side. Every one of the sides of a square is equivalent and henceforth, its region is equivalent to the square of the side.
The square of the side of the square figure gives the area, as all the sides of this shape that is a square are equal. Similarly, we can find the area of the other shapes such as rectangle, parallelogram, triangle, or any polygon, based on its sides. Only in the case of any curved object or a circle, we measure the area based on the radius or the distance of its outer line from the axis.
What is the Area of a Square?
The area of square formula in maths can be found out by the amount of space occupied within the square. Below, we will discuss the formula to calculate the area of the square. It is best known for the quadrilaterals in geometry. A square can simply be a specific case of a regular polygon, but in this case with 4 equal sides. All the facts and properties described for regular polygons can be applied to a square.
The area of a square is characterized as the number of square units expected to fill a square. As a rule, the region is characterized as the district involved inside the limit of a level article or 2d figure.
For the calculation of region, there are pre-characterized recipes for squares, square shapes, circles, triangles, and so on In this article, you will find out with regards to the space of a square.

Area of Square Formula in maths \[= a \times a = a^{2} \]
Where a is the length of the side of a square.
Arithmetically, the space of a square can be found by squaring the number addressing the proportion of the side of the square. Presently, let us utilize this equation to track down the space of a square of 7 cm. We realize that the space of a \[square = Side \times Side\].
Area of Square Formula Derivation
To better our understanding of the concept, let us take a look at the derivation of the area of square formula in maths. Let us consider a square as a rectangular object whose length is of a unit and breadth is of a unit.
The area of a square can be characterised as the district which is encased inside its limit. As we referenced, a square isn't anything a square shape with its two contiguous sides being equivalent long. Subsequently, we express region as:
\[A = L \times B \]
Where, A = Area of the square, l = Length of the object, b = Breadth of the object
Area of square formula in maths \[= l \times b \]
\[A = a \times b = a \times a = a^{2} \]
Questions To Be Solved to Find What Area of Square is Equals To
Find the area of a square plot of 8 m.
Ans: As we already have a formula for calculating the area of a square. Let us substitute the values,
Area of Square formula in maths \[= a \times a = a^{2}\]
\[A = 8^{2} \]
Area of a Square in maths \[= 64 m^2\]
A Square of 10 cm long is cut into tiny Squares of 2 cm long. Calculate the number of tiny Squares that can be created.
Ans: Since the length of the big number is in cm, hence its Area A is:
Area of Square formula\[ = a \times a = a^{2}\]
\[A = 10^{2} = 100 cm^{2} \]
Now, since the length of a tiny square is 2 cm, hence its Area is:
Area of a Square \[ = a \times b \]
\[= 2 \times 2 = 2^{2} = 4 cm^{2} \]
Therefore, the number of Squares that we can create are:
\[ \text{Number of Squares} = \frac{\text{Area of Big Square}}{\text{Area of Tiny Squares}} = \frac{100}{4} \]
Therefore, Number of Squares = 25
The area of a square-formed carrom board is \[3600 cm^{2}\]. What is the length of its side?
Ans: Area of the square carrom board \[= 3600 cm^{2} \].
We realize \[Area = side \times side = side^{2} \].
Along these lines, \[side = \sqrt{Area} = \sqrt{3600} = 60 cm \].
Along these lines, the side of the carrom board is 60 cm.
Observe the space of a square rug whose askew is 4 feet?
Ans: The space of a square when its corner to corner is given is \[\frac{D^{2}}{2}\].
Given, corner to corner d = 4 ft.
Space of the rug \[= \frac{(4 \times 4)}{2} = \frac{16}{2} = 8 square feet\].
In this manner, the space of the floor covering is 8 square feet.
Observe the space of a square rug whose inclining is 4 feet.
Ans: The Area of a square when its slanting is given is \[\frac{D^{2}}{2}\].
Given, slanting d = 4 ft.
Space of the rug \[= \frac{(4 \times 4)}{2} = \frac{16}{2} = 8 square feet\].
Accordingly, the space of the floor covering is 8 square feet.
What is the space of a square-molded pool whose one side is equivalent to 8 m?
Ans: We realise that one side of the pool is 8 m, thus,
we will utilise the equation:
\[\text{Area of a square} = side \times side = 8 \times 8 = 64 m^{2} \].
Accordingly, the space of the pool is 64 square meters.
JEE Main Maths Study and Practice Materials
Explore an array of resources in the JEE Main Maths Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Maths preparation.
JEE Main 2025 Subject-Wise Important Formulas
The JEE Main 2025 subject-wise important formulas provide a focused strategy for Chemistry, Physics, and Maths. These formulas help students prioritise revision in their preparation, ensuring better performance in the exam.
Conclusion
The area of a square is easily calculated using the formula Area = side × side or A = s², where "s" is the length of one side. This simple and straightforward formula helps in quickly determining the space inside a square, making it useful in various fields like construction, design, and geometry. By understanding this formula, you can efficiently solve problems involving squares in both academic and real-world situations.
JEE Main 2025 Subject-Wise Important Chapters
The JEE Main 2025 subject-wise important chapters provide a focused strategy for Chemistry, Physics, and Maths. These chapters help students prioritise their preparation, ensuring they cover high-weightage topics for better performance in the exam.
Check Other Important Links for JEE Main 2025
JEE 2025 needs well-structured study materials. Here are some valuable resources to help you plan your preparation and practice efficiently.
FAQs on Area of Square Formula with Examples for JEE Main 2025
1. What is the Area of Square in Geometry?
In calculation, the square is a shape with four equivalent sides. The space of a square is characterized as the number of square units that make a total square. It is determined by utilizing the space of the square equation Area = s \[\times\] s = \[s^{2}\] in square units. In other words, when we need to observe the space of a square, we think about the length of its side. Since every one of the sides of a square is equivalent, its region is the result of its different sides.
2. How to Find the Area of a Square From the Perimeter of the Square?
The space of a square can be determined assuming that the edge of the square is known.
Since the edge of a square is: P = 4 \[\times\] side,
we can track down the side of the square‘s \[= \frac{Perimeter}{4}\]. In the wake of getting the side, the pace of a square can be determined with the equation: A = s \[\times\] s.
For instance, assuming the border of a square is 32 units, we will substitute this worth in the equation:
P = 4 \[\times\] side.
32 = 4 \[\times\] side.
Thus, the side will be 8 units. Presently, we can ascertain the space of the square with 8 units. Region = s \[\times\] s = 8 \[\times\] 8 = 64 square units.
3. What is the perimeter?
Perimeter is the distance around a two-layered shape, an estimation of the distance around something; the length of the limit. It is a shut way that incorporates, encompasses, or diagrams either a two-layered shape or a one-layered length. The edge of a circle or an oval is called its perimeter.
Working out the edge has a few viable applications. A determined border is the length of fence needed to encompass a yard or nursery. The border of a wheel/circle (its circuit) portrays how far it will move in one transformation. Essentially, the measure of string twisted around a spool is identified with the spool’s border; assuming the length of the string was precise, it would rise to the edge.
3. What is the diagonal of a square?
The corner to corner of a square is a line section that joins two non-adjoining vertices. A square has two diagonals that are equivalent in length and cut up one another at right points. The properties of the diagonals of a square are as per the following:
They are equivalent in length. They are opposite bisectors of one another. A square has two diagonals and every slanting is framed by joining the contrary vertices of the square. For a more detailed explanation of any and every topic like these, use Vedantu’s free study material and grasp much more productive knowledge. The website of Vedantu has a lot more detailed explanation of various relevant topics of mathematics and other subjects, which certainly is helpful to the students.
5. What is the formula of area of square?
The formula of area of square is A = side × side, which calculates the total space enclosed within the square.
6. Can you give an area of square formula example?
Sure! If the side length of a square is 5 units, the area of the square is calculated as 5 × 5 = 25 square units.
7. Is the area of square formula applicable for all squares?
Yes, the area of square formula is valid for all squares, as they always have equal sides.
8. What units are used in the formula of area of square?
The units for the area of a square are always square units, such as square meters (m²), square centimeters (cm²), etc.
9. Can the formula of area of square be used for rectangles?
No, the formula of area of square applies only to squares. For rectangles, the area is calculated as length × breadth.
10. How to use the area of square formula in practical problems?
In practical problems, measure the side of the square, plug it into the formula A = s², and calculate the enclosed area.

















