Sum of Infinite GP - JEE Important Topic

A geometric progression is a series of numbers such that when the preceding numbers are multiplied by a fixed non-zero value, we get the succeeding number. The fixed, non-zero value that gets multiplied by the preceding value is called the common ratio. The mathematical sum of all the terms in the geometric progression, when the progression contains an infinite number of values, is known as the sum of an infinite geometric progression. 

The sum of infinite terms of a GP can be calculated. Simple formulas are used to calculate the sum of n terms in a GP series. One of the most important characteristics of a GP series is that it has an infinite number of terms, with the general ratio being smaller than and the total of such series being finite. This characteristic of the geometric progression series will be discussed in this article.

What is the Sum of Infinite GP?

A sum of a geometric series or geometric progression (GP) is an infinite series, which is mathematically expressed as $a+a r+a r^{2}+a r^{3}+\ldots \infty$. As you can see, the series gets extended up to infinity. In the mathematical expression, a is the first term of the sum of infinite GP series, and r is the common ratio such that every consecutive term gets multiplied by r. 

The derivation of the sum of infinite GP formulas is easy, but only if you understand the basics. As the name suggests, it is nothing more than adding up all the terms in a geometric progression or a GP series that extends up to infinity. Though here we mainly talk about infinite GP series, the sum of a geometrical progression can also be finite. In the case of the sum of a finite geometric progression with n terms, the formula is given as follows:

$S_{n 1}=a \dfrac{\left(1-r^{n}\right)}{(1-r)}$

Here, a is the first term of the progression and r is the common ratio.

If we need to calculate the sum of an infinite geometric series, we'll need to apply a different formula. If  -1 < r < 1 and n are both infinite, then rⁿ will tend to zero. As a result, rⁿ is 0 in the previous formula for the sum of finite geometric series, and it becomes a new formula, which is

$S_{\infty}=\dfrac{a_{r}}{1-r}$

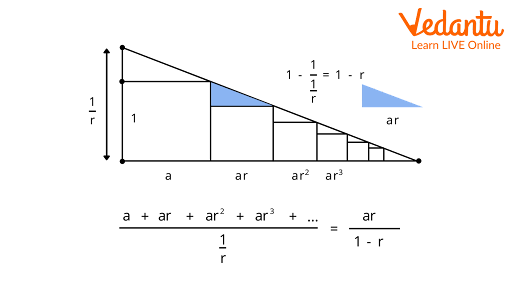
Sum of Infinite GP

Explanation and Derivation of the Formula of an Infinite GP Series

An infinite geometric series is a series of the type

$a+a r^{2}+\ldots+a r^{n}+\ldots \ldots+\infty$

Let us consider an infinite geometric progression

a = First Term, r = Common Ratio

where

 -1 < r < 1 i.e., |r| < 1

As a result, the sum of the n terms of this geometric progression is

$\begin{align} &S_{n}=\dfrac{a\left(1-r^{n}\right)}{(1-r)} \\ &S_{n}=\dfrac{a}{1-r}-\dfrac{a r^{n}}{1-r} \end{align}$ …Eq(1)

$\dfrac{a r^{n}}{1-r}=0$ as $n=\infty$ ...... Eq(2)

As a result, the total of an infinite geometric progression  is given by equation (1)

$S=\lim _{x \rightarrow 0} x_{n}=\lim _{x \rightarrow \infty}\left(\dfrac{a}{1-r}-\dfrac{a r^{2}}{1-r}\right)=\dfrac{a}{1-r}, \text { where }|r|<1$

Sum of an Infinite GP Series When |r| < 1

From the above example, it is evident that when the common ratio is less than unity, the sum of an infinite GP is a finite value. This is because as the series progresses, the value becomes smaller and smaller, and the value of the sum almost reaches 2. As the series converges to a particular point on the number line, finding out the answer to the sum becomes a lot easier.

Sum of Infinite GP Formula Derivation When |r| < 1

We consider a GP series where the first term is ‘a’ and the common ratio is $r(|r|<1)$.

Therefore, 

Multiplying both sides of the equation with the common ratio we get, $Sr=a r+a r^{2}+a r^{3}+\ldots(2)$  

Subtracting equation (2) from equation (1), we see that

$S-S r=a$. 

Or, S_(1-r) = a

Therefore, the sum of an infinite geometric series formula when, |r| < 1 is $S=\dfrac{a}{(1-r)}$.

In this case, the series converges. 

Sum of an Infinite GP Series When |r| > 1

The terms of the GP are nearly equivalent to zero after a finite number of repetitions. We get a finite number after the sum of an infinite GP whenever |r| > 1. When the value of r is more than or equal to one, we obtain a succession of numbers like 3,6,9,12,15......that keep increasing and eventually form a very large number when |r| is greater than or equal to one. A divergent series is the one in which the value of r is larger than or equal to one.

$S_{\infty}=\pm \infty, \text { when }|r| \geq 1$

Sum of Infinite GP Examples

To understand the idea of the sum of an infinite GP series, let us consider the following examples.

Example 1: Find the solution to the following sum. 

Solution: Given the infinite GP series is as follows

$S=1-\dfrac{1}{2}+\dfrac{1}{4}-\dfrac{1}{8}$

Here, the first term of the series is a = 1 and the common ratio $r=-\dfrac{1}{2}$ 

Now, since -1 < r < 1, therefore the series converges. 

Thus, the required sum of the infinite GP series is as follows:

$S=\dfrac{a}{1-r}$

$S=\dfrac{1}{1-\left(-\dfrac{1}{2}\right)}=\dfrac{1}{\left(\dfrac{3}{2}\right)}=\dfrac{2}{3}$

The sum of the series is $\dfrac{2}{3}$.

Example 2: Calculate the sum of the series.

$1, \dfrac{1}{3}, \dfrac{1}{9}, \dfrac{1}{27},.........$

Solution: Given, a = 1, and r = $\dfrac{1}{3}$

We Know that -1 < r < 1 ,

\[ S_\infty=\dfrac{a_r}{1-r} \]

$\begin{align} &S_{\infty}=\dfrac{1}{1-\dfrac{1}{3}} \\ &S_{\infty}=\dfrac{1}{\dfrac{2}{3}} \\ &S_{\infty}=\dfrac{3}{2} \end{align}$

Sum of the series is $\dfrac{3}{2}$.

Conclusion

If a sequence has a finite number of terms, it is finite; otherwise, it is infinite. Geometric Progression (GP) is a sort of sequence in which each successive phrase is obtained by demonstrating the utility of infinite functional series in the given contribution like power or Taylor. Those are useful for routine physics computations as well as the development of new physics findings and hypotheses.