Displacement Current and Maxwell’s Equations - JEE Important Topic

There are four Maxwell’s equations. In electromagnetic theory, the very first thing you learn is Maxwell’s equations. The starting of those four equations revolutionised the entire subject by unifying two separate genres of study in one. 

We all know that in electrostatics, EM fields due to stationary charges, i.e., electric and magnetic fields are mutually independent. Magnetostatics deal with charges in motion with uniform velocity, i.e., constitute steady currents. However, when the electric field varies with time, necessarily, it will be accompanied by a varying magnetic field. So, the foundation study laid out for electromagnetic theory was through Maxwell’s equations. 

Equations Governing Electromagnetism: Maxwell’s Equation

The four equations mainly summarise four very impactful laws in the entire electromagnetic theory. Let us dive into the laws that lay the foundation of the equations.

Gauss’s Law

This law is the sensational relation of electric field and charge explained meticulously in electrostatics. The statement is that the complete electric flux magnitude through a symmetric surface is equal to the total enclosed charge in that surface divided by the material permittivity.

Mathematically,

$\nabla .\vec{E}=\dfrac{\rho }{{{\varepsilon }_{0}}}$      (Differential form)

$\oint{\vec{E}\cdot d\vec{a}}=\dfrac{{{Q}_{enc}}}{{{\varepsilon }_{0}}}$    (Integral form)

Magnetic Monopoles

The magnetic equivalence of Gauss’s Law is the second equation in Maxwell’s equations. There is no existence of magnetic monopoles; so,

$\nabla .\vec{B}=0\ \ \ $ (Differential form)

$\oint{\vec{B}\cdot d\vec{a}}=0$  (Integral form)

Faraday’s Law

This law basically states that there is an emf induced in a coil or a circuit when there is a magnetic field varying with time, or there is a rate of change in magnetic flux through the coil. The direction of the induced emf is given by Lenz's law which suggests that the induced emf always opposes the cause of its formation in the first place.

Mathematically,

$\nabla \times \vec{E}=-\dfrac{\delta \vec{B}}{\delta t}$   (Differential form)

$\oint{\vec{E}\cdot d\vec{l}=-\int{\dfrac{\delta \vec{B}}{\delta t}\cdot d\vec{a}}}$   (Integral form)

Ampere’s Circuital Law 

This law relates steady currents running on a surface or circuit with the magnetic field produced by the current itself essentially wrapped around the circuit.

Mathematically the ampere maxwell equation or maxwell fourth equation is:

$\nabla \times \vec{B}={{\mu }_{0}}\vec{J}$   (Differential form)

$\oint{\vec{B}\cdot d\vec{l}}={{\mu }_{0}}{{I}_{enc}}$   (Integral form)

So, collectively the four equations can be written as,

The maxwell equation derivation results:

Insufficiency of Ampere’s Law

From the third Maxwell’s equation, which is derived from Faraday’s law, we concluded that when there is change in magnetic field or flux with time then an electric field is induced in the closed loop. The question coined by Maxwell at that time was if the reverse phenomenon is also possible or true. Whether the change in electric field will cause a magnetic field induction was the idea.

He showed that when there is a change in electric flux, a magnetic field is induced in the loop known as Maxwell's law of induction. An experimental setup was described to support the idea. A charging of parallel plate capacitor as shown below was used.

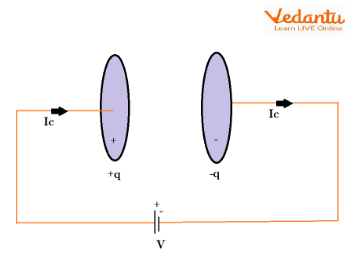
Parallel Plate Capacitors Charging

Now, mathematically, the Ampere’s circuital law for steady currents is

$\nabla \times \vec{B}={{\mu }_{0}}\vec{J}$

Now applying divergence on both sides, we get

$\nabla \cdot \left( \nabla \times \vec{B} \right)={{\mu }_{0}}\left( \nabla \cdot \vec{J} \right)=0\ $, since the divergence of a curl is zero.

Also, we know from the continuity relation of current density,

$\nabla \cdot J=-\dfrac{\partial {{\rho }_{v}}}{\partial t}\ne 0$

Here, the current density is not zero because the charge density varying with time is not zero, i.e., the charge density is not constant. This is because while charging, the charge gets piled up in between the plates and follows a relation that varies with time. So, the Ampere’s law is showing clear discrepancy and is not sufficient for explaining time varying fields.

What is Displacement Current?

To rectify the discrepancy in Ampere’s Circuital Law explained above, Maxwell adjusted the main relation with an additional term. This made the relation or law wholesome with both static and time-varying parts present to play their part. Let us dive into the mathematics part.

Determination of displacement current formula:

So, at first we take the magnetic field intensity without the magnetisation,

$B=\mu H$ 

Now, we add the additional term which we denote as J_d,

$\nabla \times H=J+{{J}_{d}}$ 

Performing the same divergence action,

$\nabla \cdot \left( \nabla \times H \right)=0=\nabla \cdot J+\nabla \cdot {{J}_{d}}$ 

Our job now is to define the term ${{J}_{d}}$. 

$\nabla \cdot {{J}_{d}}=-\nabla \cdot J$

$=\dfrac{\partial {{\rho }_{v}}}{\partial t} $

Now, we know from Gauss’s law, $\nabla \cdot D=\rho $, where D is the displacement vector and $\rho $ is the charge density. Therefore, our equation becomes,

$\dfrac{\partial {{\rho }_{v}}}{\partial t}=\dfrac{\partial }{\partial t}\left( \nabla \cdot D \right)$

$=\nabla \cdot \dfrac{\partial D}{\partial t} $ 

So, $\nabla \cdot {{J}_{d}}=\nabla \cdot \dfrac{\partial D}{\partial t}$

Therefore, ${{J}_{d}}=\dfrac{\partial D}{\partial t}$ 

So, now we have successfully defined the term and this is known as the displacement current density formula. This is only active when the time varying fields are required. For a constant displacement vector, i.e., a constant charge density the displacement current density vanishes.

So, the Maxwell’s equations explained

Significance of Displacement Current

Displacement current is vital to explain the propagation of EM radiation like light waves, microwaves or radio waves through sheer vacuum or empty space. The fact that the analogy of electric fields can be applied to magnetic fields is amazing and shows how unified two theories can be. To prove that it is indeed possible to obtain magnetic fields by changing the electric field with time was the main idea at the time of its conception. 

Now that it has been proven, the symmetry in nature and the laws that govern it is functional and amazing to witness. The displacement current concept is entirely based on elements discussed under electrostatics and the interconnection between the two branches is what electromagnetism is all about.

Summary

Static charges give rise to electrostatics. Steady currents give rise to magnetostatics. Time-varying currents give rise to electromagnetism. Maxwell’s equations unified the fields of Electrostatics and Magnetostatics. Maxwell’s equations are derived from Gauss’s Law, Faraday’s Law, Non-existence of magnetic monopoles, and Ampere’s Circuital Law. 

The Amperes’ Circuital Law worked for steady currents but failed when time varying fields were introduced. Maxwell made the crucial correction and added displacement current density to the normal current density to rectify. This correction made the law include both time-dependent and time-independent factors. The displacement current density is given as, ${{J}_{d}}=\dfrac{\partial D}{\partial t}$