




Rotational and Circular Motion
Rotational motion refers to any body that is moving or spinning around a circular path. It can also be referred to as circular motion but there is a fundamental difference between rotational motion and circular motion. While circular motion can be around an arc or a part of a circle, it is not necessarily around an axis of rotation. Rotational motion on the other hand is always around an axis of rotation and a body moving around the fixed axis constitutes circular motion.
Rotation or rotational motion refers to the movement of a body about a fixed point. This fixed point forms the centre of the rotation when a line perpendicular to the plane in which the body is travelling passes through it. Examples of rotational motion include the motion of a wheel about an axle of the bicycle or a car. Now there might be cases where the object is following a translational as well as a rotational motion. This is where the dynamics of rotational motion about a fixed axis comes in. Further in this article, we will learn what rotational motion is about the fixed axis.
An Introduction to Rotational Dynamics
A rigid body is a body in which the distance between any two points remains constant. It can also be said to be a body that does not change its shape. Now when a body is rotating about any axis, there is a point that has zero velocity and this is the point about which the rotational motion takes place. This point does not necessarily have to be on the body, it can also be away from the body. An imaginary line that passes through this line perpendicular to the plane of rotation is known as the axis of rotation. In rotational motion, the analogue of force is torque. It is the torque that brings about a change in the angular velocity of the particles.
We only take into account the components of the applied torques to the object that are along this axis since only these parts cause the body to rotate. The axis of rotation for the object will typically tend to turn from its position due to the torque's perpendicular component. These perpendicular components get cancelled due to the forces of constraint on the axis of rotation and so we could say that these components have no effect on the body and we don’t consider them during our analysis. We must only take into account the forces that exist in planes perpendicular to the axis for any rigid body rotating along a fixed axis.
We’ll now take a look at the important concepts in rotational dynamics.
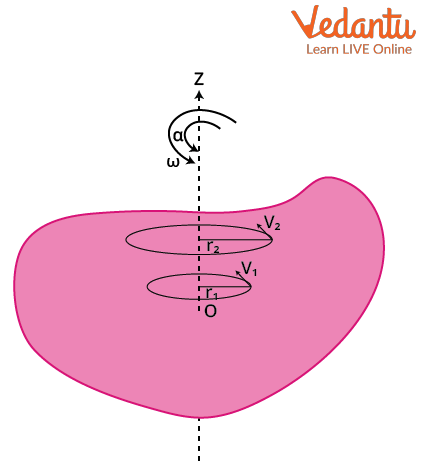
A Rigid Body Rotating about a Fixed Axis
Important Quantities in Rotational Motion
Moment of Inertia
Moment of inertia is sometimes known as the rotational mass of the body. It is the rotational analogue of mass in rotational motion. The object's resistance to a change in rotation is measured by the moment of inertia. For any rigid body, if we consider the distance of the particles from the axis of rotation as ri and the mass to be mi, then the moment of inertia is given as
As it is clear from the equation, the moment of inertia is dependent on the mass of the body.
Torque
Torque is the rotational analogue of force in rotational motion. Just as force brings about an acceleration or change in velocity in translational motion, torque brings about a change in the angular velocity of the body. Torque can be defined as the twisting result of a force F given to a rotating object at a distance r from its axis of rotation. The expression for the torque
Angular Momentum
As might be clear from the name, angular momentum is the angular or the rotational analogue of momentum in rotational motion. Just as linear momentum is conserved in translational motion when there is no external force, similarly angular momentum is conserved when there is no external torque. The expression for the angular momentum L of a particle at distance r from the axis of rotation and having linear momentum p is given as
We can also write this in terms of the moment of inertia I and angular velocity
These were the fundamental quantities in rotational motion. We’ll now take a look at the relationship between torque, moment of inertia, and angular acceleration.
Torque, Moment of Inertia, and Angular Acceleration
We have had a look at what torque and moment of inertia mean, let us now understand about angular acceleration. Angular acceleration is basically just the rotational analogue of acceleration. Angular acceleration is responsible for bringing about a change in the angular velocity
Here, r is the distance from the axis of rotation.
Now, from Newton's second law of motion, we know that
Now we also know that
Substituting this, we get
We know that
mr2=I
I is the moment of inertia and substituting this, we get
This is the relationship between torque, moment of inertia, and angular momentum.
Work-Energy Principle for Rotational Motion
We know that the work energy principle states that the work done is equal to the change in kinetic energy. We also know that the work done is equal to the dot product of force and displacement. So, work depends on the force. The rotational analogue of force is torque, so we could say that the work energy principle in rotational dynamics is based on torque. The work done is equal to the dot product of the torque and the angular displacement. We can write it as
Here, a constant torque is acting. If it was a variable torque, then we would need to integrate the expression of work for torque.
Now we know that
So, we can write
The angular acceleration can be written as the time derivative of angular velocity.
We know that the angular velocity can be written as the time derivative of the angular displacement.
So finally we can write,
If we consider
So, we have proved that the work done by torque is equal to the change in rotational kinetic energy of the body. This is the work energy principle for rotational dynamics.
Conclusion
Rotational motion is the motion of any body about a fixed axis. Most of the time rotational motion and circular motion are used interchangeably but they are different. Circular motion is not around any axis, it is just the motion in a circle. Circular motion is a part of rotational motion. Moment of inertia, torque, and angular momentum are the rotational analogues of mass, force, and momentum in rotational motion. The relationship between the torque, moment of inertia, and the angular acceleration is given as,
This expression is also known as Newton's second law of motion in rotational motion. The work-energy theorem is also applicable to rotational motion and it states that the work done by any torque is equal to the change in rotational kinetic energy of the body.






FAQs on Dynamics of Rotational Motion about a Fixed Axis for JEE
1. Is rotational motion the same as rotational dynamics?
When an object moves around in a circular path around any axis, the motion is known as rotational motion. Rotational dynamics comes in when we study the cause of rotational motion. Consequently, the torque is a force that is investigated within rotational dynamics. The item is handled differently in translational motion than it is in rotational motion, when it is viewed as a particle. The analysis of the torque responsible for the angular acceleration of objects forms the basis of rotational dynamics.
2. What is the weightage of dynamics of rotational motion about a fixed axis in JEE Main?
Dynamics of rotational motion about a fixed axis comes under the chapter ‘Rotational Mechanics’, and this is an extremely important topic to study from an exam point of view. The concepts from this topic including torque are also used in dipoles in electrostatics, gyroscopes, etc. In rotational mechanics, torque is an integral concept, and most problems involve torque. Rotational mechanics has about 3.3% of the weightage in JEE main, which means that almost one question is asked on this topic every year.

































