EMF and Internal Resistance of a Cell - JEE Important Topic

A simple cell or battery is defined as a device that is used to maintain a Potential difference that forces the charge/electron to move in the circuit. It provides electrical energy to all the components of a circuit. The EMF or Electromotive Force of a cell is defined as the potential difference between the electrodes of the cell when no current flows through the circuit. 

As soon as the current is allowed to flow in the circuit, the potential difference between the electrodes of the cell is dropped. This occurs because the material that the cell is made up of has a resistance of its own. And this resistance is known as the Internal Resistance of a cell. In other words, internal resistance is defined as the obstruction offered to the path of current due to the material of electrolyte of the cell.  The potential difference across the two terminals of the cell when the current is drawn from the cell is known as Terminal Voltage.

Electromotive Force as a Function of its Internal Resistance

The EMF of a cell is the work done by the cell to move the charge across two terminals of a circuit. It can be also written that ‘EMF of a cell is the work done per unit charge’, i.e., $\varepsilon =\frac{W}{q}$

If $I$ is the current flowing in a electric circuit, $R$ is the resistance of the load in the circuit, and $r$ is the internal resistance of the cell, then EMF is also given as 

$\varepsilon =I\left( R+r \right)$

If we replace $IR$ with $V$ in the above equation, where $V$ is the Terminal Voltage, then it can be written as 

$ \text{     }\varepsilon =V+Ir $

$\Rightarrow V=\varepsilon -Ir $

The above equation gives the formula to determine the Terminal Voltage across a circuit, provided the current flowing in the circuit, EMF, and internal resistance of the cell are known.

We can derive the above equation using the following circuit.

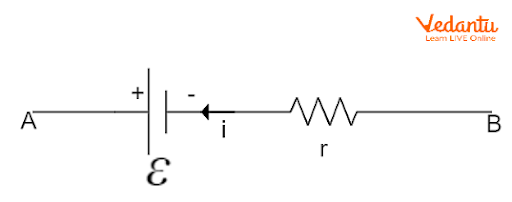
A Simple Electric Circuit

Let the potential at point $B$ be ${{V}_{B}}$ and that at point $A$ be ${{V}_{A}}$. The current in the circuit flows from point $B$ to point $A$. When the current is passing through the internal resistance $r$, a potential drop of $ir$ is observed. Again, while passing through the cell, a potential gain equal to the Emf of the cell is observed. Then, the resultant potential at the point A is observed.

Therefore, we have the equation

${{V}_{B}}-ir+\varepsilon ={{V}_{A}}$

The above equation can be written as 

 ${{V}_{A}}-{{V}_{B}}=\varepsilon -ir$

The difference of the potential across the two terminals of a circuit is termed as Terminal Voltage, denoted by $V$, so we have ${{V}_{A}}-{{V}_{B}}=V$. Then, the above equation becomes

$V=\varepsilon -ir$            ….. (1)

Now, since V is the potential difference in the external circuit, therefore, it can be written as 

$V=iR$                ….. (2)

where $R$ is the external resistance. 

Then, using equations (1) and (2), we get 

$\varepsilon -ir=iR $

$ \Rightarrow \varepsilon =i\left( r+R \right) $

$\Rightarrow i=\frac{\varepsilon }{r+R} $

Potentiometer - Measurement of EMF and Internal Resistance

Potentiometer is a device that is used to measure the potential difference. Its main uses are listed below.

1. To measure the Emf of a Cell

2. To compare Emf of two cells

3. To determine the internal resistance of cell

Construction - The potentiometer consists of a long wire of a few metres, say the length is $'I'$ of uniform cross-section area, joined with a battery in such a manner that the potential drop in the wire is $V$. This is shown in the below diagram. Now, the potential gradient, $K$ of the wire will be $\frac{V}{l'}$, that is constant throughout the wire, i.e., 

$K=\frac{V}{l}$

How to Calculate EMF of the cell

Now, we discuss the method to find the Emf of the cell

The circuit diagram is given below.

Complete Circuit Diagram with Galvanometer

In the above circuit, we firstly find the null point, i.e., the point where the Galvanometer shows zero deflection. Let $C$ be the point at which the Galvanometer shows no deflection. Since the Galvanometer shows zero deflection, that means that no current flows in the internal circuit and the potential difference across XY is same as that across $AC$. 

The potential difference across $AC$ will be equal to potential gradient multiplied by the length of $AC$, say $x$, i.e., 

${{V}_{AC}}=\frac{V}{l}x$

Since, no current flows across $XY$, therefore, the potential difference across $XY$ will be equal to the Emf $'\varepsilon '$ of the cell. Therefore, we have 

$\frac{V}{l}x=\varepsilon $

Therefore, the formula to measure Emf of cell is given as 

$\varepsilon =\frac{V}{l}x$

Now, we discuss the method to measure the internal resistance of the cell.

The circuit for the measurement of internal resistance is given below. Here, a known resistance, $R$ and a key $K$ is connected across the cell.

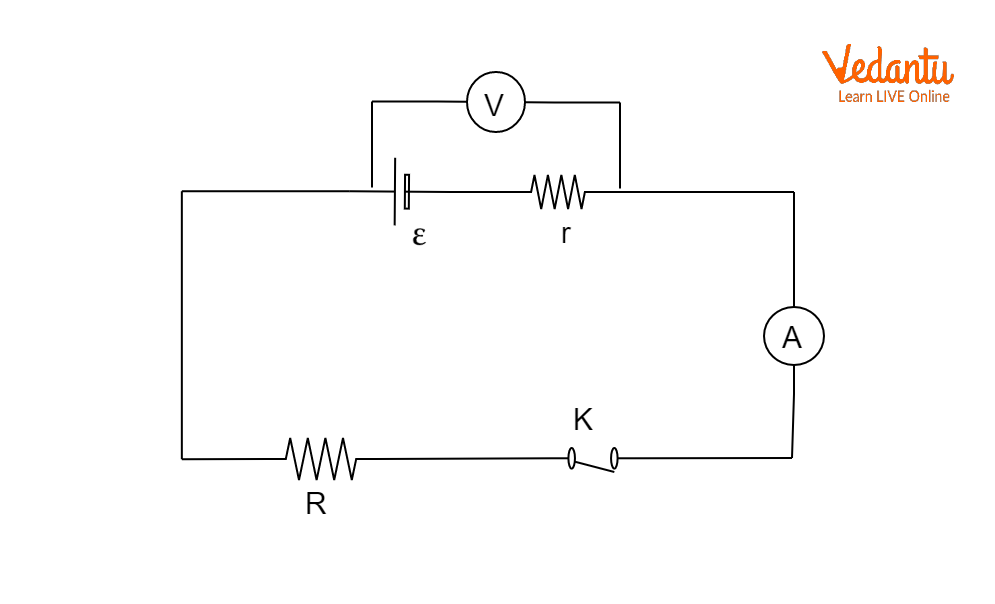
Internal Resistance of Cell

If $K$ is open and null point is obtained at a length ${{l}_{1}}$,  then

$\frac{V}{l}{{l}_{1}}=\varepsilon $           ….. (3)

When the key is closed, let’s say the null point is obtained at length ${{l}_{2}}$, then current $'i'$ flows in the internal circuit. Then, the current is given by 

$i=\frac{\varepsilon }{R+r}$

The potential drops as the current flows through the internal circuit and the length ${{l}_{2}}$ corresponds to the potential difference $\varepsilon -ir$. Therefore, we have

$\frac{V}{l}{{l}_{2}}=\varepsilon -ir,$ where $i=\frac{\varepsilon }{E+r}.$ 

Thus, we have 

$\frac{V}{l}{{l}_{2}}=\varepsilon -\frac{\varepsilon }{R+r}r$

$\Rightarrow \frac{V}{l}{{l}_{2}}=\frac{\varepsilon R}{R+r}$          ….. (4)

Dividing equation (3) by (4) gives

$ \frac{\varepsilon }{\frac{\varepsilon R}{R+r}}=\frac{{{l}_{1}}}{{{l}_{2}}} $

$\Rightarrow \frac{R+r}{R}=\frac{{{l}_{1}}}{{{l}_{2}}}$

$ \Rightarrow 1+\frac{r}{R}=\frac{{{l}_{1}}}{{{l}_{2}}} $

$\Rightarrow \frac{r}{R}=\frac{{{l}_{1}}}{{{l}_{2}}}-1 $

$\Rightarrow r=R\left( \frac{{{l}_{1}}}{{{l}_{2}}}-1 \right)$

This is the formula to measure the internal resistance of a cell using a potentiometer.

Conclusion

The EMF of a cell is defined as the potential difference between the electrodes of the cell when no current flows through the circuit. The internal resistance is the obstruction offered to the path of current due to the material of electrolyte of the cell. A Potentiometer can be used to measure the Emf and internal resistance of a cell.