Concepts of Properties of Solids and Liquids for JEE Main Physics
The chapter Properties of solids and liquids is one of the most important chapters for JEE Main. It mainly deals with the behaviour of solids under stress and liquids at rest and motion.
Related to the properties of solid, we learn about types of stresses and strain followed by the types of modulus of elasticity. The stress-strain curve of a material and Hooke's law is explained in detail in this chapter. Thermal stress and work done in stretching a wire are also discussed in this chapter in detail.
In properties of liquid, Pascal’s law and its applications are studied along with Archimedes's principles and numerical problems related to it. Bernoulli's theorem and its application and problems are very important topics in this chapter. Viscosity and surface tension is also explained in detail in this chapter. We have seen some conversion of solid to liquid examples and also liquid to solid in lower grades. A solid to liquid example of its conversion is the melting of ice.
Let us see what are solids and liquids properties and the important concepts and formulas needed for JEE Main in this chapter properties of solids and liquids with solved examples.
JEE Main Physics Chapters 2025
Important Topics of Properties of Solids and Liquids Chapter
Elasticity of solids and its elastic behaviour
Young’s modulus
Bulk modulus
Rigidity modulus
Stress-strain curve
Pascal’s Law
Mercurial Barometer
Archimedes Principle
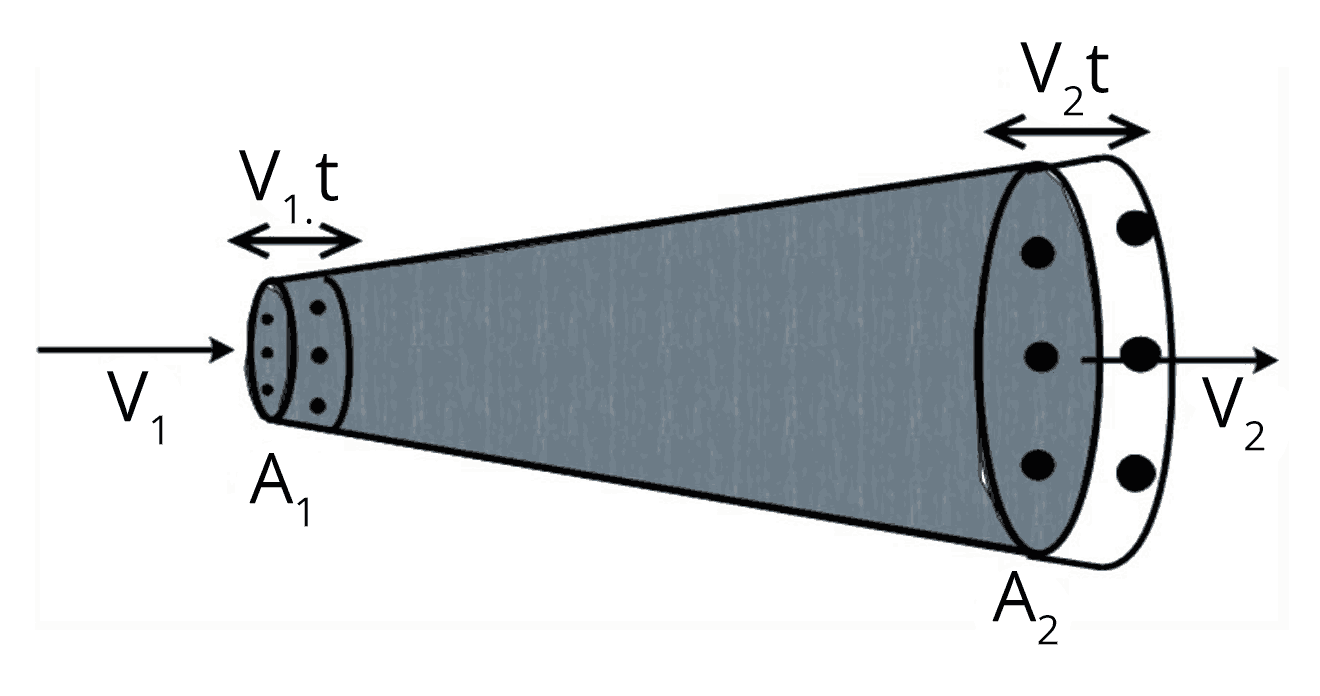
Bernoulli’s Therorem
Application of Bernoulli’s theorem
Stoke’s Law
Surface tension and surface energy
Properties of Solids and Liquids Important Concept for JEE Main
Application of Surface Tension
Raindrops: Raindrops are spherical because of surface tension. This helps the raindrops to fall faster through the air.
Bubbles: Bubbles are spherical because of surface tension. This also helps the bubbles to float in the air.
Plants: Plants absorb water from the soil through their roots. The water is transported up the stem and leaves through capillary rise.
Wicks: The wicks in candles and pens work by capillary rise. The wick draws the wax or ink up from the reservoir and to the tip of the wick.
Insects: Some insects, such as water striders, can walk on the surface of water because of surface tension.
Cleaning: Surface tension is used in some cleaning products to help them to remove dirt and grease.
Heat, Temperature, and Thermal Expansion
Heat and Temperature:
Heat is the transfer of thermal energy, while temperature is a measure of the average kinetic energy of particles in a substance. Understanding the relationship between heat and temperature is fundamental to thermodynamics.
Thermal Expansion:
Thermal expansion is the property of matter by which it expands or contracts when exposed to changes in temperature. This phenomenon has practical implications in various industries, such as construction and engineering.
Specific Heat Capacity and Calorimetry
Specific heat capacity is the amount of heat required to raise the temperature of a unit mass of a substance by one degree. Calorimetry is the science of measuring heat changes in chemical processes and has applications in various fields, including chemistry and food science.
Change of State and Latent Heat:
Change of State:
A change of state is a physical change in which a substance changes from one phase to another. The three most common phases of matter are solid, liquid, and gas. Other phases, such as plasma and Bose-Einstein condensates, also exist.
Latent Heat:
Latent heat is the energy that is absorbed or released during a change of state. It is the energy that is required to break the intermolecular bonds in a solid or liquid to form a gas, or to form the intermolecular bonds in a gas or liquid to form a solid.
Types of Latent Heat:
Latent heat of fusion is the energy that is absorbed or released when a substance changes from solid to liquid or vice versa. For example, the latent heat of fusion of ice is 334 J/g. This means that it takes 334 J of energy to melt 1 gram of ice at its melting point.
Latent heat of vaporization is the energy that is absorbed or released when a substance changes from liquid to gas or vice versa. For example, the latent heat of vaporization of water is 2260 J/g. This means that it takes 2260 J of energy to vaporize 1 gram of water at its boiling point.
Applications of Latent Heat:
Refrigeration and air conditioning: Latent heat of vaporization is used to absorb heat from the environment in refrigerators and air conditioners.
Cooking: Latent heat of vaporization is used to cook food in steamers and pressure cookers.
Power generation: Latent heat of vaporization is used to generate steam in power plants.
List of Important Formulas for Properties of Solids and Liquids Chapter
JEE Main Properties of Solids and Liquids Solved Examples
When a load of 5 kg is hung on a wire, then the extension of 3 m takes place. The work done will be,
Ans:
The mass of the load hung on the wire, m = 5 kg
The force acting on the wire is calculated as ,
We can calculate the work done in extending the wire by using the formula given below,
Key Point: The work done in extending the wire is half the product of the force acting on the wire and the extension of the wire.
In a capillary tube, water rises by 1.2 mm. The height of water will rise in another capillary tube having half the radius of the first will be ____.
Ans:
The formula to calculate the capillary rise of first tube is given by,
The capillary rise of the water in the second tube is given by,
Divide the equation (2) by equation (1) to calculate the height of capillary rise in second tube.
Key Point: The surface tension and the angle of contact in both tubes are equal since the same liquid is used in both tubes.
Previous Year Questions from JEE Exam
A hydraulic press can lift 100 kg when a mass ‘m’ is placed on the smaller piston. It can lift _______ kg when the diameter of the larger piston is increased by 4 times and that of the smaller piston is decreased by 4 times keeping the same mass ‘m’ on the smaller piston. (JEE 2021)
(Image will be uploaded soon)
Ans:
Applying Pascal’s law, the pressure acting on smaller piston due to mass m is equal to the pressure acting on the larger piston.
Let A1 and A2 be the area of the smaller piston and larger piston respectively.
Now, let M be the mass that hydraulic press can lift after changing the area of cross section of both pistons.
Again applying pascal’s law,
Using equation (1) and equation (2), we can calculate the value of M.
Therefore, the new mass it can lift is 25600 kg
Trick: The pressure acting on both the piston are equal according to the Pascal’s law.
A uniform metallic wire is elongated by 0.04 m when subjected to a linear force F. The elongation, if its length and diameter is doubled and subjected to the same force will be ________ cm. (JEE 2021)
Ans:
(Image will be uploaded soon)
The formula to calculate the young’s modulus of the wire is given by,
When the diameter is doubled, the area of cross section of the wire becomes four times initial area of cross section.
When the same force is applied after the length and diameter is doubled, then formula for young’s modulus is given by,
Using equation (1) and (2),
Therefore, the new extension will be 2 cm.
Trick: Young’s modulus deos not depend on the dimensions on the wire and only depends onthe material.
Practice Questions
An iceberg of density 900 kg/m3 is floating in water of density 1000 kg/m3. The percentage of the volume of ice cube outside the water is ? (Ans: 10 %)
A wind with speed 40 m/s blows parallel to the roof of a house. The area of the roof is 250 m2. Assuming that the pressure inside the house is atmospheric pressure, the force exerted by the wind on the roof will be (Ans: 2.4✕105 N)
JEE Main Physics Properties of Solids and Liquids Study Materials
Here, you'll find a comprehensive collection of study resources for Properties of Solids and Liquids designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Properties of Solids and Liquids and enhance your preparation for this challenging exam.
JEE Main Physics Study and Practice Materials
Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.
Conclusion
In this article, we dive into the world of Solids and Liquids, unraveling their fascinating properties. We'll explore the characteristics that make solids and liquids unique, like their ability to flow or maintain a fixed shape. You can grasp the concepts easily with our clear explanations and find solutions to common questions. All the information you need is right here, from understanding the basics to tackling exam questions. Plus, our PDF resources are free for you to download. This will undoubtedly boost your confidence for any upcoming exams!
Properties of Solids and Liquids Chapter - Physics JEE Main

 Share
ShareFAQs on Properties of Solids and Liquids Chapter - Physics JEE Main
1. How many questions are asked from properties os solids and liquids in JEE?
About 2-3 questions from this chapter are asked in JEE exam every year which corresponds to around 8-12 marks in the JEE Main exam.
2. Is the chapter properties of solids and liquids a tough chapter?
As far as teh JEE main exam is concerned, properties of solids and liquids is a moderate difficult chapter. But it is a vast chapte which consists of more theories and concepts and related formulas. But with proper learning and practices, all the concepts in this chapter can be learned without any difficulty.
3. How to get a good score in JEE Main to get into the best NITs?
To get into best NITs in the nation, we have to crack the JEE Main exam with good scores. Even though JEE Main is a very competitive exam, we can score good marks if we understand the concepts in depth and pratice lots of questions and finally doing all the previous year question papers without any fail.




















 Watch Video
Watch Video