Question

Same spring is attached with 2kg, 3kg and 1 kg blocks in three different cases as shown in figure. If x₁, x₂, x₃ be the extension in the three cases then,


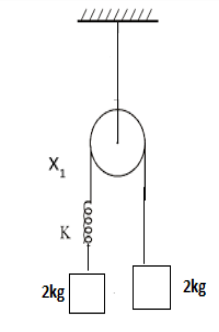
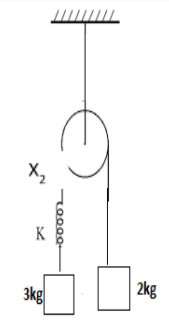
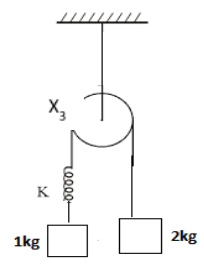
A) x₁=0, x₃ >x₂
B) x₁>x₂>x₃
C) x₃>x₂>x₁
D) x₂>x₁>x₃

Answer 1

Hint: Above problem is based on connected motion concept with the presence of second law of motion (Newton’s law of motion). In the connected system tension (force applied upwards due to the presence of pulley) is given as:
$T = \dfrac{{2{m_1}{m_2}g}}{{{m_1} + {m_2}}}$
Forces acting on a body balance each other, the force which is greater in magnitude exists in the system.
Like in the question pulley will move down if the downward force (mg) will be greater than tension.
Using the similar concept we will solve the problem.

Complete step by step solution:
We have ${x_1},{x_2},{x_3}$ proportional to tension T of the pulley:
So, we will find the tension T of the springs in each three cases by using the formula for tension of the connected system of motion.
$
\Rightarrow {T_1} = \dfrac{{2{m_1}{m_2}g}}{{{m_1} + {m_2}}} \\
\Rightarrow {T_1} = \dfrac{{2 \times 2 \times 2g}}{{2 + 2}} \\
\Rightarrow {T_1} = 2g \\
$
We have substituted the value of masses in the formula given and calculated T₁.
Now, we will calculate tension for second diagram
$
\Rightarrow {T_2} = \dfrac{{2 \times 3 \times 2g}}{{3 + 2}} \\
\Rightarrow {T_2} = \dfrac{{12g}}{5} = 2.4g \\
$ (Tension calculated for second diagram)
Now, we will move on to the third figure:
$
\Rightarrow {T_3} = \frac{{2 \times 1 \times 2g}}{{2 + 1}} \\
\Rightarrow {T_3} = \frac{{4g}}{3} = 1.66g \\
$(Tension in figure 3 is calculated)
By observation we got;
${T_2} > {T_1} > {T_3}$
As $T \propto x$
Therefore, we can write as:
${x_2} > {x_1} > {x_3}$

Option: (D) is correct.

Note: We have a number of daily life examples where we are observing Newton’s second law of motion such as, hitting the golf ball, cricket ball, football with a force the more we exert force the more we will observe the reaction. As in our question above force having higher magnitude was existing in the system.