Question

\[{T_1}\] and \[{T_2}\] in the given figure are

(A) 28 N, 48 N
(B) 48 N, 28 N
(C) 96 N, 56 N
(D) 56 N, 96 N

Answer 1

Hint: We need to identify the force acting on each block. Perform a Newton’s second law analysis on each of the blocks separately. The acceleration of each object is equal to the acceleration of the system.
Formula used: In this solution we will be using the following formulae;
\[{F_{NET}} = ma\] where \[{F_{NET}}\] is the net force acting on a body, \[m\] is the mass of the body, and \[a\] is the acceleration of the body.

Complete Step-by-Step Answer:
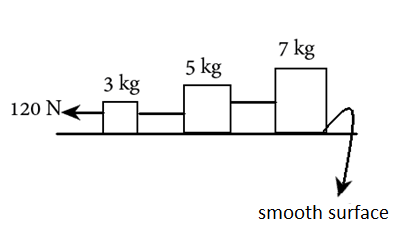
A particular amount of force is acting upon a chain of objects, the tensions in the cords are asked to be determined. To do this, we must analyse the forces on each block independently and link them with the acceleration. The acceleration of the system is the acceleration of the blocks, hence, they are equal.
Newton’s second law can be written as
\[{F_{NET}} = ma\] where \[{F_{NET}}\] is the net force acting on a body, \[m\] is the mass of the body, and \[a\] is the acceleration of the body.
Applying on the 3kg body, we have
\[120 - {T_1} = ma\]
\[ \Rightarrow 120 - {T_1} = 3a\]
Similarly, on the 5kg block
\[{T_1} - {T_2} = 5a\]
And on the 7kg block
\[{T_2} = 7a\]
We shall solve this three equations simultaneously, hence,
Insert \[{T_2} = 7a\] into \[{T_1} - {T_2} = 5a\], then,
\[{T_1} - 7a = 5a\]
\[ \Rightarrow {T_1} = 12a\]
Inserting this into \[120 - {T_1} = 3a\], we have
\[120 - 12a = 3a\]
\[ \Rightarrow 120 = 15a\]
Dividing both sides by 10, we get
\[a = 8m{s^{ - 2}}\]
Then, \[{T_1}\] can be calculated from
\[120 - {T_1} = 3 \times 8\]
\[ \Rightarrow {T_1} = 120 - 24 = 96N\]
Then the tension in the second cord \[{T_2}\] can be calculated from
\[{T_2} = 7a\] as
\[{T_2} = 7 \times 8 = 56N\]

Hence, the correct option is C

Note: Alternatively, one could take the entire system as one block which will be the sum of all three blocks and calculate the acceleration as thus
\[a = \dfrac{{120}}{M} = \dfrac{{120}}{{3 + 5 + 7}} = \dfrac{{120}}{{15}}\]
Hence, by computation,
\[a = 8m{s^{ - 2}}\]