
Answer
110.4k+ views
Hint Here, we are given the inside and outside temperature of a refrigerator. The inside of a refrigerator should be treated as a sink and the outside of the refrigerator should be taken as the source. We need to find the amount of heat delivered per unit work done. For that we first need to find the amount of heat extracted from the fridge per unit work.
Complete step by step solution
The reversible refrigeration cycle is the ideal reverse Carnot cycle or Carnot refrigeration cycle. A Carnot refrigerator is a device that removes heat \[\left( {{Q_2}} \right)\] from the low temperature source or the inside of the refrigerator \[\left( {{T_2}} \right)\] to a higher temperature \[\left( {{T_1}} \right)\] sink or the outside of the refrigerator by using mechanical work (W).
Where \[{Q_1}\] is the heat delivered to the surroundings
and \[{Q_2}\] is the heat extracted from the refrigerator
The work done by the refrigerator, $W = {Q_2} - {Q_1}$.
Now, the coefficient of performance of a Carnot refrigerator is given by:
\[\beta = \dfrac{{{Q_2}}}{W} = \dfrac{{{T_2}}}{{{T_2} - {T_1}}}\] ---- (1)
Where, \[{T_2}\] is the temperature of the refrigerator, i.e. inside of the refrigerator
\[{T_1}\] is the temperature of the surroundings, i.e. outside of the refrigerator
In the question, we are given \[{T_1}\] = 315 K and \[{T_2}\] = 260 K. Putting these values in equation (1),
$
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{{T_2}}}{{{T_2} - {T_1}}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{260}}{{315 - 260}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{260}}{{55}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{52}}{{11}} \\
$
Now, we have to find the heat delivered to the surrounding for every joule of work done. For this we will take work done, $W = 1J$.
$
\Rightarrow \dfrac{{{Q_2}}}{1} = \dfrac{{52}}{{11}} \\
\Rightarrow {Q_2} = 4.72727 \\
\Rightarrow {Q_2} \approx 4.73 \\
$
This is the heat extracted from the refrigerator per joule of work done. We need to find the heat delivered to the surroundings for every 1J of work done, i.e. we need to find \[{Q_1}\] for \[W = 1J\].
$
\because W = {Q_2} - {Q_1} \\
\Rightarrow {Q_1} = {Q_2} + W \\
\Rightarrow {Q_1} = 4.73 + 1 \\
\Rightarrow {Q_1} = 5.73 \\
$
Therefore, the heat delivered to the surrounding for every joule of work done will be 5.73 J.
Note: Here is the basic diagram for the given reversible /carnot refrigerator for better understanding of the problem :
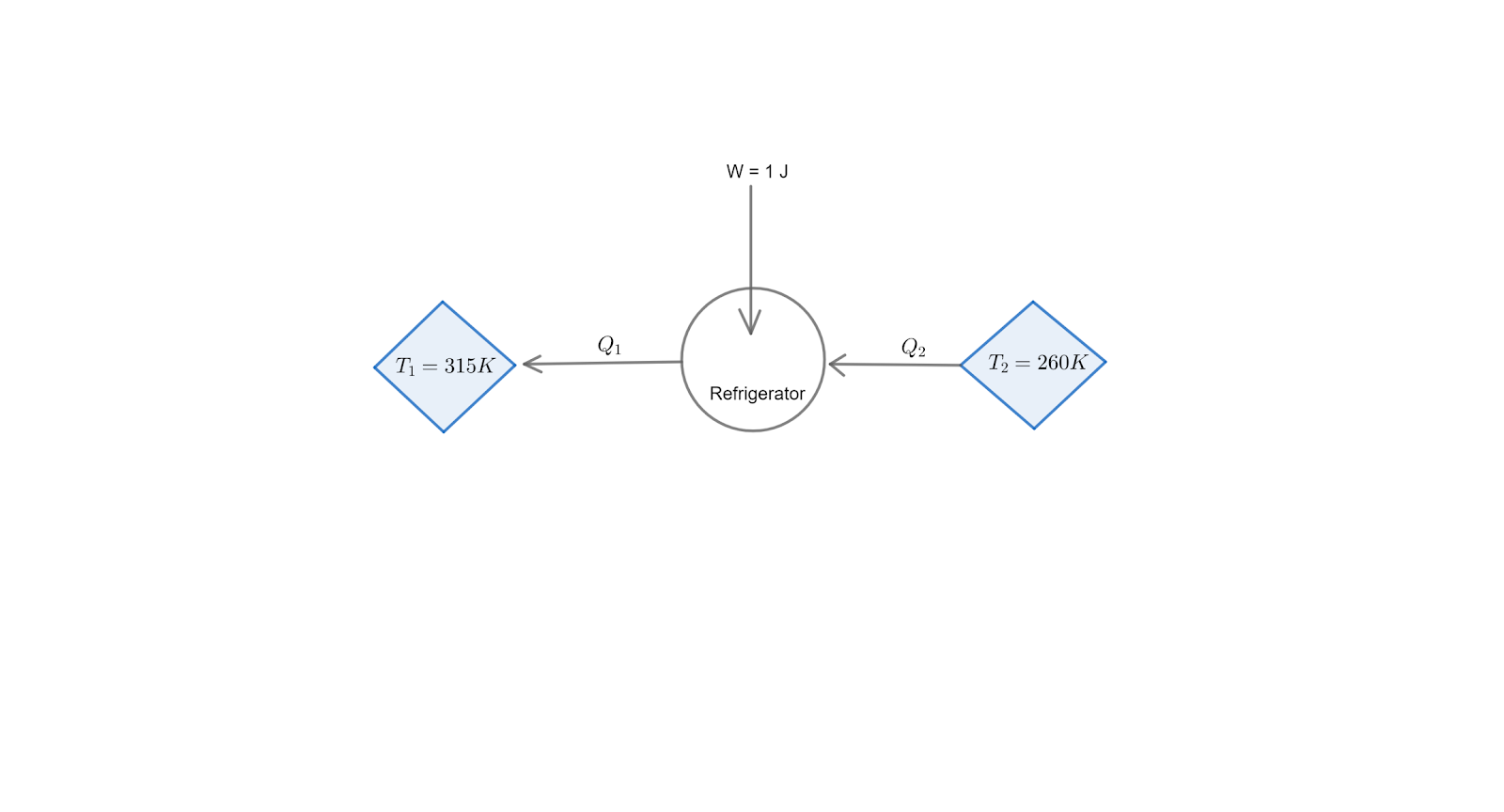
Complete step by step solution
The reversible refrigeration cycle is the ideal reverse Carnot cycle or Carnot refrigeration cycle. A Carnot refrigerator is a device that removes heat \[\left( {{Q_2}} \right)\] from the low temperature source or the inside of the refrigerator \[\left( {{T_2}} \right)\] to a higher temperature \[\left( {{T_1}} \right)\] sink or the outside of the refrigerator by using mechanical work (W).
Where \[{Q_1}\] is the heat delivered to the surroundings
and \[{Q_2}\] is the heat extracted from the refrigerator
The work done by the refrigerator, $W = {Q_2} - {Q_1}$.
Now, the coefficient of performance of a Carnot refrigerator is given by:
\[\beta = \dfrac{{{Q_2}}}{W} = \dfrac{{{T_2}}}{{{T_2} - {T_1}}}\] ---- (1)
Where, \[{T_2}\] is the temperature of the refrigerator, i.e. inside of the refrigerator
\[{T_1}\] is the temperature of the surroundings, i.e. outside of the refrigerator
In the question, we are given \[{T_1}\] = 315 K and \[{T_2}\] = 260 K. Putting these values in equation (1),
$
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{{T_2}}}{{{T_2} - {T_1}}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{260}}{{315 - 260}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{260}}{{55}} \\
\Rightarrow \dfrac{{{Q_2}}}{W} = \dfrac{{52}}{{11}} \\
$
Now, we have to find the heat delivered to the surrounding for every joule of work done. For this we will take work done, $W = 1J$.
$
\Rightarrow \dfrac{{{Q_2}}}{1} = \dfrac{{52}}{{11}} \\
\Rightarrow {Q_2} = 4.72727 \\
\Rightarrow {Q_2} \approx 4.73 \\
$
This is the heat extracted from the refrigerator per joule of work done. We need to find the heat delivered to the surroundings for every 1J of work done, i.e. we need to find \[{Q_1}\] for \[W = 1J\].
$
\because W = {Q_2} - {Q_1} \\
\Rightarrow {Q_1} = {Q_2} + W \\
\Rightarrow {Q_1} = 4.73 + 1 \\
\Rightarrow {Q_1} = 5.73 \\
$
Therefore, the heat delivered to the surrounding for every joule of work done will be 5.73 J.
Note: Here is the basic diagram for the given reversible /carnot refrigerator for better understanding of the problem :

Recently Updated Pages
Write an article on the need and importance of sports class 10 english JEE_Main

Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

If x2 hx 21 0x2 3hx + 35 0h 0 has a common root then class 10 maths JEE_Main

The radius of a sector is 12 cm and the angle is 120circ class 10 maths JEE_Main

For what value of x function fleft x right x4 4x3 + class 10 maths JEE_Main

Other Pages
If a wire of resistance R is stretched to double of class 12 physics JEE_Main

The energy stored is a condenser is in the form of class 12 physics JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In Searles apparatus when the experimental wire is class 11 physics JEE_Main




