Answer
110.7k+ views
Hint: To solve this problem, we use Kirchhoff’s Junction law to obtain the different amounts of current flowing through the resistors. This can be used to determine the amount of current flowing through the voltmeter. After this, we can use Ohm’s law to determine the resistance of the voltmeter.
Formula used:
$V = IR$
Complete step by step answer:
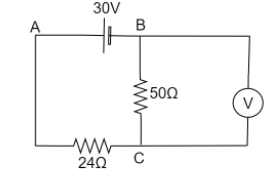
In the given circuit, we have the voltmeter connected in parallel with the $50\Omega $ resistor. The current originates from the positive terminal of the $30\;V$ battery and divides into two streams at the point $C$. The division of current can be determined by Kirchhoff’s current law or Kirchhoff’s junction law. Which states that at a junction, the total amount of current entering must be equal to the total amount of current leaving it.

Let current $I$ be the current coming out of the positive terminal of $30\;V$ battery, let ${I_1}$ be the current entering the $50\Omega $ resistor, and let ${I_2}$ be the current entering the voltmeter.
Now consider the junction $C$,
Here, current entering the junction= ${I_1}$
And the current leaving the junction$ = {I_2} + {I_3}$
Then, according to Kirchhoff's junction law,
${I_1} = {I_2} + {I_3}$
It is given that the voltage across the $50\Omega $ resistor (or between $CB\;$ or $FE\;$ ) is $18\;V$,
Thus, the current across the $50\Omega $ resistor is-
${I_2} = \dfrac{{{V_{CB}}}}{{{R_{CB}}}}$
Putting the values,
${I_2} = \dfrac{{18}}{{50}}$
${I_2} = 0.36A$
Now, the voltage across $24\Omega $ resistor (or between $DC\;$) is,
$30 - 18 = 12V$
Thus, the current across the $24\Omega $ resistor,
${I_1} = \dfrac{{{V_{DC}}}}{{{R_{DC}}}}$
Putting the values,
${I_1} = \dfrac{{12}}{{24}}$
$\Rightarrow$ ${I_1} = 0.5A$
Putting these values at the junction $C$, we get-
$0.5 = 0.36 + {I_3}$
$\Rightarrow$ ${I_3} = 0.14A$
The current that flows across the voltmeter is ${I_3} = 0.14A$
We know that the voltage across the voltmeter is, $V = 18$
Therefore, the resistance across the voltmeter is,
$R = \dfrac{V}{{{I_3}}}$
Putting the values we have,
$R = \dfrac{{18}}{{0.14}}$
$\Rightarrow$ $R = 128.6\Omega $
Thus, option (2) is the correct answer.
Note: The points in a circuit which do not have any component other than wire have the same potential and carry the same current unless it is a resistance wire or a junction. For example, the points, $B$ and $E$ have the same potential.
Formula used:
$V = IR$
Complete step by step answer:
In the given circuit, we have the voltmeter connected in parallel with the $50\Omega $ resistor. The current originates from the positive terminal of the $30\;V$ battery and divides into two streams at the point $C$. The division of current can be determined by Kirchhoff’s current law or Kirchhoff’s junction law. Which states that at a junction, the total amount of current entering must be equal to the total amount of current leaving it.

Let current $I$ be the current coming out of the positive terminal of $30\;V$ battery, let ${I_1}$ be the current entering the $50\Omega $ resistor, and let ${I_2}$ be the current entering the voltmeter.
Now consider the junction $C$,
Here, current entering the junction= ${I_1}$
And the current leaving the junction$ = {I_2} + {I_3}$
Then, according to Kirchhoff's junction law,
${I_1} = {I_2} + {I_3}$
It is given that the voltage across the $50\Omega $ resistor (or between $CB\;$ or $FE\;$ ) is $18\;V$,
Thus, the current across the $50\Omega $ resistor is-
${I_2} = \dfrac{{{V_{CB}}}}{{{R_{CB}}}}$
Putting the values,
${I_2} = \dfrac{{18}}{{50}}$
${I_2} = 0.36A$
Now, the voltage across $24\Omega $ resistor (or between $DC\;$) is,
$30 - 18 = 12V$
Thus, the current across the $24\Omega $ resistor,
${I_1} = \dfrac{{{V_{DC}}}}{{{R_{DC}}}}$
Putting the values,
${I_1} = \dfrac{{12}}{{24}}$
$\Rightarrow$ ${I_1} = 0.5A$
Putting these values at the junction $C$, we get-
$0.5 = 0.36 + {I_3}$
$\Rightarrow$ ${I_3} = 0.14A$
The current that flows across the voltmeter is ${I_3} = 0.14A$
We know that the voltage across the voltmeter is, $V = 18$
Therefore, the resistance across the voltmeter is,
$R = \dfrac{V}{{{I_3}}}$
Putting the values we have,
$R = \dfrac{{18}}{{0.14}}$
$\Rightarrow$ $R = 128.6\Omega $
Thus, option (2) is the correct answer.
Note: The points in a circuit which do not have any component other than wire have the same potential and carry the same current unless it is a resistance wire or a junction. For example, the points, $B$ and $E$ have the same potential.
Recently Updated Pages
Write an article on the need and importance of sports class 10 english JEE_Main

Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

If x2 hx 21 0x2 3hx + 35 0h 0 has a common root then class 10 maths JEE_Main

The radius of a sector is 12 cm and the angle is 120circ class 10 maths JEE_Main

For what value of x function fleft x right x4 4x3 + class 10 maths JEE_Main

Other Pages
Electric field due to uniformly charged sphere class 12 physics JEE_Main

If a wire of resistance R is stretched to double of class 12 physics JEE_Main

In Searles apparatus when the experimental wire is class 11 physics JEE_Main

The energy stored is a condenser is in the form of class 12 physics JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main