Answer
110.7k+ views
Hint At first, find the value of limiting friction on all contact surfaces which is denoted by ${f_{s\max }}$.
Then, by using the below formula, find out the acceleration for both blocks one by one from given values in question,
$\Rightarrow F - {f_{s\max }} = ma$
where, ${f_{s\max }} = $limiting value of friction
$m = $mass of a block
$a = $acceleration of a block.
Complete step by step Solution
The force which opposes motion of an object moving relative to another is called Friction. It is not a fundamental force.
The maximum value of static friction that occurs when the body is just at the sliding point of the surface of another body. In other words, we can say that it is a product of coefficients of limiting friction and normal force. We can express it mathematically as,
$\Rightarrow {f_{s\max }} = \mu N$
Normal force can also be written as,
$\Rightarrow$ $N = mg$(where, $g = 10m{s^{ - 2}}$)
To find out the acceleration from limiting friction we need to use the below expression,
$\Rightarrow$ $F - {f_{s\max }} = ma \cdots (1)$
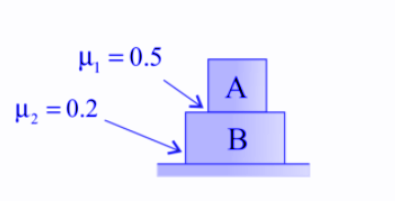
So, let ${m_a}$ and ${m_b}$ be the mass of blocks A and B respectively and let ${a_a}$ and ${a_b}$ be the acceleration of blocks A and B respectively.
So, at first, we will find the acceleration for block A
Therefore, from equation $(1)$ we get,
$\Rightarrow F - {f_{s\max }} = {m_a}{a_a} $
$\Rightarrow F - \mu \times N = {m_a}{a_a} $
$\Rightarrow F - \mu \times {m_a}g = {m_a}{a_a} $
$\Rightarrow 40 - 0.5 \times 5 \times 10 = 5{a_a} $
$\Rightarrow 40 - 25 = 5{a_a} $
$\Rightarrow 15 = 5{a_a} $
$\Rightarrow {a_a} = 3m/{s^2} $
Let ${N'}$ be the force acting upon block A from block B -
$\therefore {N'} = N + {m_b}g$
So,
$\Rightarrow {\mu _1}N - {\mu _2}N' = {m_b}{a_b} $
$\Rightarrow 0.5 \times 50 - 0.2(50 + 10 \times 10) = 10{a_b} $
$\Rightarrow 25 - 0.2(150) = 10{a_b} $
$\Rightarrow 25 - 30 = 10{a_b} $
$\Rightarrow {a_b} = \dfrac{{ - 5}}{{10}}m/{s^2} $
So, the acceleration of block B is nearly 0.
Note Limiting friction occurs when the body is just at sliding point over another’s body surface. Limiting friction always opposes the motion of an object. When the surfaces are in contact, the limiting friction always acts tangentially to them.
Then, by using the below formula, find out the acceleration for both blocks one by one from given values in question,
$\Rightarrow F - {f_{s\max }} = ma$
where, ${f_{s\max }} = $limiting value of friction
$m = $mass of a block
$a = $acceleration of a block.
Complete step by step Solution
The force which opposes motion of an object moving relative to another is called Friction. It is not a fundamental force.
The maximum value of static friction that occurs when the body is just at the sliding point of the surface of another body. In other words, we can say that it is a product of coefficients of limiting friction and normal force. We can express it mathematically as,
$\Rightarrow {f_{s\max }} = \mu N$
Normal force can also be written as,
$\Rightarrow$ $N = mg$(where, $g = 10m{s^{ - 2}}$)
To find out the acceleration from limiting friction we need to use the below expression,
$\Rightarrow$ $F - {f_{s\max }} = ma \cdots (1)$
So, let ${m_a}$ and ${m_b}$ be the mass of blocks A and B respectively and let ${a_a}$ and ${a_b}$ be the acceleration of blocks A and B respectively.
So, at first, we will find the acceleration for block A
Therefore, from equation $(1)$ we get,
$\Rightarrow F - {f_{s\max }} = {m_a}{a_a} $
$\Rightarrow F - \mu \times N = {m_a}{a_a} $
$\Rightarrow F - \mu \times {m_a}g = {m_a}{a_a} $
$\Rightarrow 40 - 0.5 \times 5 \times 10 = 5{a_a} $
$\Rightarrow 40 - 25 = 5{a_a} $
$\Rightarrow 15 = 5{a_a} $
$\Rightarrow {a_a} = 3m/{s^2} $
Let ${N'}$ be the force acting upon block A from block B -
$\therefore {N'} = N + {m_b}g$
So,
$\Rightarrow {\mu _1}N - {\mu _2}N' = {m_b}{a_b} $
$\Rightarrow 0.5 \times 50 - 0.2(50 + 10 \times 10) = 10{a_b} $
$\Rightarrow 25 - 0.2(150) = 10{a_b} $
$\Rightarrow 25 - 30 = 10{a_b} $
$\Rightarrow {a_b} = \dfrac{{ - 5}}{{10}}m/{s^2} $
So, the acceleration of block B is nearly 0.
Note Limiting friction occurs when the body is just at sliding point over another’s body surface. Limiting friction always opposes the motion of an object. When the surfaces are in contact, the limiting friction always acts tangentially to them.
Recently Updated Pages
Write an article on the need and importance of sports class 10 english JEE_Main

Write a composition in approximately 450 500 words class 10 english JEE_Main

Arrange the sentences P Q R between S1 and S5 such class 10 english JEE_Main

If x2 hx 21 0x2 3hx + 35 0h 0 has a common root then class 10 maths JEE_Main

The radius of a sector is 12 cm and the angle is 120circ class 10 maths JEE_Main

For what value of x function fleft x right x4 4x3 + class 10 maths JEE_Main

Other Pages
If a wire of resistance R is stretched to double of class 12 physics JEE_Main

The energy stored is a condenser is in the form of class 12 physics JEE_Main

Excluding stoppages the speed of a bus is 54 kmph and class 11 maths JEE_Main

Electric field due to uniformly charged sphere class 12 physics JEE_Main

In Searles apparatus when the experimental wire is class 11 physics JEE_Main