Subtraction of 3-Digit Numbers with Borrowing

We will talk about borrowing while solving subtraction of 3-digit numbers, in this section. In some classes, it's also employed to teach pupils math fundamentals. However, it is a subject that many students need help understanding and solving. Subtraction of 3-digit numbers is a widespread mathematical operation. Subtraction can be done differently depending on the number of digits and their relationship. This article discusses borrowing to subtract three-digit numbers. It will help give you an idea about how this operation works in your everyday life.

What is 3-Digit Subtraction?

In 3-digit subtraction, we need to subtract the given numbers after placing them correctly according to their place values, and we need to ensure that the bigger number is placed in the upper row while the smaller number is placed below it. After aligning them into ones, tens and hundreds of columns, we start the subtraction process. We know that the number from which the other number is subtracted is called the minuend, and the number subtracted from the minuend is called the subtrahend.

Steps to Solve 3-Digit Subtraction with Borrowing

When subtracting three digits while regrouping, we must take a number from the digit before it. Regrouping is often referred to as borrowing in subtraction. There are situations when a digit in the upper row is smaller than the digit in the bottom row while subtracting 3-digit values. In this case, we borrow a number from the preceding column so that the smaller minuend becomes bigger than the subtrahend. Regrouping or borrowing is the term used for this.

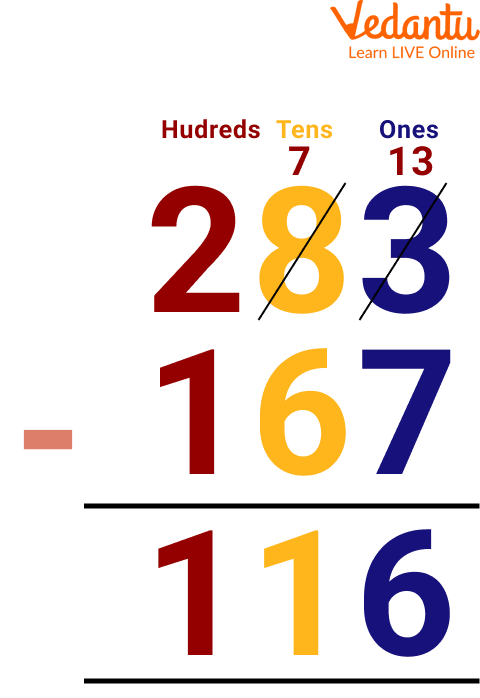
For example, let us subtract 167 from 283.

Step 1: Write the given numbers according to their place values, one below the other so that 283 is placed up and 167 is placed below it. They should be correctly placed under the ones, tens, and hundreds of columns.

Step 2: Start subtracting the numbers from the one's column. It can be seen that 3 is smaller than 7. So, let us borrow 1 from the tens column, which will make it 13. This is known as borrowing or regrouping in subtraction. So, 13 - 7 = 6. We will write the difference (6) under one column.

Step 3: After giving 1 to the one's column in the previous step, the '8' in the tens column changes to 7. Now, let us subtract the digits at the tens place and write the difference under the tens column (7 - 6 = 1).

Step 4: In the hundreds column, we will subtract 1 from 2 and write the difference (1). (2 - 1 = 1). Thus, after subtracting all the 3 digits, we get the difference as 116.

Subtraction of Numbers

Solved Examples

Here are some examples related to 3-digit subtraction with borrowing:

Q 1 Subtract 174 from 463.

Ans: Given an expression, subtract 174 from 463.

Step 1: Start subtracting the numbers under one column. We can see that 3 is less than 4. So, let us borrow 1 from the tens column, which will make it 13. Now, 13 - 4 = 9. Write the difference (9) in one column.

Step 2: Moving on to the tens column, we know that after giving 1 to the one column in the previous step, the '6' in the tens column changes to 5. But 5 is again smaller than 7. So, let us borrow 1 from the hundreds column, which will make it 15. Now, 15 - 7 = 8. We will write the difference (8) in the tens column.

Step 3: Now, subtract the numbers under the hundreds column. Since 1 was given to the tens column, the '4' in the hundreds of column changes to 3. Now, 3 - 1 = 2, so we will write the difference (2) in the hundreds column. Thus, after subtracting all the 3 digits, we get the difference as 289.

Thus, the result of the subtraction of 174 and 463 is 289.

Q 2 Subtract 165 from 574.

Ans: Given an expression, subtract 165 from 574.

Step 1: Start subtracting the numbers under the one column. We can see that 4 is less than 5. So, let us borrow 1 from the tens column which will make it 14. Now, 14 - 5 = 9. Write the difference (9) in ones column.

Step 2: Moving on to the tens column, we know that after giving 1 to the ones column in the previous step, the '4' in the tens column changes to 3. But 3 is again smaller than 6. So, let us borrow 1 from the hundreds column, which will make it 13. Now, 13 - 6 = 7. We will write the difference (7) in the tens column.

Step 3: Now, let us subtract the numbers under the hundreds column. Since 1 was given to the tens column, the '5' in the hundreds column changes to 4. Now, 4 - 1 = 3, so we will write the difference (3) in the hundreds column. Thus, after subtracting all the 3 digits, we get the difference as 379.

Thus, the result of the subtraction of 165 and 544 is 379.

Practice Problems on 3-Digit Addition and Subtraction

Here are some practice problems on 3-digit addition and subtraction:

Q 1. Subtract 396 from 436.

Ans. 40

Q 2. Subtract 689 from 905.

Ans. 216

Q3. Subtract 389 from 666.

Ans. 277

3-Digit Subtraction with Regrouping Worksheet

Given below is the 3-Digit Subtraction with Regrouping Worksheet that students should solve on their own to evaluate their learning.

3-digit Subtraction with Regrouping Worksheet

Summary

This is a very common problem many people face daily. It is easy to forget the 3-digit number when you write it down, but it becomes a real challenge if you subtract it from something else. In this article, we have discussed the subtraction of 3-digit numbers with borrowing. We have also shown how to do it and explained its importance. Many people are interested in how to subtract 3-digit numbers. In this lesson, we have covered borrowing and how it can be used to solve problems. It provides a simple algorithm to solve some examples and practice problems.