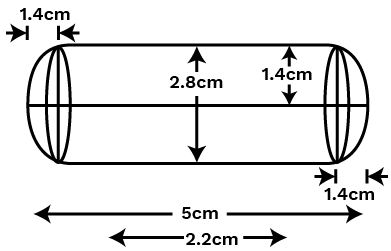
Complete Resource of NCERT Solutions for Class 10 Maths Chapter 12 - Free PDF Download
NCERT Solutions for Class 10 Maths Chapter 12 Surface Area and Volume
FAQs on NCERT Solutions for Class 10 Maths Chapter 12 Surface Area and Volume
1. List out all the formulas of Class 9 Maths Chapter 13 Surface Area and Volume
All the important formulas that are included in Class 9 Maths Chapter 13 Surface Area and Volume are listed in the table below:
NCERT Class 9 Maths Chapter 13 Surface Area and Volume Formulas | |||
Objects | Total Surface Area | Curved Surface Area | Volume |
Cube | 6a2 | 4a2 | a2 |
Cuboid | 2(lb+bh+hl) | 2(l+b)h | lbh |
Right-circular Cone | πr(l+r) | πrl | ⅓πr2h |
Right-circular Cylinder | 2πr(h+r) | 2πrh | π2h |
Sphere | 4πr2 | 4πr2 | 4/3πr3 |
Hollow Hemisphere | 2πr2 | 2πr2 | 2/3πr3 |
Solid Hemisphere | 3πr2 | 2πr2 | 2/3πr3 |
2. What are the important topics that come under NCERT Solutions for Class 9 Maths Chapter 13 Surface Areas and Volumes?
The important topics that are included under NCERT Class 9 Maths Chapter 13 Surface Areas and Volumes are mentioned below:
Curved surface area, total surface area and volume of cubes and cuboids
Surface area and volume of a right circular cone and right-circular cylinder.
The curved surface area of a sphere and a hemisphere
Surface area and volume of a sphere, hollow hemisphere and solid hemisphere
Curved surface area and volume of a right circular cylinder and cone.
All the above-mentioned topics are very important for the CBSE Class 9 final examination. Students must practise these topics and formulas regularly for better performance.
3. Where can I download NCERT Solutions for Class 9 Maths Chapter 13 pdf for free?
You can download free PDFs of NCERT Solutions for Class 9 Maths Chapter 13 by registering yourself on the Vedantu app. We provide free PDF downloads of solved NCERT Solutions which are created by expert teachers in a very simple and step-by-step manner.
4. How can Vedantu help me in scoring good grades in Class 10 Maths?
Class 10 holds an important place in the life of every student. Securing good grades on 10th boards is what every student wishes for. Therefore, it is important to have the best study partner for exam preparations. Given that, Vedantu comes with exclusive study materials developed by a team of experts. Also, you will get NCERT Solutions for Class 10 Maths, mock tests, and previous year papers for Class 10 Maths.
5. Is Class 10 Maths Chapter 13 important for Boards?
Class 10 Maths Chapter 13 is essential for Boards. This chapter may present you with long word-problem-type questions. Since it is fairly interesting and fun to solve, all you need to do is recall the key formulas of surface areas and volumes and their applications. You can use Vedantu's study materials to help you understand all of the ideas. You will be given a series of questions to aid you with quality practices.
6. How is Chapter 13 ‘Surface area and volume’ helpful in real life?
Chapter 13 ‘Surface area and volume’ is not just a chapter that teaches you a few formulas but if you understand the concepts thoroughly, you can apply them in real-world situations as well. In our daily life, we apply them unknowingly. For example, filling oil in a can, covering the floor with tiles, making a box out of cardboard, or wrapping a box with gift paper, all come under surface area and volume.
7. Are PDFs of NCERT Solutions for Class 10 Maths Chapter 13 available online?
Yes, PDFs of NCERT Solutions for Class 10 Maths Chapter 13 are available on the online platform of Vedantu (vedantu.com). It is freely accessible for download from the website as well as the Vedantu app. The NCERT solutions are offered in an easy-to-understand and detailed format. Subject matter experts have ensured that the finest answers are curated for students. You can browse over these solutions and get clarity on any sort of query or doubt.
8. How should I prepare myself for Class 10 CBSE Boards?
The Class 10 boards are essential in determining your specializing subject for later. Hence, we recommend that you take the following steps to ensure good scores in the Class 10 Boards:
Follow the CBSE syllabus.
Choose books wisely.
Focus on important chapters and topics.
Once the subject is prepared, revise and practice on a regular basis.
Solve the sample papers and also previous years' exam papers.
9. How to avoid mistakes in surface area and volume in Chapter 12 maths class 10?
Understanding what surface area and volume mean is key. Imagine the difference between a box's painted surface and the amount of air it can hold. When solving problems, use the correct formula for the shape (cube, cylinder, etc.) and plug in the right measurements (length, radius, etc.). Always double-check your calculations and make sure your answer has the correct unit (square centimetres for area, cubic centimetres for volume). By following these simple tips, you'll be a surface area and volume pro in no time!
10. Why is surface area and volume important in class 10 maths surface area and volume ?
These concepts are crucial in real-world applications! Surface area helps calculate paint needed, material costs, or heat transfer in objects. Volume helps determine how much liquid a container can hold or the amount of material needed to build something.
11. What is the key concept of surface area and volume class 10?
Surface area and volume are like two sides of the same coin; they both deal with 3D shapes, but in different ways. Surface area focuses on the total "outer covering" of a shape, like the amount of paint you'd need to coat a box. Volume, on the other hand, deals with the "inner space" a shape can hold, like how much juice a cylindrical glass can contain. Mastering these concepts is like having a 3D measuring toolkit to solve problems involving boxes, cans, balls, and more!




















 Watch Video
Watch Video