NCERT Solutions for Class 12 Maths Miscellaneous Exercise Chapter 6 - Application of Derivatives

Miscellaneous Exercise

1. Show that the function given by $f(x) = \dfrac{{\log x}}{x}$ has maximum at $x = e$.

Ans: $f(x) = \dfrac{{\log x}}{x}$

${f^\prime }(x) = \dfrac{{x\left( {\dfrac{1}{x}} \right) - \log x}}{{{x^2}}} = \dfrac{{1 - \log x}}{{{x^2}}}$

${f^\prime }(x) = 0$

$\Rightarrow 1 - \log x = 0$

$\Rightarrow \log x = 1$

$\Rightarrow \log x = \log e$

$\Rightarrow x = e$

${f^{\prime \prime }}(x) = \dfrac{{{x^2}\left( { - \dfrac{1}{x}} \right) - (1 - \log x)(2x)}}{{{x^4}}}$

$= \dfrac{{ - x - 2x(1 - \log x)}}{{{x^4}}}$

$= \dfrac{{ - 3 + 2\log x}}{{{x^3}}}$

${f^{\prime \prime }}(e) =  = \dfrac{{ - 3 + 2\log e}}{{{e^3}}} = \dfrac{{ - 3 + 2}}{{{e^3}}} = \dfrac{{ - 1}}{{{e^3}}} < 0$

$f$ is the maximum at $x = e$

2. The two equal sides of an isosceles triangle with fixed base $b$ are decreasing at the rate of $3\;{\text{cm}}$ per second. How fast is the area decreasing when the two equal sides are equal to the base? 

Ans: Let $\vartriangle ABC$ be isosceles where \[BC\]is the base of fixed length $b$. 

Let the length of the two equal sides of $\vartriangle ABC$ be $a$. 

Draw $AD \bot BC$.

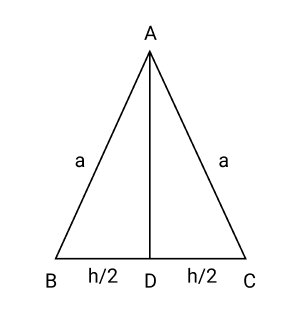
$AD = \sqrt {{a^2} - \dfrac{{{b^2}}}{4}} $

Area of triangle $= \dfrac{1}{2}b\sqrt {{a^2} - \dfrac{{{b^2}}}{4}} $

$\dfrac{{dA}}{{dt}} = \dfrac{1}{2}b \cdot \dfrac{{2a}}{{2\sqrt {{a^2} - \dfrac{{{b^2}}}{4}} }}\dfrac{{da}}{{dt}} = \dfrac{{ab}}{{\sqrt {4{a^2} - {b^2}} }}\dfrac{{da}}{{dt}}$

$\dfrac{{da}}{{dt}} =  - 3\;{\text{cm}}/{\text{s}}$

$\therefore \dfrac{{da}}{{dt}} = \dfrac{{ - 3ab}}{{\sqrt {4{a^2} - {b^2}} }}$

when $a = b$

$\dfrac{{dA}}{{dt}} = \dfrac{{ - 3{b^2}}}{{\sqrt {4{b^2} - {b^2}} }} = \dfrac{{ - 3{b^2}}}{{\sqrt {3{b^2}} }} =  - \sqrt {3b}$

3. Find the intervals in which the function $f$ given by $f(x) = \dfrac{{4\sin x - 2x - x\cos x}}{{2 + \cos x}}$ Is 

(i) increasing (ii) decreasing

Ans: $f(x) = \dfrac{{4\sin x - 2x - x\cos x}}{{2 + \cos x}}$

$\therefore {f^\prime }(x) = \dfrac{{(2 + \cos x)(4\cos x - 2 - \cos x + x\sin x) - (4\sin x - 2x - x\cos x)( - \sin x)}}{{{{(2 + \cos x)}^2}}}$

$= \dfrac{{(2 + \cos x)(3\cos x - 2 + x\sin x) + \sin x(4\sin x - 2x - x\cos x)}}{{{{(2 + \cos x)}^2}}}$

$= \dfrac{{6\cos x - 4 + 2x\sin x + 3{{\cos }^2}x - 2\cos x + x\sin x\cos x + 4{{\sin }^2}x - 2{{\sin }^2}x - 2x\sin x - x\sin x\cos x}}{{{{(2 + \cos x)}^2}}}$

$= \dfrac{{4\cos x - 4 + 3{{\cos }^2}x + 4{{\sin }^2}x}}{{{{(2 + \cos x)}^2}}}$

$= \dfrac{{4\cos x - {{\cos }^2}x}}{{{{(2 + \cos x)}^2}}} = \dfrac{{\cos x(4 - \cos x)}}{{{{(2 + \cos x)}^2}}}$

${f^\prime }(x) = 0$

$\Rightarrow \cos x = 0\cos x = 4$

$\cos x \ne 4$

$\cos x = 0$

$\Rightarrow x = \dfrac{\pi }{2},\dfrac{{3\pi }}{2}$

$\operatorname{In} \left( {0,\dfrac{\pi }{2}} \right)$ and $\left( {\dfrac{{3\pi }}{2},2\pi } \right),{f^\prime }(x) > 0$

$f(x)$ is increasing for $0 < x < \dfrac{x}{2}$ and $\dfrac{{3\pi }}{2} < x < 2\pi $.

$\operatorname{In} \left( {\dfrac{\pi }{2},\dfrac{{3\pi }}{2}} \right),{f^\prime }(x) < 0$

$f(x)$ is decreasing for $\dfrac{\pi }{2} < x < \dfrac{{3\pi }}{2}$.

4. Find the intervals in which the function $f$ given by $f(x) = {x^3} + \dfrac{1}{{{x^3}}},x \ne 0$ is

(i) increasing 

(ii) decreasing

Ans: $f(x) = {x^3} + \dfrac{1}{{{x^3}}}$

$\therefore {f^\prime }(x) = 3{x^2} - \dfrac{3}{{{x^4}}} = \dfrac{{3{x^6} - 3}}{{{x^4}}}$

${f^\prime }(x) = 0 \Rightarrow 3{x^6} - 3 = 0 \Rightarrow {x^6} = x =  \pm 1$

In $( - \infty ,1)$ and $(1,\infty )$ i.e.., when $x <  - 1$ and $x > 1,{f^\prime }(x) > 0.$ when $x <  - 1$ and $x > 1,f$ is increasing. In $( - 1,1)$ i.e., when $ - 1 < x < 1,{f^\prime }(x) < 0$.

Thus, when $ - 1 < x < 1,f$ is decreasing.

5. Find the maximum area of an isosceles triangle inscribed in the ellipse $\dfrac{x^{2}}{a^{2}}+\dfrac{y^{2}}{b^{2}}=1$ with its vertex at one end of the major axis.

Ans:

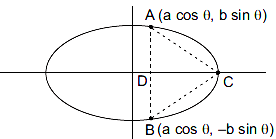
Ellipse $\dfrac{{{x^2}}}{{{a^2}}} + \dfrac{{{y^2}}}{{{b^2}}} = 1$

Let $A B C$, be the triangle inscribed in the ellipse where vertex $C$ is at $(a,0)$. Since the ellipse is symmetrical with $x$-axis and $y - axis$ ${y_1} =  \pm \dfrac{b}{a}\sqrt {{a^2} - x_1^2} .$

Coordinates of $A$ are $\left( { - {x_{1,}}\dfrac{b}{a}\sqrt {{a^2} - {x^2}1} } \right)$ and coordinates of $B$ are $\left( {{x_1}, - \dfrac{b}{a}\sqrt {{a^2} - x_1^2} .} \right)$ As the point $\left( { - {x_1},{y_1}} \right)$ lies on the ellipse, the area of triangle $A B C$ is $A = \dfrac{1}{2}\mid a\left( {\dfrac{{2b}}{a}\sqrt {{a^2} - x_1^2} } \right) + \left( { - {x_1}} \right)\left( { - \dfrac{b}{a}\sqrt {{a^2} - x_1^2} } \right) + \left( { - {x_1}} \right)\left( { - \dfrac{b}{a}\sqrt {{a^2} - {x^2}1} } \right)$

$\Rightarrow A = ba\sqrt {{a^2} - {x^2}1}  + {x_1}\dfrac{b}{a}\sqrt {{a^2} - x_1^2} $

$\therefore \dfrac{{dA}}{{d{x_1}}} = \dfrac{{ - 2xb}}{{2\sqrt {{a^2} - x_1^2} }} + \dfrac{b}{a}\sqrt {{a^2} - x_1^2}  - \dfrac{{2bx_1^2}}{{{a^2}\sqrt {{a^2} - x_1^2} }}$

$= \dfrac{b}{{2\sqrt {{a^2} - x_1^2} }}\left[ { - {x_1}a + \left( {{a^2} - x_1^2} \right) - x_1^2} \right]$

$= \dfrac{{b\left( { - 2x_1^2 - x_1^2 + {a^2}} \right)}}{{a\sqrt {{a^2} - x_1^2} }}$

$\dfrac{{dA}}{{d{x_1}}} = 0$

$\Rightarrow  - 2x_1^2 - {x_1}a + {a^2} = 0$

$\Rightarrow {x_1} = \dfrac{{a \pm \sqrt {{a^2} - 4( - 2)\left( {{a^2}} \right)} }}{{2( - 2)}}$

$= \dfrac{{a \pm \sqrt {9{a^2}} }}{{ - 4}}$

$= \dfrac{{a \pm 3a}}{{ - 4}}$

$\Rightarrow {x_1} =  - a,\dfrac{a}{2}$

${x_1}$ cannot be equal to $a$. $\therefore {x_1} = \dfrac{a}{2} \Rightarrow {y_1} = \dfrac{b}{a}\sqrt {{a^2} - \dfrac{{{a^2}}}{4}}  = \dfrac{{ba}}{{2a}}\sqrt 3  = \dfrac{{\sqrt {3b} }}{2}$

Now,$\dfrac{{{d^2}A}}{{d{x^2}1}} = \dfrac{b}{a}\left\{ {\dfrac{{\sqrt {{a^2} - x_1^2} \left( { - 4{x_1} - a} \right) - \left( { - 2x_1^2 - {x_1}a + {a^2}} \right)\dfrac{{\left( { - 2{x_1}} \right)}}{{2\sqrt {{a^2} - x_1^2} }}}}{{{a^2} - x_1^2}}} \right\}$

$= \dfrac{b}{a}\left\{ {\dfrac{{\left( {{a^2} - x_1^2} \right)\left( { - 4{x_1} - a} \right) + {x_1}\left( { - 2x_1^2 - {x_1}a + {a^2}} \right)}}{{{{\left( {{a^2} - x_1^2} \right)}^{\dfrac{2}{3}}}}}} \right\}$

$= \dfrac{b}{a}\left\{ {\dfrac{{2{x^3} - 3{a^2}x - {a^3}}}{{{{\left( {{a^2} - x_1^2} \right)}^{\dfrac{3}{2}}}}}} \right\}$

when ${x_1} = \dfrac{a}{2}$,

$\dfrac{{{d^2}A}}{{dx_1^2}} = \dfrac{b}{a}\left\{ {\dfrac{{2\dfrac{{{a^3}}}{8} - 3\dfrac{{{a^3}}}{2} - {a^3}}}{{{{\left( {\dfrac{{3{a^2}}}{4}} \right)}^{\dfrac{3}{2}}}}}} \right\} = \dfrac{b}{a}\left\{ {\dfrac{{\dfrac{{{a^3}}}{4} - \dfrac{3}{2}{a^3} - {a^3}}}{{{{\left( {\dfrac{{3{a^2}}}{4}} \right)}^{\dfrac{3}{2}}}}}} \right\}$

$= \dfrac{b}{a}\left\{ {\dfrac{{\dfrac{9}{4}{a^3}}}{{{{\left( {\dfrac{{3{a^2}}}{4}} \right)}^{\dfrac{3}{2}}}}}} \right\} < 0$

Area is the maximum when ${x_1} = \dfrac{a}{2}$. Maximum area of the triangle is $A = b\sqrt {{a^2} - \dfrac{{{a^2}}}{4}}  + \left( {\dfrac{a}{2}} \right)\dfrac{b}{a}\sqrt {{a^2} - \dfrac{{{a^2}}}{4}} $

$= ab\dfrac{{\sqrt 3 }}{2} + \left( {\dfrac{a}{2}} \right)\dfrac{b}{a} \times \dfrac{{a\sqrt 3 }}{2}$

$= \dfrac{{ab\sqrt 3 }}{2} + \dfrac{{ab\sqrt 3 }}{4} = \dfrac{{3\sqrt 3 }}{4}ab$

6. A tank with a rectangular base and rectangular sides, open at the top is to be constructed so that its depth is \[2m\]and volume is $8{m^3}$. If the building of the tank costs Rs 70 per sq meter for the base and Rs 45 per sq meter for the sides. What is the cost of the least expensive tank?

Ans: Let \[l,b\]and $h$ represent the length, breadth, and height of the tank respectively.

height $(h) = 2m$

Volume of the tank $= 8\;{{\text{m}}^3}$ Volume of the tank $= l \times b \times {\text{h}}$ $8 = l \times b \times 2$

$\Rightarrow lb = 4 \Rightarrow b = \dfrac{4}{l}$

Area of the base $= lb = 4$ 

Area of 4 walls $(A) = 2h(l + b)$

$\therefore A = 4\left( {l + \dfrac{4}{l}} \right)$

$\Rightarrow \dfrac{{dA}}{{dl}} = 4\left( {1 - \dfrac{4}{{{l^2}}}} \right)$

$Now,\dfrac{{dA}}{{dl}} = 0$

$\Rightarrow l - \dfrac{4}{{{l^2}}} = 0$

$\Rightarrow {l^2} = 4$

$\Rightarrow l =  \pm 2$

Therefore, we have $l = 4$. 

$\therefore b = \dfrac{4}{l} = \dfrac{4}{2} = 2$

$\dfrac{{{d^2}A}}{{d{l^2}}} = \dfrac{{32}}{{{l^3}}}$

$l = 2,\dfrac{{{d^2}A}}{{d{l^2}}} = \dfrac{{32}}{8} = 4 > 0.$

Area is the minimum when $l = 2$. 

We have $l = b = h = 2$.

Cost of building base \[ = \] Rs $70{\text{x}}(lb) = $ Rs$70(4) = $ Rs 280

Cost of building walls $= Rs2h(l + h) \times 45 = Rs90(2)(2 + 2) = $ 

Rs $8(90) = $ Rs 720

Required total cost $= Rs(280 + 720) = $ Rs 1000

7. The sum of the perimeter of a circle and square is $k$, where $k$ is some constant. Prove that the sum of their area is least when the side of the square is double the radius of the circle. 

Ans: $2\pi r + 4a = k$ (where $k$ is constant) $\Rightarrow a = \dfrac{{k - 2\pi r}}{4}$

the sum of the areas of the circle and the square $(A)$ is given by,

$A = \pi {r^2} + {a^2} = \pi {r^2} + \dfrac{{{{(k = 2\pi r)}^2}}}{{16}}$

$\therefore \dfrac{{dA}}{{dr}} = 2\pi r + \dfrac{{2(k - 2\pi r)(2\pi )}}{{16}} = 2\pi r$

$=  - \dfrac{{\pi (k - 2\pi r)}}{4}$

Now, $\dfrac{{dA}}{{dr}} = 0$

$\Rightarrow 2\pi r = \dfrac{{\pi (k - 2\pi r)}}{4}$

$8r = k - 2\pi r$

$\Rightarrow (8 + 2\pi )r = k$

$\Rightarrow r = \dfrac{k}{{8 + 2\pi }} = \dfrac{k}{{2(4 + \pi )}}$

Now, $\dfrac{{{d^2}A}}{{d{r^2}}} = 2\pi  + \dfrac{{{\pi ^2}}}{2} > 0$

$\therefore $ where $r = \dfrac{k}{{2(4 + \pi )}},\dfrac{{{d^2}A}}{{d{r^2}}} > 0.$

area is least when $r = \dfrac{k}{{2(4 + \pi )}}$ where $r = \dfrac{k}{{2(4 + \pi )}},$

$a = \dfrac{{k - 2\pi \left[ {\dfrac{k}{{2(4 + \pi )}}} \right]}}{4} = \dfrac{{8k + 2\pi k - 2\pi k}}{{2(4 + \pi ) \times 4}} = \dfrac{k}{{4 + \pi }} = 2r$

8. A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is $10\;{\text{m}}$. Find the dimensions of the window to admit maximum light through the whole opening. 

Ans: $x$ and $y$ are the length and breadth of the rectangular window.

Radius of semicircular opening $= \dfrac{x}{2}$

$\therefore x + 2y + \dfrac{{\pi {\text{x}}}}{2} = 10$

$\Rightarrow x\left( {1 + \dfrac{\pi }{2}} \right) + 2y = 10$

$\Rightarrow 2y = 10 - x\left( {1 + \dfrac{\pi }{2}} \right)$

$\Rightarrow y = 5 - x\left( {\dfrac{1}{2} + \dfrac{\pi }{4}} \right)$

$A = xy + \dfrac{\pi }{2}{\left( {\dfrac{x}{2}} \right)^2}$

$= x\left[ {5 - x\left( {\dfrac{1}{2} + \dfrac{\pi }{4}} \right)} \right] + \dfrac{\pi }{8}{\text{x}}{x^2}$

$= 5x - {x^2}\left( {\dfrac{1}{2} + \dfrac{\pi }{4}} \right) + \dfrac{\pi }{8}{\text{x}}{x^2}$

$\therefore \dfrac{{dA}}{{dx}} = 5 - 2x\left( {\dfrac{1}{2} + \dfrac{\pi }{4}} \right) + \dfrac{\pi }{4}x$

$\dfrac{{{d^2}A}}{{d{x^2}}} =  - \left( {1 - \dfrac{\pi }{2}} \right) + \dfrac{\pi }{4} =  - 1 - \dfrac{\pi }{4}$

$\dfrac{{dA}}{{dx}} = 0$

$\Rightarrow 5 - x\left( {1 + \dfrac{\pi }{2}} \right) + \dfrac{\pi }{4}x = 0$

$\Rightarrow 5 - x - \dfrac{\pi }{4}x = 0$

$\Rightarrow x\left( {1 + \dfrac{\pi }{4}} \right) = 5$

$\Rightarrow x = \dfrac{5}{{\left( {1 + \dfrac{\pi }{4}} \right)}} = \dfrac{{20}}{{\pi  + 4}}$

$x = \dfrac{{20}}{{\pi  + 4}},\dfrac{{{d^2}A}}{{d{x^2}}} < 0.$

area is maximum when length $x = \dfrac{{20}}{{\pi  + 4}}m$. 

Now, $y = 5 - \dfrac{{20}}{{\pi  + 4}}\left( {\dfrac{{2 + \pi }}{4}} \right) = 5 - \dfrac{{5(2 + \pi )}}{{\pi  + 4}} = \dfrac{{10}}{{\pi  + 4}}m$

the required dimensions length $= \dfrac{{20}}{{\pi  + 4}}m$ and breadth $= \dfrac{{10}}{{\pi  + 4}}m$.

9. A point of the hypotenuse of a triangle is at distance $a$ and $b$ from the sides of the triangle. Show that the minimum length of the hypotenuse is ${\left( {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} \right)^{\dfrac{3}{2}}}$ 

Ans: $\vartriangle ABC$ right-angled at $B$. $AB = x$ and $BC = y$.

$P$ be a point on hypotenuse such that ${\text{P}}$ is at a distance of a and ${\text{b}}$ from the sides \[AB\]and \[BC\]respectively.

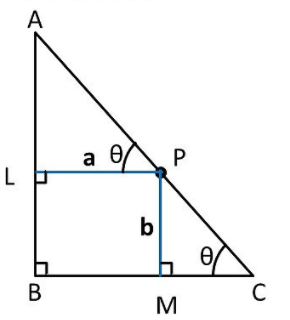
$\angle c = \theta $

$AC = \sqrt {{x^2} + {y^2}} $

$PC = b\operatorname{cosec} \theta $

$AP = a\sec \theta $

$AC = AP + PC$

$AC = b\operatorname{cosec} \theta  + a\sec \theta  \ldots ..(1)$

$\therefore \dfrac{{d(AC)}}{{d\theta }} =  - b\operatorname{cosec} \theta \cot \theta  + a\sec \theta \tan \theta $

$\therefore \dfrac{{d(AC)}}{{d\theta }} = 0$

$\Rightarrow a\sec \theta \tan \theta  = b\cos \operatorname{ec} \theta \cot \theta $

$\Rightarrow \dfrac{a}{{\cos \theta }} \cdot \dfrac{{\sin \theta }}{{\cos \theta }} = \dfrac{b}{{\sin \theta }}\dfrac{{\cos \theta }}{{\sin \theta }}$

$\Rightarrow a{\sin ^3}\theta  = b{\cos ^3}\theta $

$\Rightarrow {(a)^{\dfrac{1}{3}}}\sin \theta  = {(b)^{\dfrac{1}{3}}}\cos \theta $

$\Rightarrow \tan \theta  = {\left( {\dfrac{b}{a}} \right)^{\dfrac{1}{3}}}$

$\therefore \sin \theta  = \dfrac{{{{(b)}^{\dfrac{1}{3}}}}}{{\sqrt {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} }}$ and $\cos \theta  = \dfrac{{{{(a)}^{\dfrac{1}{3}}}}}{{\sqrt {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} }}\quad  \ldots ..(2)$

clearly $\dfrac{{{d^2}(AC)}}{{d{\theta ^2}}} < 0$ when $\tan \theta  = {\left( {\dfrac{b}{a}} \right)^{\dfrac{1}{3}}}$.

the length of the hypotenuse is the maximum when $\tan \theta  = {\left( {\dfrac{b}{a}} \right)^{\dfrac{1}{3}}}$. 

Now, when $\tan \theta  = {\left( {\dfrac{b}{a}} \right)^{\dfrac{1}{3}}}$

$\tan \theta  = {\left( {\dfrac{b}{a}} \right)^{\dfrac{1}{3}}}$

$AC = \dfrac{{b\sqrt {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} }}{{{b^{\dfrac{1}{3}}}}} + \dfrac{{a\sqrt {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} }}{{{a^{\dfrac{1}{3}}}}}$

$= a\sqrt {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} \left( {{b^{\dfrac{2}{3}}} + {a^{\dfrac{2}{3}}}} \right)$

$= {\left( {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} \right)^{\dfrac{3}{2}}}$

maximum length of the hypotenuse is $= {\left( {{a^{\dfrac{2}{3}}} + {b^{\dfrac{2}{3}}}} \right)^{\dfrac{3}{2}}}$.

10. Find the points at which the function $f$ given by $f(x) = {(x - 2)^4}{(x + 1)^3}$ has

(i) local maxima

(ii) local minima

(iii) point of inflexion

Ans: $f(x) = {(x - 2)^4}{(x + 1)^3}$

$\therefore {f^\prime }(x) = 4{(x - 2)^3}{(x + 1)^3} + 3{(x + 1)^2}{(x - 2)^4}$

$= {(x - 2)^3}{(x + 1)^2}[4(x + 1) + 3(x - 2)]$

$= {(x - 2)^3}{(x + 1)^2}(7x - 2)$

${f^\prime }(x) = 0 \Rightarrow x =  - 1$ and $x = \dfrac{2}{7}$ or $x = 2$

for $x$ close to $\dfrac{2}{7}$ and to left of $\dfrac{2}{7},{f^\prime }(x) > 0$.

for $x$ close to $\dfrac{2}{7}$ and to right of $\dfrac{2}{7},{f^\prime }(x) > 0$. $x = \dfrac{2}{7}$ is point of local minima.

as the value of $x$ varies ${f^\prime }(x)$ does not changes its sign.

$x =  - 1$ is point of inflexion.

11. Find the absolute maximum and minimum values of the function $f$ given by

$f(x) = {\cos ^2}x + \sin x,x \in [0,\pi ]$

Ans: $f(x) = {\cos ^2}x + \sin x$

${f^\prime }(x) = 2\cos x( - \sin x) + \cos x$

$=  - 2\sin x\cos x + \cos x$

${f^\prime }(x) = 0{\text{ }}$

$\Rightarrow 2\sin x\cos x = \cos x \Rightarrow \cos x(2\sin x - 1) = 0$

$\Rightarrow \sin x = \dfrac{1}{2}$ or $\cos x = 0$

$\Rightarrow x = \dfrac{\pi }{6}$, or $\dfrac{\pi }{2}$ as $x \in [0,\pi ]$

$f\left( {\dfrac{\pi }{6}} \right) = {\cos ^2}\dfrac{\pi }{6} + \sin \dfrac{\pi }{6} = {\left( {\dfrac{{\sqrt 3 }}{2}} \right)^2} + \dfrac{1}{2} = \dfrac{5}{4}$

$f(0) = {\cos ^2}0 + \sin 0 = 1 + 0 = 1$

$f(\pi ) = {\cos ^2}\pi  + \sin \pi  = {( - 1)^2} + 0 = 1$

$f\left( {\dfrac{\pi }{2}} \right) = {\cos ^2}\dfrac{\pi }{2} + \sin \dfrac{\pi }{2} = 0 + 1 = 1$

absolute maximum value of $f$ is $\dfrac{5}{4}$ at $x = \dfrac{\pi }{6}$

The absolute minimum value of $f$ is 1 at $x = 0,x = \dfrac{\pi }{2}$, and $\pi $.

12. Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius $\mathrm{r}$ is $\dfrac{4 r}{3}$.

Ans: $V = \dfrac{1}{3}\pi {R^2}h$

$BC = \sqrt {{r^2} - {R^2}} $

$h = r + \sqrt {{r^2} - {R^2}} $

$\therefore V = \dfrac{1}{3}\pi {R^2}\left( {r + \sqrt {{r^2} - {R^2}} } \right) = \dfrac{1}{3}\pi {R^2}r + \dfrac{1}{3}\pi {R^2}\sqrt {{r^2} - {R^2}} $

$\therefore \dfrac{{dV}}{{dR}} = \dfrac{2}{3}\pi Rr + \dfrac{{2\pi }}{3}\pi R\sqrt {{r^2} - {R^2}}  + \dfrac{{{R^2}}}{3} \cdot \dfrac{{( - 2R)}}{{2\sqrt {{r^2} - {R^2}} }}$

$= \dfrac{2}{3}\pi Rr + \dfrac{{2\pi }}{3}\pi R\sqrt {{r^2} - {R^2}}  - \dfrac{{{R^3}}}{{3\sqrt {{r^2} - {R^2}} }}$

$= \dfrac{2}{3}\pi Rr + \dfrac{{2\pi Rr\left( {{r^2} - {R^2}} \right) - \pi {R^3}}}{{3\sqrt {{r^2} - {R^2}} }}$

$= \dfrac{2}{3}\pi Rr + \dfrac{{2\pi R{r^2} - 3\pi R{r^3}}}{{3\sqrt {{r^2} - {R^2}} }}$

$\dfrac{{dV}}{{d{R^2}}} = 0$

$\Rightarrow \dfrac{{2\pi rR}}{3} = \dfrac{{3\pi {R^2} - 2\pi R{r^2}}}{{3\sqrt {{r^2} - {R^2}} }}$

$\Rightarrow 2{\text{r}}\sqrt {{r^2} - {R^2}}  = 3{R^2} - 2{r^2}$

$\Rightarrow 4{r^2}\left( {{r^2} - {R^2}} \right) = {\left( {3{R^2} - 2{r^2}} \right)^2}$

$\Rightarrow 14{r^4} - 4{r^2}{R^2} = 9{R^4} + 4{r^4} - 12{R^2}{r^2}$

$\Rightarrow 9{R^4} - 8{r^2}{R^2} = 0$

$\Rightarrow 9{R^2} = 8{r^2}$

$\Rightarrow {R^2} = \dfrac{{8{r^2}}}{9}$

$\dfrac{{{d^2}V}}{{d{R^2}}} = \dfrac{{2\pi r}}{3} + \dfrac{{3\sqrt {{r^2} - {R^2}} \left( {2\pi {r^2} - 9\pi {R^2}} \right) - \left( {2\pi {R^3} - 3\pi {R^3}} \right)( - 6R)\dfrac{1}{{2{{\sqrt {{r^2} - R} }^2}}}}}{{9\left( {{r^2} - {R^2}} \right)}}$

$= \dfrac{{2\pi r}}{3} + \dfrac{{3\sqrt {{r^2} - {R^2}} \left( {2\pi {r^2} - 9\pi {R^2}} \right) - \left( {2\pi {R^3} - 3\pi {R^3}} \right)(3R)\dfrac{1}{{2{{\sqrt {{r^2} - R} }^2}}}}}{{9\left( {{r^2} - {R^2}} \right)}}$

when ${R^2} = \dfrac{{8{r^2}}}{9},\dfrac{{{d^2}V}}{{d{R^2}}} < 0$.

volume is the maximum when ${R^2} = \dfrac{{8{r^2}}}{9}$. ${R^2} = \dfrac{{8{r^2}}}{9}$, 

height of the cone $= r + \sqrt {{r^2} - \dfrac{{8{R^2}}}{9}}  = r + \sqrt {\dfrac{{{r^2}}}{9}}  = r + \dfrac{r}{3} = \dfrac{{4r}}{3}$

13. Let f be a function defined on $\left[ a,b \right]$such that ${{f}^{'}}(x)>0$ for all $x\in (a,b)$. Then prove that f is an increasing function on (a, b).

Ans: Consider I be the interval (a, b)

Given that ${{f}^{'}}(x)>0$for all x in an interval I. Consider ${{x}_{1}},{{x}_{1}},\in $I with ${{x}_{1}}<{{x}_{2}}$.

By Lagrange’s Mean Value Theorem, we have, 

$\frac{f({{x}_{2}})-f({{x}_{1}})}{{{x}_{2}}-{{x}_{1}}}={{f}^{'}}(c)\,$where ${{x}_{1}}<c<{{x}_{2}}$

$\Rightarrow f({{x}_{2}})-f({{x}_{1}})=({{x}_{2}}-{{x}_{1}}){{f}^{'}}(c)$where ${{x}_{1}}<c<{{x}_{2}}$

Now ${{x}_{1}}<{{x}_{2}}$

$\Rightarrow {{x}_{2}}-{{x}_{1}}>0$……….(1)

 Also, ${{f}^{'}}(x)>0$for all $x$ in an interval I

$\Rightarrow {{f}^{'}}(c)>0$

From equation (1), $f({{x}_{2}})-f({{x}_{1}})>0$

$\Rightarrow f({{x}_{1}})<f({{x}_{2}})$

Thus, for every pair of points ${{x}_{1}},{{x}_{1}},\in $I with ${{x}_{1}}<{{x}_{2}}$

$\Rightarrow f({{x}_{1}})<f({{x}_{2}})$

Therefore, f(x) is strictly increasing in I.

14. Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius $R$ is $\dfrac{{2R}}{{\sqrt 3 }}$, also find the maximum volume.

Ans: $h = 2\sqrt {{R^2} - {r^2}} $

$V = \pi {r^2}h = 2\pi {r^2}\sqrt {{R^2} - {r^2}} $

$\therefore \dfrac{{dV}}{{dr}} = 4\pi r\sqrt {{R^2} - {r^2}}  + \dfrac{{2\pi {r^2}( - 2r)}}{{2\sqrt {{R^2} - {r^2}} }}$

$= 4\pi r\sqrt {{R^2} - {r^2}}  - \dfrac{{2\pi {r^3}}}{{\sqrt {{R^2} - {r^2}} }}$

$= \dfrac{{4\pi r\left( {{R^2} - {r^2}} \right) - 2\pi {r^3}}}{{\sqrt {{R^2} - {r^2}} }}$

$= \dfrac{{4\pi r{R^2} - 6\pi {r^3}}}{{\sqrt {{R^2} - {r^2}} }}$

Now, $\dfrac{{dV}}{{dr}} = 0 \Rightarrow 4\pi r{R^2} - 6\pi {r^3} = 0$

$\Rightarrow {r^2} = \dfrac{{2{R^2}}}{3}$

$\dfrac{{{d^2}V}}{{d{r^2}}} = \dfrac{{\sqrt {{R^2} - {r^2}} \left( {4\pi {R^2} - 18\pi {r^2}} \right) - \left( {4\pi r{R^2} - 6\pi {r^3}} \right)\dfrac{{( - 2r)}}{{2\sqrt {{R^2} - {r^2}} }}}}{{\left( {{R^2} - {r^2}} \right)}}$

$= \dfrac{{\left( {{R^2} - {r^2}} \right)\left( {4\pi {R^2} - 18\pi {r^2}} \right) + r\left( {4\pi r{R^2} - 6\pi {r^3}} \right)}}{{{{\left( {{R^2} - {r^2}} \right)}^{\dfrac{3}{2}}}}}$

$= \dfrac{{4\pi {R^4} - 22\pi {r^2}{R^2} + 12\pi {r^4} + 4\pi {r^2}{R^2}}}{{{{\left( {{R^2} - {r^2}} \right)}^{\dfrac{3}{2}}}}}$

${r^2} = \dfrac{{2{R^2}}}{3},\dfrac{{{d^2}V}}{{d{r^2}}} < 0.$

volume is maximum when ${r^2} = \dfrac{{2{R^2}}}{3}$. ${r^2} = \dfrac{{2{R^2}}}{3}$.

height of the cylinder is $2\sqrt {{R^2} - \dfrac{{2{R^2}}}{3}}  = 2\sqrt {\dfrac{{{R^2}}}{3}}  = \dfrac{{2R}}{{\sqrt 3 }}$.

the volume of the cylinder is maximum when the height of the cylinder is $\dfrac{{2R}}{{\sqrt 3 }}$

15. Show that the height of the cylinder of greatest volume which can be inscribed in a right circular cone of height ${\text{h}}$ and semi veritical angle a is one-third that of the cone and the greatest volume of the cylinder is $\dfrac{4}{{27}}\pi {h^2}{\tan^2}a$.

Ans:

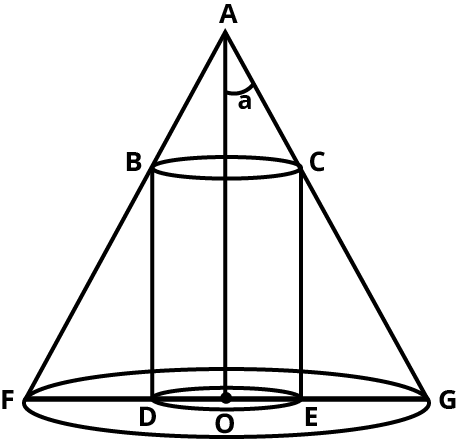
$r = h\tan a$

since $\Delta AOG$ is similar to $\Delta CEG$,

$\dfrac{{AO}}{{OG}} = \dfrac{{CE}}{{EG}}$

$\Rightarrow \dfrac{h}{r} = \dfrac{H}{{r - R}}$

$\Rightarrow H = \dfrac{h}{r}(r - R) = \dfrac{h}{{h\tan a}}(h\tan a - R) = \dfrac{1}{{\tan a}}(h\tan a - R)$

volume of the cylinder is $V = \pi {R^2}H = \dfrac{{\pi {R^2}}}{{\tan a}}(h\tan a - R)$

$= \pi {R^2}h - \dfrac{{\pi {R^3}}}{{\tan a}}$

$\therefore \dfrac{{dV}}{{dR}} = 2\pi Rh\dfrac{{3\pi {R^2}}}{{\tan a}}$

$\dfrac{{dV}}{{dR}} = 0$

$\Rightarrow 2\pi Rh = \dfrac{{3\pi {R^2}}}{{\tan a}}$

$\Rightarrow 2h\tan a = 3R$

$\Rightarrow R = \dfrac{{2h}}{3}\tan a$

$\dfrac{{{d^2}V}}{{d{R^2}}} = 2\pi Rh - \dfrac{{6\pi R}}{{\tan a}}$

And, for $R = \dfrac{{2h}}{3}\tan a$, we have:

$\dfrac{{{d^2}V}}{{d{R^2}}} = 2\pi h - \dfrac{{6\pi }}{{\tan a}}\left( {\dfrac{{2h}}{3}\tan a} \right) = 2\pi h - 4\pi h =  - 2\pi h < 0$

volume of the cylinder is greatest when $R = \dfrac{{2h}}{3}\tan a$. $R = \dfrac{{2h}}{3}\tan a,H = \dfrac{1}{{\tan a}}\left( {h\tan a - \dfrac{{2h}}{3}\tan a} \right) = \dfrac{1}{{\tan a}}\left( {\dfrac{{h\tan a}}{3}} \right) = \dfrac{h}{3}$.

the maximum volume of the cylinder can be obtained as

$\pi {\left( {\dfrac{{2h}}{3}\tan a} \right)^2}\left( {\dfrac{h}{3}} \right) = \pi \left( {\dfrac{{4{h^2}}}{9}{{\tan }^2}a} \right)\left( {\dfrac{h}{3}} \right) = \dfrac{4}{{27}}\pi {h^3}{\tan ^2}a$

16. A cylindrical tank of radius 10 m is being filled with wheat at the rate of 314 cubic meters per hour. Then the depth of the wheat is increasing at the rate of

(A) 1 m / h 

(B) 0.1 m / h 

(C) 1.1 m / h

(D) 0.5 m / h

Ans. $V = \pi {({\text{ radius }})^2}{\text{x}}$ height

$ = \pi {(10)^2}h\quad ($ radius $ = 10\;{\text{m}})$

$ = 100\pi h$

$\dfrac{{dV}}{{dt}} = 100\pi \dfrac{{dh}}{{dt}}$

Tank is being filled with wheat at rate of 314 cubic meters per hour.

 $\dfrac{{dV}}{{dt}} = 314\;{{\text{m}}^3}/{\text{h}}$

$314 = 100\pi \dfrac{{dh}}{{dt}}$

$ \Rightarrow \dfrac{{dh}}{{dt}} = \dfrac{{314}}{{100(3.14)}} = \dfrac{{314}}{{314}} = 1$

The depth of wheat is increasing at $1\;{\text{m}}/{\text{h}}$.

The correct answer is ${\mathbf{A}}$.

Conclusion

Miscellaneous Exercise Class 12 Chapter 6 is important for understanding various concepts thoroughly. Application of Derivatives Class 12 Miscellaneous Exercise Solutions cover diverse problems that require the application of multiple formulas and techniques. It's important to focus on understanding the underlying principles behind each question rather than just memorizing solutions. Remember to understand the theory behind each concept, practice regularly, and refer to solved examples to master this exercise effectively.

Class 12 Maths Chapter 6: Exercises Breakdown

CBSE Class 12 Maths Chapter 6 Other Study Materials

Chapter-Specific NCERT Solutions for Class 12 Maths

Given below are the chapter-wise NCERT Solutions for Class 12 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.