NCERT Solutions for Class 5 Maths Chapter 3 How Many Squares - FREE PDF Download
NCERT Solutions Class 5 Maths Chapter 3 - How Many Squares are provided to help students prepare for their exams. Subject matter experts create the NCERT Solutions following the CBSE Class 5 Maths Syllabus.
NCERT Solutions for Class 5 Maths of How Many Squares helps students to draw squares from a given collection of dots. This chapter provides a series of questions about measuring the sides of squares that will help them improve their understanding of the subject. Students can download NCERT Solutions for Class 5 Maths Chapter 3 in PDF format and start practicing for exams.
Glance on Maths Chapter 3 Class 5 - How Many Squares?
NCERT Class 5 Maths Chapter 3 Solutions explains how to count and identify squares in different shapes and patterns.
It defines a square as a four-sided figure with equal sides and right angles.
To find squares in a pattern, look for smaller squares inside the big one.
To find the area of a square, multiply one side by itself.
Look for equal sides and right angles to find squares.
Squares can be different sizes in one big shape.
Practice finding squares in different patterns to get better at it.
Knowing about squares helps you solve problems with shapes and areas in real life.
This article contains chapter notes, important questions, exemplar solutions, exercises and video links for Chapter 3 - How Many Squares?, which you can download as PDFs.
There are 18 fully solved questions in class 5th maths chapter 3 How Many Squares?.
1.
Measure the side of the red square on the dotted sheet. Draw here as many rectangles as possible using 12 such squares.
(i) How many rectangles could you make? ________
Ans: On the dotted sheet, the side of the square is 1 cm.
We can make 7 possible rectangles in the following figure.
We can make 7 rectangles.
2 rectangles are of size 1 $\times$ 12 centimetre.
1 rectangle is of size 2 $\times$ 6 centimetre.
4 rectangles are of size 3 $\times$ 4 centimetre
Hence, number of rectangles = 2 + 1 + 4 = 7
2. Each rectangle is made out of 12 equal squares, so all have the same area, but the length of the boundary will be different.
(i) Which of these rectangles has the longest perimeter?
Ans: To find the perimeter of the rectangle for 1 x 12 cm we have to do to following steps:
By using Formula : 2(length + breadth)
= 2(1 + 12)
= 2 $\times$ 13 = 26 cm
To find the perimeter of a rectangle for 2 $\times$ 6 cm we have to do to following steps:
By using Formula : 2(length + breadth)
= 2(2 + 6)
= 2 $\times$ 8 = 16 cm
To find the perimeter of the rectangle for 3 $\times$ 4 cm we have to do to following steps:
By using Formula : 2(length + breadth)
= 2(3 + 4)
= 2 $\times$ 7 = 14 cm
(ii) Which of these rectangles has the smallest perimeter?
Ans: The rectangle 3 $\times$ 4 cm has the smallest perimeter.
3. Measure Stamps
Stamp D covers 12 squares. Each square is of side 1 cm. So the area of stamp D is 12 square cm.
Look at these interesting stamps.
(a) How many squares of one centimetre side does stamp A cover and stamp B? ________
Ans: By using the formula:
Area = length $\times$ breadth
= 4 $\times$ 2 = 8 square cm
(b) Which stamp has the biggest area?
Ans: The biggest area is Stamp A.
(i) How many squares of side 1 cm does this stamp cover?
Ans: This stamp covers 18 squares with sides of 1 cm.
(ii) How much is the area of the biggest stamp? _____ square cm.
Ans: 18 square cm is the area of the biggest stamp.
(c) Which two stamps have the same area? _____
Ans: Stamp ‘D’ and Stamp ‘F’ have the same area.
(i) How much is the area of each of these stamps? ____ square cm.
Ans: The area of Stamp ‘D’ is 12 square cm,
The area of Stamp ‘F’ is 12 square cm.
(d) The area of the smallest stamp is _____ square cm.
Ans: The area of the smallest stamp that is Stamp ‘E’ is length $\times$ breadth
= 2 $\times$ 2
= 4 $cm^{2}$
(i) The difference between the area of the smallest and the biggest stamp is _____ square cm.
Ans: To find the difference between the area of the smallest and the biggest stamp of square cm
Area of the Biggest Stamp - Area of the Smallest Stamp
= 18 - 4 = 14 square cm.
4. Guess
(a) Which has the bigger area — one of your footprints or the page of this book?
Ans: When the area of the footprints is compared to the area of the page of the textbook, the page of the textbook has a larger area than the footprints.
(b) Which has the smaller area - two five-rupee notes together or a hundred rupee note?
Ans: A hundred rupee note takes the smaller area by comparing the two five-rupee notes together or a hundred rupee note.
(c) Look at a 10 rupee-note. Is its area more than hundred square cm?
Ans: No, a 10 rupee note has an area size of less than 100 square centimetres.
(d) Is the area of the blue shape more than the area of the yellow shape? Why?
Ans: No, the blue shape's area and the yellow shape's area are equal. By cutting out these shapes and using the square grid paper to calculate their areas, it is simple to figure out this.
e) Is the perimeter of the yellow shape more than the perimeter of the blue shape? Why?
Ans: No, the yellow shape's perimeter is smaller than the blue shape's. You can measure their limits with a ruler or a thread to prove this.
6. How Big is My Hand?
Trace your hand on the squared sheet on the next page.
How will you decide whose hand is bigger - your hand or your friend’s hand?
What is the area of your hand? _______ square cm.
What is the area of your friend’s hand? _______ square cm.
Ans: Trace both of your hands and your friend's hands on a square piece of paper. Find how many of each type of square there are: full, half-filled, more than half-filled, and less than half-filled. Remove the squares that are less than half full. To find the area, add the number of squares that are filled and those that are more than half full.
Considering Figure A:
6 complete squares are present.
There are 7 half-filled squares.
6 squares are more than half-filled.
Area = 6 + (7/2) + 6
= 6 + 3.5 + 6
= 15.5 square cm is the area.
Considering Figure B:
13 complete squares are present.
There are 4 half-filled squares.
13 squares are more than half-filled.
Area = 13 + (4/2) + 13
= 13 + 2 + 13
= 28 square cm is the area.
7. My Footprints
My footprint is longer!
But my footprint is wider. So whose foot is bigger?
(i) Whose footprint is larger yours or your friend’s?
Ans: My footprint is larger.
(ii) How will you decide? Discuss.
Ans: We can decide by tracing both footprints on a squared sheet of paper and counting the number of complete squares, half-filled squares, and more than half-filled squares inside each footprint. The one with the larger total area is the bigger footprint.
(iii) Is the area of both your footprints the same?
Ans: No, the area of both footprints is not the same.
8. Guess which animal’s footprint will have the same area as yours. Discuss.
Here are some footprints of animals — in actual sizes. Guess the area of their footprints.
Ans: A monkey's footprint may be similar in size to mine.
Hen's Footprint:
Number of complete squares: 5
Number of half-filled squares: 4
Number of more-than-half-filled squares: 3
Area of hen's footprint:
= 5 + (4/2) + 3
= 5 + 2 + 3
= 10 square units
Dog's Footprint:
Number of complete squares: 8
Number of half-filled squares: 6
Number of more-than-half-filled squares: 5
Area of dog's footprint;
= 8 + (6/2) + 5
= 8 + 3 + 5
= 16 square units
From this example, you can see the dog's footprint has a larger area than the hen's footprint.
9. Make big squares and rectangles like this to find the area faster.
Ans:
Area of P:
= 5 + 2/2 + 7
= 5 + 1 + 7
= 13 square cm
Area OF Q:
= 7 + 2/2 + 4
= 7 + 1 + 4
= 12 square cm
Area of R:
= 6 + 0/2 + 9
= 6 + 9
= 15 square cm
Area of S:
= 4 + 0/2 + 6
= 4 + 6
= 10 square cm
Area of T:
= 96 + 4/2 + 21
= 96 + 2 + 21
= 119 square cm
10. How Many Squares in Me?
(i) What is the area of this triangle?
Ans: The area of this triangle is 1 square centimetre.
(ii) The triangle is half the rectangle of area 2 square cm. So its area is ___ square cm.
Ans: The triangle is half the rectangle of area 2 square cm. So its area is 1 square cm.
(iii) Is this shape half of the big rectangle?
Ans: Yes, the above shape is half of the big rectangle.
(iv) Hmmm…… So its area is _____ square cm.
Ans: So its area is 4 square cm.
10. Write the area (in square cm) of the shapes below.
Ans:
11. Sameena: Both the big triangles in this rectangle have the same area.
Sadiq: But these look very different.
(i) The blue triangle is half of the big rectangle. The area of the big rectangle is 20 square cm. So the area of the blue triangle is _______ square cm.
Ans: According to the question, The area of the triangle blue is $\dfrac{1}{2}\times 20$ = 10 square cm.
(ii) And what about the red triangle?
Ans: According to the question, The area of the triangle red is half of the area of a rectangle.
So, the Area of the triangle red is 10 square cm and there are two different rectangles with two halves.
(iii) Now find the area of the two rectangles in the figure. What is the area of the red triangle? Explain.
Ans: From the figure which is given, we can say that,
Given,
The orange rectangle = 12 squares
Then, the green rectangle = 8 squares
So, the area of the orange rectangle is 12 square cm
So, the area of the green rectangle is 8 square cm
Now, the area of the orange portion of the triangle = 12/2 = 6 square cm
The area of the yellow portion of the triangle
= 8/2
= 4 cm square
Therefore, the area of the red triangle
= 6 + 4
= 10 square cm.
12. Complete the Shape
Suruchi drew two sides of a shape. She asked Asif to complete the shape with two more sides, so that its area is 10 square cm.
He completed the shape like this
(i) How did you do this?
Ans: Well, that's simple! The green area, which is 4 square centimetres, is seen. The yellow, 6 square centimetre area is below it. My form so it's 10 square centimetres in area.
(ii) Is he correct? Discuss.
Ans: Yes, he is correct about the above solution.
(iii) Explain how the green area is 4 square cm and the yellow area is 6 square cm.
Ans: To find the area of the green portion we have to solve by using,
Area of green portion = Number of complete squares + Number of half-filled squares
=$2+\dfrac{1}{2}\times 4$
= 2 + 2
= 4 square cm
To find the Area of the yellow portion = Number of complete squares + Number of half-filled squares + Number of more than half-filled squares
= $3+\dfrac{1}{2}\times 2+2$
= 3 + 1 + 2
= 6 square cm
13. Suruchi : Oh, I thought of doing it differently! If you draw like this, the area is still 10 square cm.
(i) Is Suruchi correct? How much is the blue area? Explain.
Ans: Suruchi is right,
The area of the green triangle = Half of area of rectangle measuring $4\times 2 = \dfrac{1}{2}\times 4\times 2 = 2\times 2$
=4 cm square
Area of the blue triangle = Half of the area of the rectangle measuring $ 4\times 3$
= $\dfrac{1}{2}\times 4\times 3$
= $2\times 3$
= 6 square cm.
thereby, 4 + 6 = 10 square centimetres is the total area.
(ii) Can you think of some other ways of completing the shape?
Ans: Thus, a rectangle with a 12 square centimetre area can be created. We can then draw as many triangles in it as we can after that. It can be seen in the figure next to it.
Practice time
14. This is one of the sides of a shape. Complete the shape so that its area is 4 square cm.
Ans:
Finding the completed shape = 2 complete square + 4 half square
=$2+\left ( \dfrac{1}{2}\times 4 \right )$
=2+2
=4 $cm^{2}$
15. Two sides of a shape are drawn here. Complete the shape by drawing two more sides so that its area is less than 2 square cm.
Ans:
16. Here is a rectangle of area 20 square cm.
(i) Draw one straight line in this rectangle to divide it into two equal triangles. What is the area of each of the triangles?
Ans:
Area of rectangle:
= $10\times 2$
= 20 square cm
Then, the area of two equal triangles:
= $\dfrac{20}{2}$
= 10 square cm
(ii) Draw one straight line in this rectangle to divide it into two equal rectangles. What is the area of each of the smaller rectangles?
Ans:
Area of big rectangle = 10 square cm
The area of each of the smaller rectangles is $\dfrac{20}{2}$
= 10 square cm
(iii) Draw two straight lines in this rectangle to divide it into one rectangle and two equal triangles.
(a) What is the area of the rectangle?
Ans: Area of Rectangle = (Length $\times$ Breadth)
= 2 $\times$ 5
= 10 square cm
(b) What is the area of each of the triangles?
Ans: Area of each Triangle = $\frac{1}{2}$ $\times$ Area of the smaller Rectangle
= $\frac{1}{2}$ $\times$ 10
= 5 square cm
Puzzles with Five Squares
17. Measure the side of a small square on the squared paper. Make as many shapes as possible using 5 such squares. Three Eire drawn for you.
a) How many different shapes can you draw? ___________
Ans: I can draw 12 shapes, by using 5 squares.
b) Which shape has the longest perimeter? How much? _______ cm
Ans: The smallest perimeter out of 12 shapes is Shape 4, it has 12 square cm.
c) Which shape has the shortest perimeter? How much? _______ cm
Ans: Shape 4 has the smallest perimeter of all 12 shapes, and Shape 4 has 10 cm square.
d) What is the area of the shapes? _______ square cm. That’s simple!
Ans: The total area of the shapes is 5 square cm.
Practice time
18 . Ziri tried to make some other tiles.
She started with a square of 2 cm side and made shapes like these.
Look at these carefully and find out.
(i) Which of these shapes will tile a floor (without any gaps)? Discuss.
Ans: Shapes C and D may create a floor without any gaps by following the provided figure.
Conclusion
NCERT Class 5 Maths Chapter 3 Solutions of "How Many Squares" offer a comprehensive and insightful exploration of squares and their arrangements. These solutions provide step-by-step explanations and examples to help students count the number of squares in various patterns and grids. By mastering the concept of squares, students enhance their problem-solving skills and geometric understanding. The free PDF download of NCERT Solutions facilitates easy access to valuable study materials, supporting students in their mathematical journey. Overall, these solutions encourage a deeper appreciation for patterns and shapes, fostering a solid foundation in mathematics and preparing students for more advanced concepts.
Other Study Material for CBSE Class 5 Maths Chapter 3
Chapter-Specific NCERT Solutions for Class 5 Maths
Given below are the chapter-wise NCERT Solutions for Class 5 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
Important Related Links for CBSE Class 5 Maths
FAQs on NCERT Solutions for Class 5 Maths Chapter 3 How Many Squares?
1. How can I get the NCERT Class 5 Maths Chapter 3 Solutions?
Students can easily avail of the NCERT Class 5 Maths Chapter 3 Solutions from the internet. Also, online learning platforms like Vedantu provide students with NCERT Solutions.
Moreover, these solutions also come in a PDF format which can be easily downloaded from Vedantu website or app. Students need to register to their site in some simple steps and can get access to the PDF and the material for free.
2. What are the advantages of NCERT Class 5 Maths Chapter 3 Solutions?
CBSE Solutions for How Many Squares Class 5 provided by NCERT are prepared as per CBSE guidelines (2024-25).
Skilled teachers design these solutions, which are easy to understand and help students in several ways. Also, as veteran teachers have years of experience, they know enough tricks and approaches that benefit students to fetch more marks in the exams.
3. What is the importance of How Many Squares Class 5?
Chapter 3 of How Many Squares Class 5. This chapter makes students aware of the geometric shape square and related mathematical operations.
After solving this chapter, along with the NCERT How Many Squares Class 5 Maths Chapter 3 Solutions provided by Vedantu, students will be able to draw a square using a given set of dots. As this chapter comprises questions regarding measuring sides of squares, they will also be able to answer related to this topic.
4. How many squares are there in Chapter 3 How Many Squares Class 5 Maths?
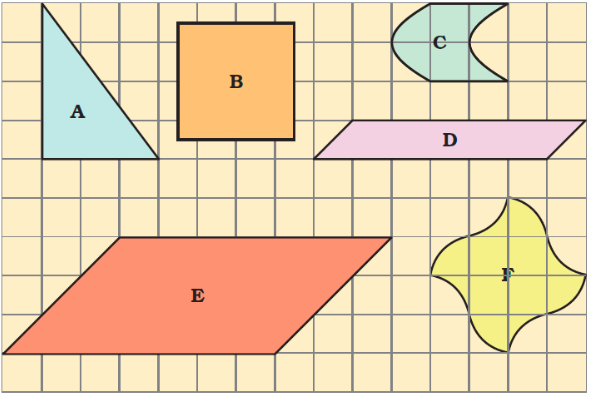
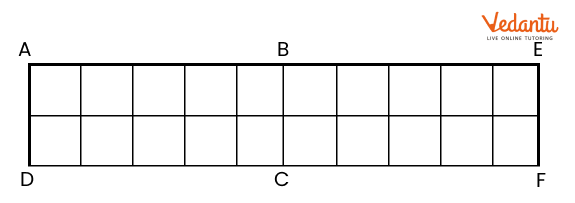
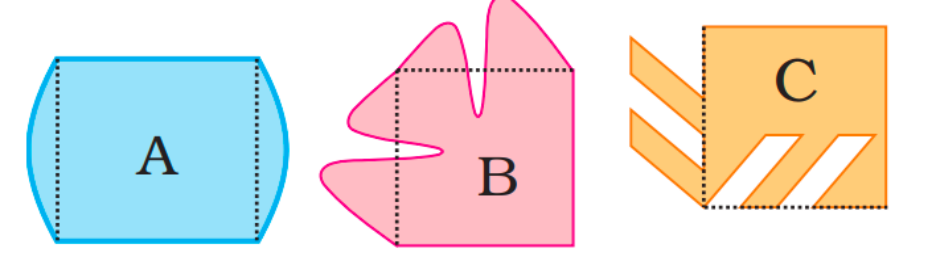
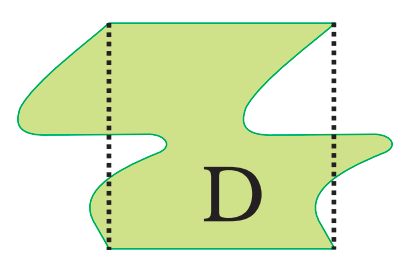
In the NCERT Chapter 3 ‘How Many Squares’ of Class 5 Maths, in stamp A, there are 18 squares of 1 cm side. Stamp B has eight squares of 1 cm side, stamp C includes six squares of 1 cm side, stamp D includes 12 squares of 1 cm side, stamp E has four squares inside it of 1 cm side, and stamp F has 12 squares of 1 cm side.
5. How many squares of 1 cm side does stamp A and stamp B cover according to ‘How Many Squares’ of Class 5 Math Chapter 3?
Stamp A has 18 squares of 1 cm side and stamp B has eight squares of 1 cm side. The area covered by stamp A is 18 square cm and the area covered by stamp B is 8 square cm. For similar solutions of the chapter, download the NCERT Solutions of Class 5 Math Chapter 3 Maths PDF and access full solutions to all the questions that are present in the chapter on Vedantu.
6. What is the area of the floor pattern made according to ‘How Many Squares’ of Class 5 Math Chapter 3?
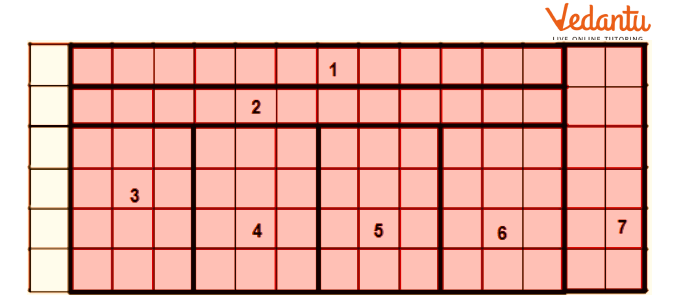
Tiles C and D will fit the floor without any gaps. Since each of these squares has a 2 cm side, the area of the floor pattern of each tile will be 2 x 2 square cm = 4 square cm. You can download the full NCERT Solutions of Class 5 Math Chapter 3 available only on Vedantu. Find all the answers to your questions and practice them to get full marks on your test. The solution PFDs can also be downloaded free of cost on Vedantu.
7. Where do I find solutions for ‘How Many Squares’ of Class 5th Chapter 3 Maths?
Get the complete NCERT Solutions of ‘How Many Squares’ of Class 5th Chapter 3 Maths available on Vedantu. You can even download the NCERT Solutions Class 5 Maths Chapter 5 PDF. This solution has answers to all the questions that are there in the chapter. The answers have been framed by experts who make sure that each solution is precise and to the point. Each question is solved in steps to give a clearer understanding of the questions.
8. Where can I get a full Class of ‘How Many Squares’ of Class 5th Chapter 3 Maths?
At Vedantu, you can get the full NCERT Solutions of ‘How Many Squares’ of Class 5th Chapter 3 Maths. Also, the student can attend classes conducted by top-class faculty by registering for the same. The NCERT solutions are even available for downloading as PDF for free if you want to access it sometime later for homework or revision. The NCERT Solution has step-by-step answers to all exercise questions. All the concepts have also been cleared through various examples. Studying this solution will help you in improving your score.
9. What is Chapter 3 - How Many Squares about in Class 5th Maths Chapter 3?
This chapter teaches kids how to find the area of shapes by counting the number of squares inside them. It helps them understand how to measure space and compare different shapes.
10. How can I help my child learn about the area from Class 5th Maths Chapter 3?
Use everyday items like tiles or graph paper to show your child how to count squares. You can also cut out shapes and have them count the squares to find the area. This makes learning fun and practical.
11. What kind of practice questions are in this Class 5th Maths Chapter 3?
The chapter has questions where kids count squares in different shapes, compare areas, solve shape puzzles, and draw shapes with a given area. These activities help them practice and understand the concept better.
12. What tools can help teach this chapter better?
Visual tools like graph paper, coloured tiles, or drawing apps can help a lot. These tools make it easier for kids to see and count the squares in Class 5th Maths Chapter 3. Games and puzzles related to the area can also make learning more enjoyable.
