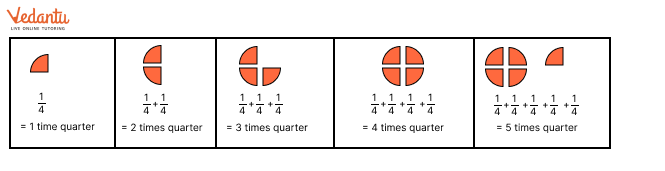NCERT Solutions for Class 6 Chapter 7 Maths - FREE PDF Download
FAQs on NCERT Solutions for Class 6 Maths Chapter 7 Fractions
1. What is included in the NCERT Solutions for Chapter 7?
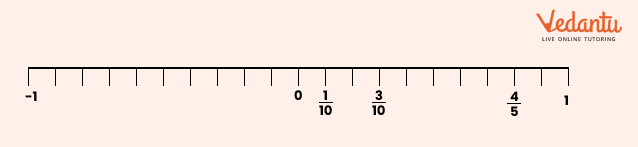
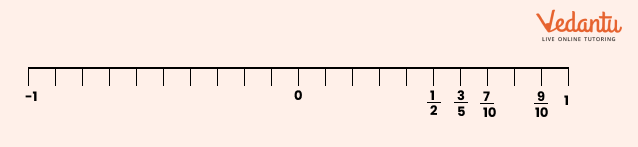
The NCERT Solutions for Chapter 7 cover explanations for fractions, including proper and improper fractions. It also includes methods for adding, subtracting, multiplying, and dividing fractions. This helps students understand how to work with fractions effectively.
2. Are there practice problems in the notes?
The notes include various practice problems for each topic in the chapter. These problems help reinforce what students have learned. Practising these problems improves their skills in handling fractions.
3. How do the notes help with understanding fractions?
The notes provide clear explanations and step-by-step methods for solving fraction problems. This makes it easier for students to understand the concepts. It also shows how fractions are used in real-life situations.
4. Are the solutions aligned with the CBSE syllabus?
The NCERT Solutions for Chapter 7 are designed to match the CBSE Class 6 Maths syllabus. This ensures that students learn the necessary material for their grades. It helps them prepare well for exams.
5. Can I download the notes for free?
The NCERT Solutions for Chapter 7 are available for FREE download in PDF format. Students can access them anytime for study and practice. This makes learning more convenient and flexible.
6. How can I use these notes to prepare for tests?
Students can use the notes to review key concepts and practice problems before tests. Going through the exercises helps reinforce their understanding. This preparation is important for doing well in exams.
7. What are equivalent fractions?
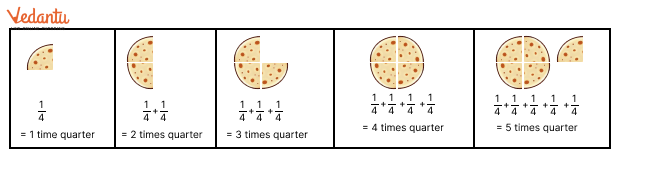
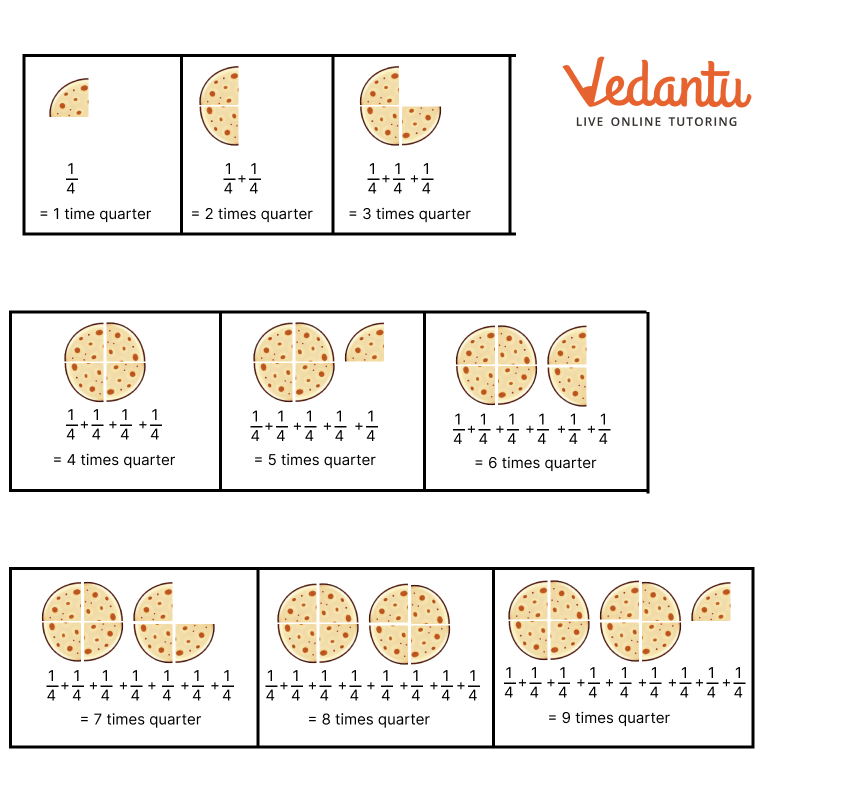
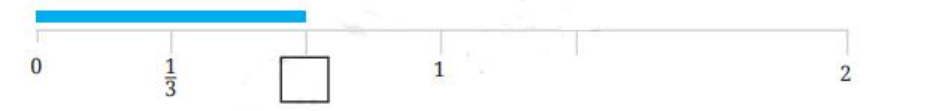
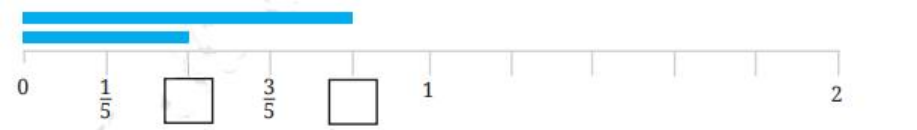
Equivalent fractions are different fractions that represent the same value. The notes explain how to find and recognize equivalent fractions. This concept is important for simplifying and comparing fractions.
8. What are mixed fractions?
Mixed fractions are combinations of whole numbers and fractions. The notes explain how to convert between mixed fractions and improper fractions. Understanding this helps with performing operations on fractions.
9. How do the notes explain adding and subtracting fractions?
The notes provide clear steps for adding and subtracting fractions, including finding a common denominator. This makes it easier for students to learn these operations. Practice problems reinforce their skills.
10. What should I do if I have questions about the notes?
If students have questions about the notes, they should review the explanations and examples provided. They can also ask teachers or classmates for help. Discussing problems can lead to better understanding.