
When it is 10:30, what kind of angle is formed by the hands of the clock?
A. Acute
B. Obtuse
C. Right
D. Straight

Answer
486.3k+ views
Hint: For solving this question, we first need to find out the angle any two consecutive numbers in clock make. Using this we will calculate the angle between the minute hand and hour hand of the clock. Since time is 10:30, so the minute hand will be at 6 and hour hand will be between 10 and 11. At last, from the formed angle we will determine the type of angle.
Complete step-by-step answer:
Here the angle between any two consecutive numbers in a clock is
Now we know that when time is 30 minutes, then the minute clock will be at 6.
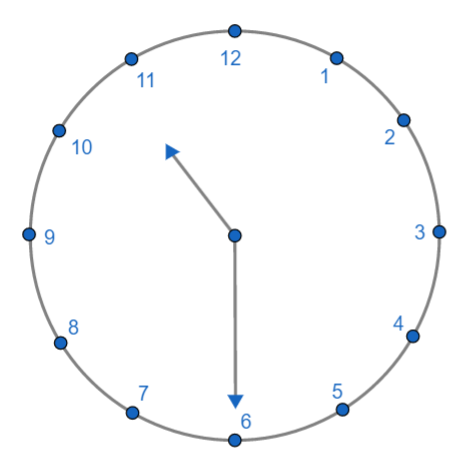
And when time is 10:30, the hour clock will be between 10 and 11. Let us draw a clock here for better understanding.
Now the number of parts (number) between 6 to 10 are 4. So an angle between 6 and 10 will be
Hence our angle will be between
Hence our required angle between the hands of a clock as 10:30 is
Since
So, the correct answer is “Option B”.
Note: Students can also directly see from the diagram that angle between 6 and 9 would be
Complete step-by-step answer:
Here the angle between any two consecutive numbers in a clock is
Now we know that when time is 30 minutes, then the minute clock will be at 6.
And when time is 10:30, the hour clock will be between 10 and 11. Let us draw a clock here for better understanding.

Now the number of parts (number) between 6 to 10 are 4. So an angle between 6 and 10 will be
Hence our angle will be between
Hence our required angle between the hands of a clock as 10:30 is
Since
So, the correct answer is “Option B”.
Note: Students can also directly see from the diagram that angle between 6 and 9 would be
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




