Question

A comet is moving in a parabolic orbit around the sun which is at the focus of a parabola. When the comet is 80 million km from the sun, the line segment from the sun to the comet makes an angle of \[\dfrac{\pi }{3}\] radians with the axis of the orbit. Find
(i) the equation of the comet’s orbit
(ii) how close does the comet come nearer to the sun? (Take the orbit as open rightward)

Answer 1

Hint: We start solving the problem by drawing the given information to get a better view of the problem. We then assume the equation of the parabola, vertex, parametric point on parabola and find the required distances. We then find the vertical distance between Sun and Comet using the cosine rule of the angle given in the problem. We equate this vertical distance with the distance given in the problem to find the only unknown present in the equation of parabola. We use the fact that the point closest to the focus in a parabola is its vertex to get the closest distance.

Complete step-by-step answer:
The orbit is rightward open. Therefore, draw a rightward open parabola with the sun at its focus and its vertex is at the origin. Let C be the position of the comet and S be the position of the sun.
Let us draw the given information to get a better view.

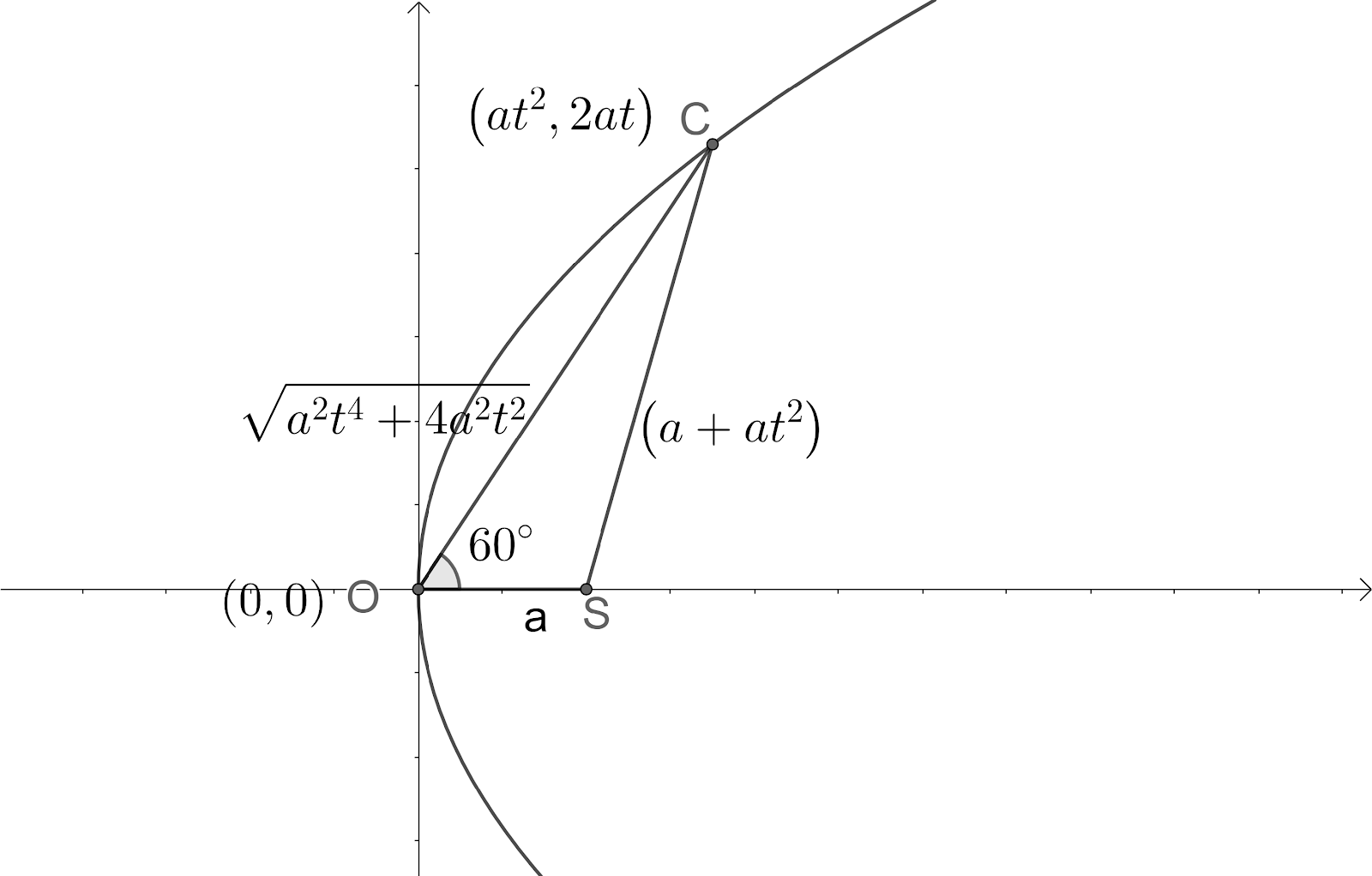
Let us assume the equation of the parabola be ${{y}^{2}}=4ax$. We know that the distance between focus and vertex in a parabola ${{y}^{2}}=4ax$ is ‘a’. According to the problem we are given that the line segment from the sun to the comet makes an angle of \[\dfrac{\pi }{3}\] radians with the axis of the orbit.
We know that the parametric equation of the point in a parabola is $C\left( a{{t}^{2}},2at \right)$. Let us find the distances OC and SC.
So, we get $OC=\sqrt{{{\left( 0-a{{t}^{2}} \right)}^{2}}+{{\left( 0-2at \right)}^{2}}}=\sqrt{{{a}^{2}}{{t}^{4}}+4{{a}^{2}}{{t}^{2}}}$.
$SC=\sqrt{{{\left( a-a{{t}^{2}} \right)}^{2}}+{{\left( 0-2at \right)}^{2}}}=\sqrt{{{\left( a-a{{t}^{2}} \right)}^{2}}+4{{a}^{2}}{{t}^{2}}}=\sqrt{{{\left( a+a{{t}^{2}} \right)}^{2}}}=\left( a+a{{t}^{2}} \right)$.
Let us use cosine rule for the angle at vertex O in the triangle OSC i.e., $\cos \left( {{60}^{\circ }} \right)=\dfrac{O{{S}^{2}}+O{{C}^{2}}-C{{S}^{2}}}{2\left( OS \right)\left( OC \right)}$
From the figure we get $\cos \left( {{60}^{\circ }} \right)=\dfrac{{{\left( a \right)}^{2}}+{{\left( \sqrt{{{a}^{2}}{{t}^{4}}+4{{a}^{2}}{{t}^{2}}} \right)}^{2}}-{{\left( a+a{{t}^{2}} \right)}^{2}}}{2\left( a \right)\left( \sqrt{{{a}^{2}}{{t}^{4}}+4{{a}^{2}}{{t}^{2}}} \right)}$.
$\Rightarrow \dfrac{1}{2}=\dfrac{{{a}^{2}}+{{a}^{2}}{{t}^{4}}+4{{a}^{2}}{{t}^{2}}-{{a}^{2}}-{{a}^{2}}{{t}^{4}}-2{{a}^{2}}{{t}^{2}}}{2\left( a \right)\left( \sqrt{{{a}^{2}}{{t}^{2}}\left( {{t}^{2}}+4 \right)} \right)}$.
$\Rightarrow 1=\dfrac{{{a}^{2}}+{{a}^{2}}{{t}^{4}}+4{{a}^{2}}{{t}^{2}}-{{a}^{2}}-{{a}^{2}}{{t}^{4}}-2{{a}^{2}}{{t}^{2}}}{\left( a \right)\left( \sqrt{{{a}^{2}}{{t}^{2}}\left( {{t}^{2}}+4 \right)} \right)}$.
$\Rightarrow 1=\dfrac{2{{a}^{2}}{{t}^{2}}}{\left( a \right)\left( at \right)\left( \sqrt{\left( {{t}^{2}}+4 \right)} \right)}$.
$\Rightarrow 1=\dfrac{2t}{\sqrt{{{t}^{2}}+4}}$.
$\Rightarrow \sqrt{{{t}^{2}}+4}=2t$.
$\Rightarrow {{t}^{2}}+4=4{{t}^{2}}$.
$\Rightarrow 3{{t}^{2}}=4$.
$\Rightarrow {{t}^{2}}=\dfrac{4}{3}$.
$\Rightarrow t=\sqrt{\dfrac{4}{3}}=\dfrac{2}{\sqrt{3}}$. We use this to find the distance between the sun and Comet.
So, we get $SC=a+a{{t}^{2}}=a\left( 1+\dfrac{4}{3} \right)=\dfrac{7a}{3}$.
So, we have found the vertical distance between Sun and Comet as$\dfrac{7a}{3}$.
But according to the problem, we are given that the comet is 80 million km from the sun.
So, we get $\dfrac{7a}{3}=80million\ km$.
$\Rightarrow a=\dfrac{240}{7}million\ km$.
So, we have got the equation of the parabola as ${{y}^{2}}=\dfrac{240}{7}x$.
(ii) We know that the nearest point to the focus in a parabola is its vertex. We know that the distance between vertex and parabola is ‘a’ which is $\dfrac{240}{7}million\ km$.

Note: We should not take the vertical distance between Sun and Comet randomly as it will not satisfy the given condition about the angle. We can make mistakes while drawing the diagram representing the information in the problem, as it is very important for solving this problem. Here we should not assume the point of the Comet as $\left( a,2a \right)$, as it will not always be the situation always.