
A cone made up of a cardboard has a vertical height of 8 cm and a radius of 6 cm. If this cone is cut along the slanted height to make a sector, what is the central angle in degrees of the sector?
(a)
(b)
(c)
(d)
Answer
505.8k+ views
Hint: First of all find the slant height of the given cone by using the formula of slant height which is equal to
Complete step-by-step answer:
The radius and height of the cone is given as 6 cm and 8 cm respectively.
In the below diagram, we have shown a cone with radius and height as r and h respectively.
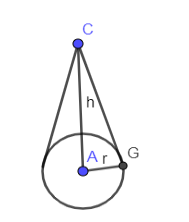
In the above figure, slant height is given as CG.
As you can see that triangle CAG is forming a right angled triangle and CG is the hypotenuse so applying Pythagoras theorem in this triangle we get,
Substituting CA as h and AG as r in the above equation we get,
Taking square root on both the sides we get,
Substituting the value of h and r as 8 cm and 6 cm respectively we get,
From the above, we have got the slant height as 10 cm.
Now, look carefully towards the below figure when we are cutting the cone along the slant height (i.e. CG) to make a sector then the radius of the sector is slant height and length of the arc is equal to the circumference of the base of the cone.
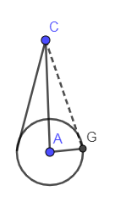
Circumference of the cone is equal to:
Substituting the value of r as 6 cm in the above formula we get,
After making a cut along the slant height the newly formed sector will look like:
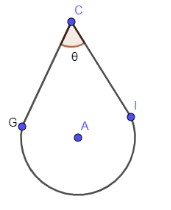
The central angle that we have to find in the above problem is the angle
We know that
Substituting arc length as
Substituting
From the above calculations, we have found the value of the central angle is equal to
Hence, the correct option is (a).
Note: The point where you go wrong in this problem is in writing the value of radius and arc length for the sector because it demands a clear visualization of how the cutting of cone along slant height will give you radius of the sector as slant height and arc length as the circumference of the base.
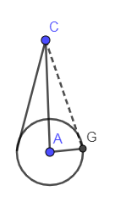
You can visualize it by imagining the given cone in the form of a birthday cap so when we make a cut along the slant height then the cone shape will roll out into an arc. For instance, let us assume that the below figure is in the birthday cap so when we make a cut alone CG then the folded cone will open into an arc whose radius is CG.
Complete step-by-step answer:
The radius and height of the cone is given as 6 cm and 8 cm respectively.
In the below diagram, we have shown a cone with radius and height as r and h respectively.

In the above figure, slant height is given as CG.
As you can see that triangle CAG is forming a right angled triangle and CG is the hypotenuse so applying Pythagoras theorem in this triangle we get,
Substituting CA as h and AG as r in the above equation we get,
Taking square root on both the sides we get,
Substituting the value of h and r as 8 cm and 6 cm respectively we get,
From the above, we have got the slant height as 10 cm.
Now, look carefully towards the below figure when we are cutting the cone along the slant height (i.e. CG) to make a sector then the radius of the sector is slant height and length of the arc is equal to the circumference of the base of the cone.

Circumference of the cone is equal to:
Substituting the value of r as 6 cm in the above formula we get,
After making a cut along the slant height the newly formed sector will look like:

The central angle that we have to find in the above problem is the angle
We know that
Substituting arc length as
Substituting
From the above calculations, we have found the value of the central angle is equal to
Hence, the correct option is (a).
Note: The point where you go wrong in this problem is in writing the value of radius and arc length for the sector because it demands a clear visualization of how the cutting of cone along slant height will give you radius of the sector as slant height and arc length as the circumference of the base.
You can visualize it by imagining the given cone in the form of a birthday cap so when we make a cut along the slant height then the cone shape will roll out into an arc. For instance, let us assume that the below figure is in the birthday cap so when we make a cut alone CG then the folded cone will open into an arc whose radius is CG.

Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




