
A octahedron has _____ faces and 6 vertices with 12 edges. (Use Euler’s formula)
Answer
489k+ views
Hint: In the question, we were given that the number of edges of octahedron and number of vertices of an octahedron. Let us assume the number of faces in octahedron as F, number of vertices as V, number of faces as F, and number of edges as E. Now by Euler’s rule,
Complete step-by-step solution:
Before solving the question, we should have a clear idea about Euler’s rule.
According to Euler’s Rule, “If F is the number of faces of a polyhedron, V be the number of polyhedron and E be the number of edges in a polyhedron, then
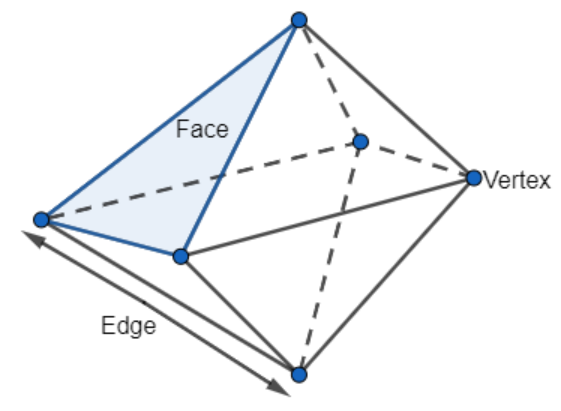
From the question, we were given the number of vertices on the octahedron and the number of edges of the octahedron. With these, we have to find the number of faces of the octahedron.
The number of faces of octahedron can be found by Euler’s rule.
Let us assume the number of faces in octahedron as F, the number of vertices as V, and the number of edges as E.
So,
We have to find the number of faces.
So,
We should rewrite the formula such that we should place the number of faces on L.H.S and remaining terms on R.H.S.
In the question, we were given that number of edges of the octahedron is equal to 12.
So,
In the question, we were also given that the number of vertices on an octahedron is equal to 6.
So,
Now we will substitute equation (2) and equation (3) in equation (1).
So, the number of faces in an octahedron is equal to 8.
Hence, option B is correct.
Note: We should know that Euler’s rule deals with polyhedrons. By using Euler’s rule, we can say whether a polyhedron exists in nature or not. We should know that the value of F, V, and E should not be negative or zero at any cost. So, the value of F, V, and E should be positive. By using Euler’s rule, we can find whether a polyhedron of given values of F, V, and E exists or not.
Complete step-by-step solution:
Before solving the question, we should have a clear idea about Euler’s rule.
According to Euler’s Rule, “If F is the number of faces of a polyhedron, V be the number of polyhedron and E be the number of edges in a polyhedron, then

From the question, we were given the number of vertices on the octahedron and the number of edges of the octahedron. With these, we have to find the number of faces of the octahedron.
The number of faces of octahedron can be found by Euler’s rule.
Let us assume the number of faces in octahedron as F, the number of vertices as V, and the number of edges as E.
So,
We have to find the number of faces.
So,
We should rewrite the formula such that we should place the number of faces on L.H.S and remaining terms on R.H.S.
In the question, we were given that number of edges of the octahedron is equal to 12.
So,
In the question, we were also given that the number of vertices on an octahedron is equal to 6.
So,
Now we will substitute equation (2) and equation (3) in equation (1).
So, the number of faces in an octahedron is equal to 8.
Hence, option B is correct.
Note: We should know that Euler’s rule deals with polyhedrons. By using Euler’s rule, we can say whether a polyhedron exists in nature or not. We should know that the value of F, V, and E should not be negative or zero at any cost. So, the value of F, V, and E should be positive. By using Euler’s rule, we can find whether a polyhedron of given values of F, V, and E exists or not.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

What crosssections do you get when you give a Vertical class 7 maths CBSE

What were the major teachings of Baba Guru Nanak class 7 social science CBSE

What are the controls affecting the climate of Ind class 7 social science CBSE





