
A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold Pens. The dimensions of the cuboid are 15cm by 10cm by 3.5 cm . The radius of each of the depression is 0.5cm and the depth is 1⋅4cm .Find the volume of the wood in the entire stand.
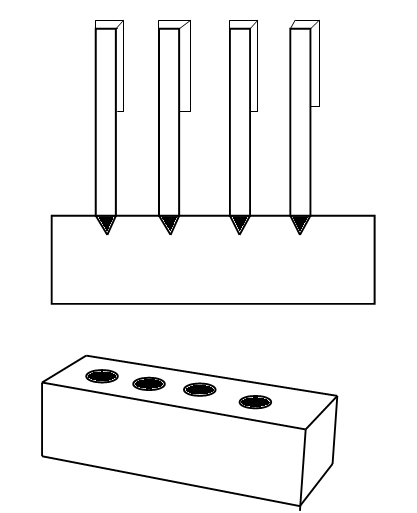

Answer
430.2k+ views
Hint: We find the volume of the stand as
Complete step by step answer:
We know that a cuboid is a three-dimensional object with six rectangular faces joined by 8 vertices. It has three different types of sides called length, breadth and height denoted
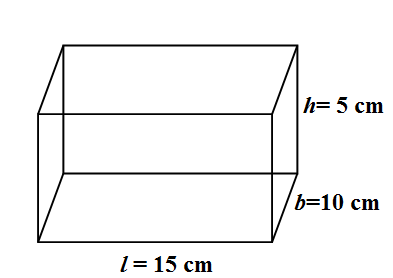
We are given the question that the wooden pen stand is in the shape of a cuboid with The dimensions of the cuboid are 15cm by 10cm by 3.5 cm. So let us assign
So the volume of the cuboid is
We know from the right circular cone that the line segment joining the apex to the center is the height of the cone denoted as
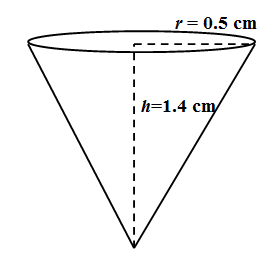
We see that there are 4 conical depressions on the wooden pen stand. We are given that the radius of each of the depressions is 0.5cm and the depth is 1⋅4cm. Here the radius of the depression is the radius of the base that is
So volume of one conical depression is ;
So the volume of the wooden pen stand volume of the cuboid minus the volumes of 4 conical depressions that is
Note:
We have taken the value of
Complete step by step answer:
We know that a cuboid is a three-dimensional object with six rectangular faces joined by 8 vertices. It has three different types of sides called length, breadth and height denoted

We are given the question that the wooden pen stand is in the shape of a cuboid with The dimensions of the cuboid are 15cm by 10cm by 3.5 cm. So let us assign

So the volume of the cuboid is
We know from the right circular cone that the line segment joining the apex to the center is the height of the cone denoted as
We see that there are 4 conical depressions on the wooden pen stand. We are given that the radius of each of the depressions is 0.5cm and the depth is 1⋅4cm. Here the radius of the depression is the radius of the base that is
So volume of one conical depression is ;
So the volume of the wooden pen stand volume of the cuboid minus the volumes of 4 conical depressions that is
Note:
We have taken the value of
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE

The capital of British India was transferred from Calcutta class 10 social science CBSE

What is the full form of POSCO class 10 social science CBSE

Identify the phrase s in the following sentence The class 10 english CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE




